Transient analysis of inductor circuit and back EMF

Question

In a lecture exercise from a circuit course, I encountered the following circuit with only an inductor and a voltage source. The \$v\$ vs \$t\$ and \$i\$ vs \$t\$ graph is as follows:

-The KVL equation for the circuit is \$V-L\ \frac{di}{dt}=0\$.

-Assume \$t<0\$, no energy is stored in the inductor and the switch is open.

-Switch closed at \$t=0\$.

What I have trouble understanding is that from my previous electromagnetic physics course, change in current,\$ \frac{di}{dt}\$, generates a back emf, and the induced current of this back emf opposes the change in current. So from my understanding, the sequence of events when we just close the switch will be as follows:

1. Current has jumped at \$t=0\$ i.e.\$ \frac{di}{dt} = \Delta I > 0\$
2. A back EMF is generated due to the jump in current i.e. \$\varepsilon_{emf}=-L\ \frac{di}{dt}=-L\Delta I<0 \$
3. Current slowed down by the back EMF generated i.e. \$ \ \frac{di}{dt} <0\$
4. The updated back EMF generated is now \$\varepsilon_{emf}=-L\ \frac{di}{dt}>0 \$ because \$ \ \frac{di}{dt} <0\$

However, if the above sequence of events is correct, then it looks like the KVL equation has to be violated because in order to satisfy the KVL equation, \$ \ \frac{di}{dt}\$ has to stay constant for all \$t\$. In addition, the \$i\$ vs \$t\$ graph above only reveals the current that has already been slowed down by the back emf but not the current before the drop. I hope someone can tell me what is wrong with my understanding in circuit with inductor.

Answer 1

the i vs t graph above only reveals the current that has already been slowed down by the back emf but not the current before the drop.

Stop thinking that there has to be some initial current to cause the back-emf. There was no current 'before the drop'. It started at zero exactly as the equation says it must, because that is the definition of inductance (if it acted differently it wouldn't be an inductor!).

If you have difficulty understanding the concept then consider this analogy:-

Newton's Second Law of Motion says that Force = mass x acceleration. But acceleration is change in velocity divided by change in time, so the equation is \$F = m\ \frac{dv}{dt}\$ . Notice how similar this is to \$V=L\ \frac{di}{dt}\$

Now imagine that you have a heavy block of mass \$m\$, resting on a frictionless surface. You then apply a constant force \$F\$, and of course you know what will happen; the block moves - imperceptibly slowly at first, but continuously building up speed as it responds to the constant force pushing on it.

The 'force' is your voltage, the 'mass' is your inductor, and the 'velocity' is your current. The inductor resists the voltage causing the current to increase just like the massive block resisted the force causing its velocity to increase, and the current in the inductor starts at zero just like the velocity of the block started at zero.

So you see the equation works both ways. If the current changes continuously at a certain rate it generates a constant voltage in the inductor. If a constant voltage is applied to the inductor it causes the current to continuously change at a rate determined by the voltage and inductance. It doesn't matter what the 'cause' and 'effect' are, the result is the same - the equation always applies.

Answer 2

You are right, there is no back EMF without current.

BUT: the electrons making the current are the same, whose motion generates the magnetic field that creates back EMF.

Therefore, it is not like you first briefly accelerate, then note the back EMF, decelerate, accelerate again etc. It is a smooth process described by Maxwell's differential equations of moving charges in an electric field. The charges gradually pick up speed (in a classical non-quantum dynamic description anyway).

However, the notion of a pure inductance L is an idealization and doesn't work when taking Maxwell equations literally to the charge level. If there is an extended conductor of any shape, there will be always stray capacitance. Therefore, the electric field front will also move a little bit of charge as it shoots to the other side of the inductor.

Answer 3

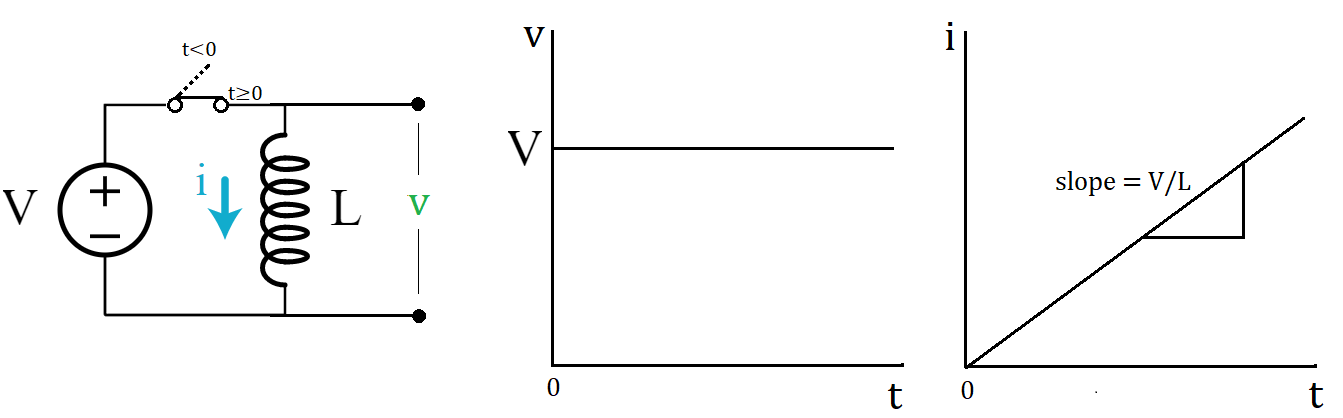
What you have learnt in electromagnetic course is valid of course

i think you are following the right definitions to get the understanding but you are missing important facts

so in order to understand you need to keep in mind these facts :

1-For the back emf to exist it needs a change in the magnetic flux to happen :that change has to happen before the back emf develops

2-The voltage source has always and advance on the back emf what that means is the back emf does not develop instantaneously when the source is connected , the back emf takes a delay to develop(that`s the lag you see in ac inductive circuit) but we tend to forget about it in DC

3-an opposing equal force does not mean necessary a decreasing flow (speed mechanically , or current electrically) in the system itself, it decreases the original flow that would have to happen if the inductor or inertia was not there and depending on what the system is you get a constant rate change (constant acceleration ) if the system is an inductor, or a constant flow (current/speed ) if the system is a dynamic resistor, no flow if the system is a static resistor (a non conductive material is kind of static resistor ,you need a breakdown voltage to get through it and transform it into a dynamic resistor)

before taking an attempt in explaining the current behaviour in your circuit above before t=0 at t=0 and after , recalling some analogies will be useful:

The third law of newton in mechanical systems is a lifesaver and still valid for electrical systems by analogy:

where mass (inertia) is equivalent to inductance L dynamic resistance is equivalent to the Resistor R Static resistance is equivalent to non conductive material which requires a breakdown force to overcome the static force Stiffness K is equivalent to the capacitor C

Let a system subject only to it`s own inertia (mass) with an external force F acting on it ,we get the following equilibrium equation:

F=M*a where a is the acceleration or the rate at which the speed of the system changes over time

This equation shows that for M*a to develop a change in the system creating the "a " has to occur so a change in the initial speed of the system has to occur.

The initial speed is zero, so as long as F is not there ,no change in the speed occurs and a is zero so M*a is zero and no back opposing force is developed Now We introduce F to act on the system M , F has an immediate effect and starts pushing on M ,if the system was massless it will accelerate it with an infinite acceleration and hence it will gain an infinite speed but because M is there it can only push a little amount immediately so that at t=0+dt the Mass would gain a limited speed of 0+dv ,even if we make dt the smallest possible (epsilon), the dv will also be smaller so that the di/dt is always constant and defined by the M value

Now M*a will develop to oppose F but this opposing force takes time to appear (not only the dt inherent to the creation of "a" but a real lag behind F, that's the famous inductor lag which is responsible for example for the slip in the induction motor ), M*a is always chasing F during that time F will push another amount of speed in the system with dv/dt being always the same so V increases as M*a is only generating the first deviation dv for now which is the new steady state for M , but as F pushed a new amount and V increased again ,M again keeps developing an M*a to oppose the new change which is now the new dv pushed by F ,or it is (the new dv plus the old dv)-the old dv = the new dv , and again during that time F pushes a new amount dv in the system etc., and dv/dt stays always constant which means the system increases its speed with a constant rate . If you remove F now , a becomes zero which means the system will keep it`s last speed as a constant and keep moving at this speed unchanged till a new force tries to change it .

If the system had a dynamic resistor , that would only change the maximum original speed vmax that F can Push so the inductor still limits the change but only up to the maximum value allowed by the resistor and then you get constant speed as if you only had the mass with F=0 ( which is true as here R*Vmax completely opposes F)

Now for your example just replace the speed V by the current I and you end up with exactly the same behaviour .

You can also reason using the external original maximum speed trying to push through( as M opposing the maximum speed rather then the pushed amount of speed into the system but always keeping in mind that F has and advance ) instead of the internal system speed you will come to the same conclusion.

Another tool that really is powerful in understanding how the power flows in different systems with different characteristics (stiffness,resistance,inertia) is bond graphs, it always works with flow and force whatever the system is mechanical (force is the mechanical force and flow is current),hydraulic (force is pressure and flow is the hydraulic flow ,electric (force is electric potential and flow is current ),thermal (force is temperature and flow i don't remember what was that ) , unfortunately it has been a while since i have studied it and used it so i don`t remember well the principles to use it but i know for sure it is a powerful tool to understand the exact flow of power/energy in the system , what goes into what and how including transformations, if you can spend some time on understanding it, it will help you understand and solve this kind of problems

Answer 4

For \$t < 0\$ we have \$i(t) = 0\$. The inductor feels no pressure from the voltage source and so no back-emf is needed to keep the current at zero.

At \$t = 0\$ we also have zero inductor current but the inductor is now feeling the pressure from the voltage source. The back-emf in this case exactly cancels out the voltage source emf to make the current zero at that instant.

So, you're right that there is back-emf at \$t = 0\$, but there is also emf from the voltage source.

Answer 5

Inductor opposes any "change" in the current in the circuit. As you know, $$V_L = -E = L\frac{di}{dt}$$.

The more the change in current the greater the opposition. But this does not mean that if, for instance, the current is rising then inductor would start reducing the current. The presence of inductor only affects how fast the change is happening, not the change itself.
So if you have a circuit where current is rising with time, then presence of inductor would only reduce the rate at which the current is rising.
This is exactly what is happening in your circuit. Assuming the ideal case, as you present, if the wire has no inductance, then it is simply a short circuit around a voltage source. Thus at t=0, you get, $$i=\infty => \frac{di}{dt} = \infty$$. And now because of non-zero inductance, the rate of change of current is lower but the current would again rise to infinity after long time.

Answer 6

The problem of power development and energy exchange under equilibrium conditions is treated in thermodynamic theory using the concept of a quasi-static process. In the question as posed the driving voltage source represents a source of electrical power and the inductor represents a sink of electrical power. The transfer of power from the electrical source to the inductor as power sink occurs at constant voltage with rising current where voltage times current is electrical power at an instant of time.

Kirchoff's Voltage Law (KVL) states that the sum of voltage drops around a closed circuit loop equals zero. This law holds when we assume that the ideal inductor takes a back-emf voltage equal to the applied source voltage. This assumption is based on Lenz's law.

Kirchoff's Current Law (KCL) states that the sum of currents flowing into a circuit node equals zero. This law holds when we assume that the current output from the voltage source is equal to the current through the ideal inductor in the circuit as drawn in the question.

The question, as I understand it, is as follows: If the back-emf voltage at time t => 0 always equals the source voltage then why does the inductor current not remain i = 0 but instead rises as a function of time given by:

$$i = \frac{V}{L}t + 0$$

since zero current would flow through the switch if we replace the inductor with a voltage source equal to the potential of the driving source?

The answer from physics escapes me at the moment. However in circuit theory the ideal inductor would sink current when connected across an external voltage source. This inductor as current sink takes the value of the external voltage applied (due to Lenz's law) and increases current draw from the voltage source while storing energy in a magnetic field.

If the inductor is not ideal there is a series resistance causing an exponential rise in the current with a finite limit on the maximum inductor current proportional to the applied voltage. Then the current becomes constant through the inductor at steady state equilibrium where the inductor performs like a constant current sink and the magnetic field stores finite energy and the internal resistance dissipates thermal energy to the surroundings.