The circuit is in a steady state, and the switch is lifted at t=0. In (a), i calculated the current i and the voltage v at the instantaneous moment that the switch is lifted. The current is 2A and the voltage is 12V.
For (b), I need to calculate di/dt at the same instantaneous moment. I can reach that value if I can figure out the back emf (VL) of the conductor. Using Kirchoff's Law on the loop present after the switch is opened, I derive the following equation:
VL - 10(2) - VC = 0
Where VL is the back voltage given off by the inductor, 10(2) is the voltage drop across the two resistors in series, and VC is the voltage across the capacitor. Solving for VL then gives me 32V. It this correct? This seems like an unlikely answer. I believe my signs in my kirchoff equation may be wrong. Can someone check this for me?

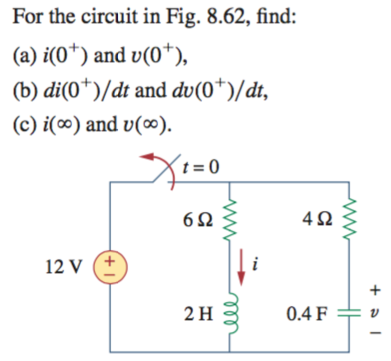
VLthat comes fromVL = L * di/dtwas the back emf generated by the inductor. Thanks for the help. \$\endgroup\$