That's not a transmission line, that's a trace in space.
It would be a transmission line if the trace were over ground plane, and spaced adequately from nearby traces (microstrip geometry).
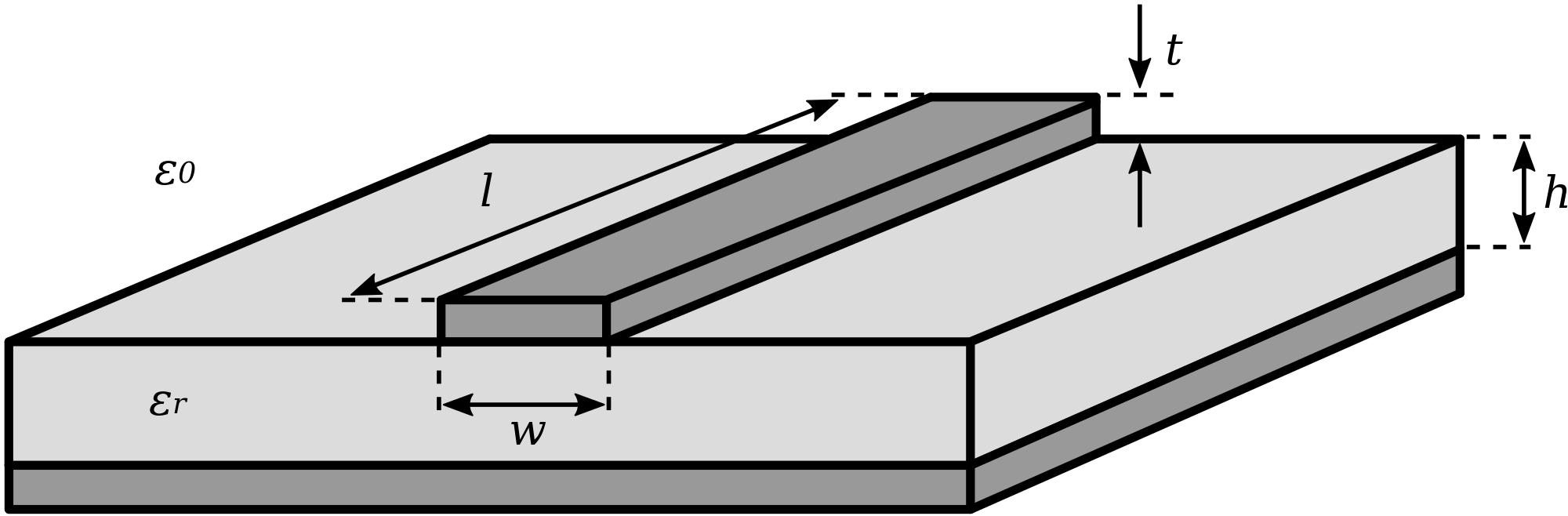
Follow standard calculation methods for square spiral inductors.
To explain in more detail:
A "normal transmission line", would be a well-defined pair of conductors, of constant cross-section, dielectric inbetween, and well-defined impedance and propagation velocity.
For the flat spiral geometry, you can think of (for very short time scales i.e. ≪1ns), one trace, with respect to its neighbor, as an edge-coupled flat (planar) differential pair; which will be a fairly high impedance, 100-200Ω say. But after the wave propagates once around the loop, now what was the signal becomes the ground, and vice versa.
The structure can't be modeled as a simple normal TL.
We further have the problem that, for each pair of traces, it's not against ground at all, but the companion of one pair, is in series with the next, and so on until the end of the winding. The transient impedance is actually a whole bunch of TLs in series (at the "start" port), then looped around in parallel (at the "finish" port, skewed by one index, except for the start and finish which connect to nothing else).
And furthermore, all the TL lengths vary, because the length around the spiral varies. (They're equal when it's e.g. a cylindrical helix, as in a single-layer solenoid coil.)
For more information along those lines, you might want to research the helical waveguide: this is one model of the helical solenoid winding, used to describe its impedance. The overall result is a much higher impedance (Zo in the kohms is easily achieved), and dispersion (propagation velocity varies with frequency). Similar analysis applies to the spiral case, with much stronger dispersion I suppose, and there are other known periodic structures with peculiar frequency response, meta-materials, etc.
Also consider the converse:
A transmission line is a transmission line, specifically because it is not an antenna. That is, EM fields are confined within the structure, rather than propagating out into free space.
Microstrip is a fairly poor example, as TLs go -- one whole side is open to space, and indeed radiation is noticeable from such structures. But it's usually low enough to be an acceptable compromise; for example, a 4-layer PCB with logic-level signals (e.g. LVCMOS at 3.3V and ~ns edge rate, or LVDS-style signals at ~10mA and ~Gbps data rates) can pass commercial emissions limits without much difficulty.
This is in direct contradiction to the purpose of an RFID coil: to couple to free fields.
A transmission line, as a trace over ground plane, is precisely the wrong thing to use here; the ground plane closes the loop local to the trace, greatly reducing the coupling to free fields. Rather, a loop antenna is normally used, with huge open area and no ground plane, specifically so that wireless communication is possible.
As above, we can still make some kinds of transmission-line-based observations -- but they become much more hand-wavey in nature, harder to scope out and model and analyze, and pretty quickly our one-dimensional analysis method (the transmission line) fails and we must submit to full-field 3D analysis.
Conversely, when we don't need the full-field analysis, as say at microwave frequencies -- it suffices to use low-frequency approximations. Hence the above suggestion to follow standard calculation methods. The most common of which I believe is due to Wheeler (sadly, often repeated but rarely cited!). Approximations by the way, that include ignoring various aspects such as wire shape, diameter, spacing, material (conductivity) and frequency; so expect some error (easily >10%) between calculation and the real article. This is worse than the tuning can be for an RFID gadget, so allow a step in the project plan to tune it -- for example, design for a low target value then increase load capacitance until on frequency; or iterate the layout until the self-resonant frequency is correct.
Finally, to answer the title question as curious readers may find it:
Does coiling a transmission line affect its characteristic impedance?
No, or not very much. If you wind up some coax on a spool for example, as long as the radius of curvature is much larger than the outer diameter, nothing much happens. Do observe that the cable is made of a range of materials -- the polyethylene or whatever dielectric is much less stiff and strong compared to the copper (or plated steel) conductors -- and so the cross-section becomes deformed when bent around, more and more as the radius of curvature approaches the outer diameter.
The resulting displacement, for tight bends or kinks, still doesn't amount to much for most signals -- it's a brief interruption where the impedance changes, and such changes are only sensible by waves by their size in relation to the wavelength. A deformation 1cm long is hardly sensible by a wavelength of 1m, etc. Such a kink can be detected by TDR (time domain reflectometry), where the wavefront length is comparable to the defect size.
Similarly, crushed cables can be detected by TDR; such is the basis of some traffic measurement systems for example.
The most important consequence from bending, is probably the reduced breakdown voltage, if the line is operated at high voltage (including at DC), or near the maximum [apparent] power rating. The core conductor being squished towards one side within the dielectric, causes the dielectric to thin, reducing its breakdown voltage. In the extreme, the core conductor can indeed get pulled all the way through the dielectric, shorting out the cable entirely.
Hence, you're advised not to bend such cables tightly. Always read the datasheet and supporting information from the manufacturer!