How to convert the equation to its complex form?

Question

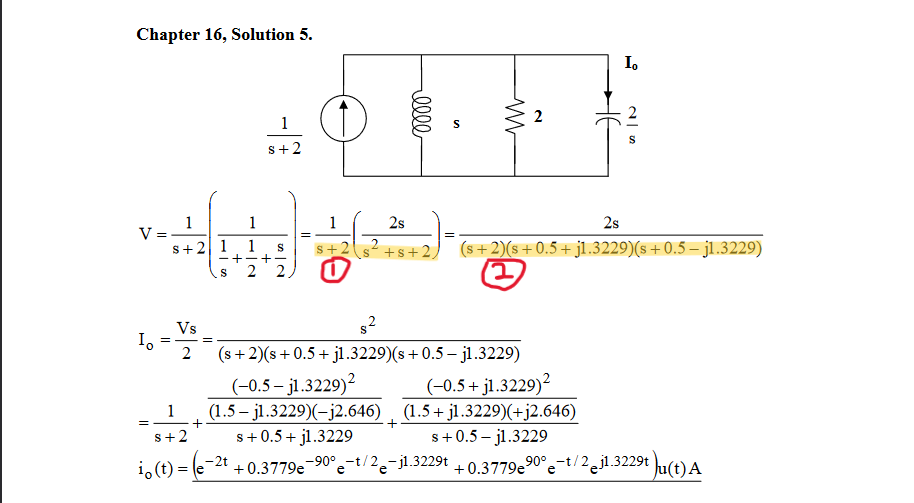
I'm currently trying to calculate the answer for I_o. However, I don't understand how the equation is converted from the highlighted value labelled (1) to the one labelled (2), as shown in the picture attached. How do I obtain the equation in (2) form? Any help is much appreciated.

Answer 1

It's just the quadratic equation: \$\frac{-b\pm\sqrt{b^2-4ac}}{2a}\$. In your case, \$a=1\$, \$b=1\$, and \$c=2\$.
So \$\frac{-1\pm\sqrt{-7}}{2}\$ or \$-\frac12\pm j\frac12\sqrt{7}\$.

So \$s^2+s+2=>\left(s-\left[-\frac12+ j\frac12\sqrt{7}\right]\right)\cdot\left(s-\left[-\frac12- j\frac12\sqrt{7}\right]\right)\$.

In freely available Python/SymPy/SageMath:

list(roots(s**2+s+2,s))
[-1/2 - sqrt(7)*I/2, -1/2 + sqrt(7)*I/2]
expand(prod([s-i for i in list(roots(s**2+s+2,s))]))
s**2 + s + 2

Answer 2

In general, an n-th order polynomial can be factored out into n factors: $$ c_n z^n + c_{n-1}z^{n-1} + \dots + \overbrace{c_1z^1}^{c_1z} + \overbrace{c_0z^0}^{1} = \overbrace{(z-z_n)}^{\text{n-th factor}} \overbrace{(z-z_{n-1})}^{\text{(n-1)-th factor}} \dots \overbrace{(z-z_1)}^{\text{1st factor}}. $$

2nd-order polynomials, called also quadratic formulas, can be converted to a product of 2 factors: $$ as^2+bs+c = \overbrace{(s-s_1)}^{\text{1st factor}} \cdot \overbrace{(s-s_2)}^{\text{2nd factor}} $$ where \$s_1, s_2\$ are zeroes (solutions to) the quadratic equation \$(s-s_1)(s-s_2)=0\$. It's just s instead of x or z as you may see in the literature for quadratic equation solving.

The roots \$s_{1,2}\$ are complex numbers. Don't forget that real numbers are a proper subset of complex numbers. The roots can be equal to each other, and then they are called a double root. That's not the case here.

Here, \$s^2+s+2\$ has zeroes \$s_{1,2}=-\frac{1}{2}\pm j\frac{\sqrt{7}}{2}\$. The zeroes here are a complex conjugate pair. That's the case when the coefficients a,b,c are real.

You can check it:

$$\begin{aligned} (s-s_1)(s-s_2) &= \left( s+\frac{1}{2}+j\frac{\sqrt{7}}{2} \right) \cdot \left( s+\frac{1}{2}-j\frac{\sqrt{7}}{2} \right) \\ &= \dots \text{carry out multiplications and additions} \\ &= s^2+s+2 \end{aligned}$$