I've always considered voltage to be absolute, i.e. something that is there or is not there. However, the more I think about it, it seems to be more like a delta.
For example, let's say we have a supply that lists its pins as (A) -50V and (B) 0V. If we treat pin (A) as "ground", i.e. as if it were 0V, can we treat pin (B) as +50V?
Another example might be that pin (A) is +10V, and pin B is +25V, so the potential difference is +15V. Can we treat this the same way as if it were 0V and +15V?
You are always using/measuring the difference in potential between two points.
There is no absolute zero in voltage (like there is with temperature), although it is common practice to define earth as 0V. This is not absolutely necessary, you can use any potential as reference.
Practically, voltage is a measurement of a difference between two points. You can think of it only this way, and be a very good engineer. Measuring the difference between two points is easy with a voltmeter, as you no doubt know. This thing you are measuring is usually called voltage but is more properly called electrical potential difference.
But, there is a thing that can be measured in volts that is defined at only one point, and that is the electric field potential. To understand it, you must exit the field of engineering, and enter the field of physics (no pun intended).
Say you have an electron (negative charge) and a proton (positive charge). Naturally, these two will attract, and (so far as I understand it; I'm not a physicist!) this is what keeps electrons stuck to their atomic nuclei.
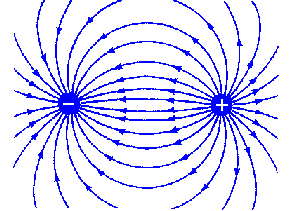
But, if you can pull these two apart, you get a field between them. You might visualize it like this:
These lines represent the force (in our case, the electromotive force) that would be experienced by a charge, were it to be in this field. That is, if you were an infinitesimally small charge in that picture, you would be feel a force pushing you in the direction of the arrows. You can think of the proton as spewing out an invisible fluid, and the electron sucking it in. This invisible fluid acts on other charges like wind.
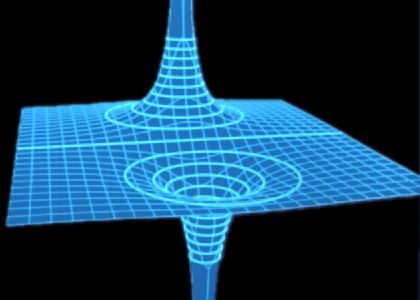
Here's another way to visualize the same field. The proton is a mountain, and the electron is a valley:
If you are a ball on this field, gravity will do work on you, and you will roll downhill. Except, this isn't a gravity field, so our "ball" is made of "charge", not mass. Of course, if you add any charge to this picture, the field changes. This is also true of gravity fields, except the Earth is so much more massive than the ball you imagine that its effect is negligible. So, imagine that your ball of charge rolling around in this field is infinitesimal.
Now one thing you will notice about this field: as we extend it out to infinity, it becomes flat. The electric field potential at this infinitely distant place is \$0V\$, by definition.
If we want to put a ball on the mountain from infinitely far away, we will have to do work. How much? Well, it depends on two things: how high we want to push it, and how big the ball is. A big ball takes more work. Pushing it higher takes more work.
One way to define the volt is joules (energy, work) per coulomb (charge):
$$ V = \frac{J}{C} $$
So you can think of it this way: if you had a ball of charge that was 1 coulomb big, and you did 1 joule of work pushing it uphill, you are one volt high. Or, if you have a 1 coulomb ball of charge, and you let it roll downhill into the electron, and stop it after 1 joule of work has been done, you are at -1 volt. If your ball was 2 coulombs big, then the work is doubled, but it's still just 1 volt.
Thus, you can pick any point in this field, and get its electric potential. It's how much work could be done, or has been done, per unit of charge, getting to there from infinitely far away. With our hill and valley analogy, electric potential is analogous to the elevation.
When you stick your probes on two points, you are asking the question:
If I let a ball of charge that is 1 coulomb big roll between these points, how many joules of work will be done on it?
Of course, we can't get infinitely far away from all charge in the universe, so we can't actually measure electric field potential directly with a multimeter. We can only measure electric potential difference. But, we can calculate the electric field potential, if we know where the charges in a system are.
Since we aren't infinitely far away from all charge in the universe, there is necessarily some electric field potential everywhere. But, we can't do work with just potential; we need a difference. You can't do any work with a ball on a mountain unless you can roll it off.
According to the first three sentences in the Wikipedia entry for Volt:
A single volt is defined as the difference in electric potential across a wire when an electric current of one ampere dissipates one watt of power. It is also equal to the potential difference between two parallel, infinite planes spaced 1 meter apart that create an electric field of 1 newton per coulomb. Additionally, it is the potential difference between two points that will impart one joule of energy per coulomb of charge that passes through it.
3 sentences. 3 times the word "difference" is used.
Spot on. Voltage is a measurement of the electric potential difference between two points.
