In the below circuit, capacitors with several values are utilized (Eg: why 1000μF and 100μF capacitors are chosen). Could you please let me know how to identify the required values of the capacitors and the reason to have a electrolytic capacitor and a normal capacitor in parallel.
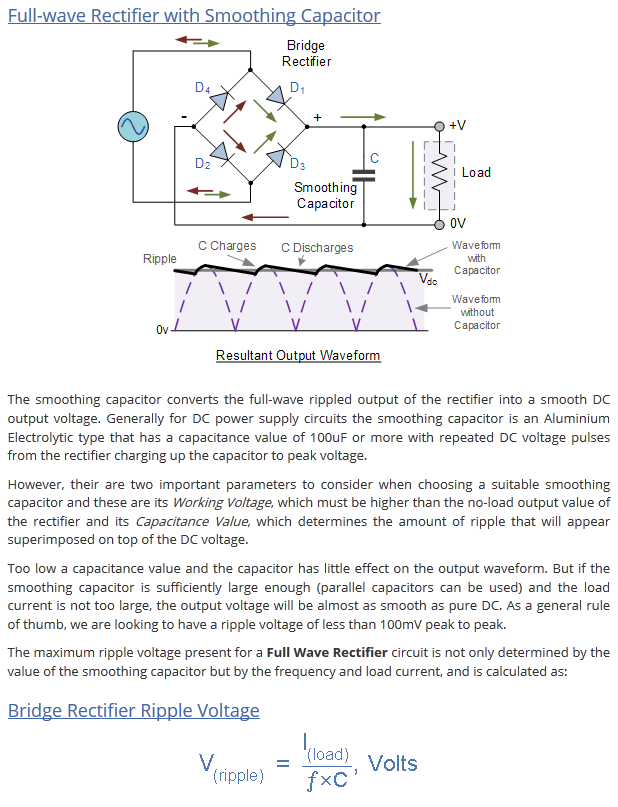
When the bridge rectifier converts AC to DC it does so by ensuring that the most positive voltage from the transformer is routed (via the diodes) to the positive DC feed to the circuit. This charges the 1000uF capacitor up to approximately the positive peak of the AC voltage.
What happens next is that the AC voltage starts falling down from the peak and the 1000uF cap is left charged up. Not until the next peak of the AC comes along (this time on the other AC wire from the transformer) is the capacitor recharged.
The capacitor is charged over a period of a few milli-seconds and, becomes slightly discharged by the regulators and load current. This is why those capacitors are so large - they have to supply a near constant voltage between re-charge pulses delivered through the bridge rectifier. Here is a good article that explains how to calculate the capacitor.
The 1000uF caps are used as bulk energy storage devices and they need to be big because of the duty cycle of charge and discharge. But a big cap like 1000uF will have considerable internal resistance (maybe 1 ohm) and inductance (maybe several tens or hundreds of nano henries). The R and L do not make this capacitor very good for a linear voltage regulator so, a much smaller capacitor (like the 100nF) is applied in parallel - the 100nF might have tens of mill ohms series resistance and inductance of tens of micro henries at the most - together, the 1000uF and 100nF make a good all-round combination.
