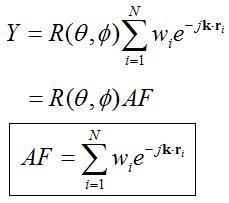In the image, ri means the location of the element in space(x,y,z). and k is alpha + pidcos(thetha)/Lambda and the R(theta,phi) is the radiation pattern of individual element.
In the array factor,
Please explain as to what is the weight wi, Is it the current that we give to the element? Because, the exp part takes care of the phased angle steering. So, what is the role of w, and what are its probable values ? Please elaborate.
This notation is the usual notation for a complex oscillation:
$$f(x) = A*e^{-j2\pi f_0 t}$$
A is the amplitude, the rest is a complex pointer that rotates around zero. If there is no time dependend value, the exponential part introduces a phase shift.
In your case w_i is equivalent to the amplitude and - depending on what unit you want to have - you need to use the appropriate value there. The exponential part replaces the frequency from my example with a spatial location.
If wi is a complex factor it means it consists of amplitude and phase. The equation you showed in your image only takes the phaseshift that occurs by spatial placement off the center axis. Imagine one transmitter antenna and many receiver antennas. The RX antennas are seperated by the distance d which introduces a phaseshift of p. Each antenna has a small mixer+amplifier (which is why the factor wi has been introduced) whose output runs into a summing amplifier. Therefore you can use the multiplier (mathematically expressed by wi) to alter amplitude and phase of the signal. If you choose wi wisely you are able to do some beamforming (eg. by introducing a larger phaseshift to wm than to w1 in the picture)
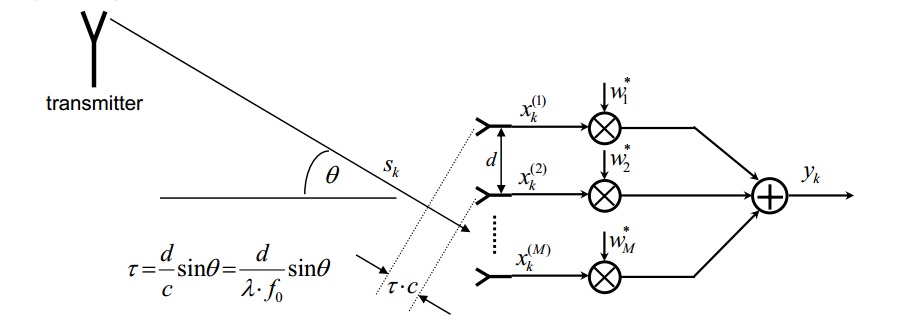
I - and this is only my gut feeling - would use the power fed to the antenna. If all antenna elements are the same you can pull that factor out of the sum:
$$ \sum_{i=1}^n{(a_i*k)} = k*\sum_{i=1}^n{a_i}$$
