How Do Batteries Behave In Series-Parallel (Verify)

Question

Hi I am confused about How batteries behave in series and parallel. I read online and saw that as you stack more batteries in series, the voltage adds together and the mA's stay the same, and if you stack them in parallel, the mA's add together, and the voltages stay the same. But I have found nothing about series-parallel.

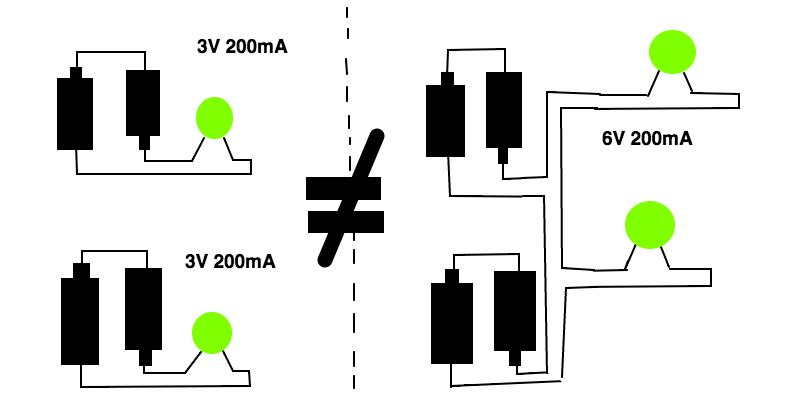
I am have assumed that the following two diagrams would be true based on how loads behave in series-parallel, but I am here to verify that the batteries will actually behave like this in practice, and that there are no hidden quirks in this setup.

1st Set: Parallel - - - 2nd Set: Series

For The sake of this Illustration Lets Say: -------Battery:1.5V 100mA -------Lamp:3V 200mA

This Question is actually for a project that I am doing Which You can see Right >>>Here!<<<

Answer 1

If you add batteries in series their voltage will add. If you add batteries in parallel their capacity (given in mAh most of the time) will add and the voltage will stay the same.

If you do a series and a parallel connection, the rules still stay the same, you could imagine to replace the parallel connection as a single battery with doubled capacity and then have just a series connection of two batteries again.

Note that this does not concern the current of the battery, which is still determined by Ohm's law.

So given that, both of your circuits are sort of equivalent. Just that the lamps in series will be both dark if one fails.

Batteries are capable of handling a certain current, limited by internal resistance and chemistry. This is also increased if you put them in parallel. The current capability is often given as a C-Rate which connects it to the capacity of the cell. 1C means it can handle a current equivalent to the capacity for 1 hour. So a 300mAh cell with 1C can handle a current of 300mA. Now if you put two of those in parallel, the C-rate stays the same 1C, but you increased the capacity to 600mAh, and now you can safely draw 600mA.

Going above the C-rate will reduce the energy you get out of the battery. So instead of 300mAh the capacity might be reduced to 200mAh if you draw a current of 500mA out of a single cell (there is no formula to this, it's specific for every cell). For rechargeable batteries, increasing the current draw and charging current reduces the lifetime of the batteries.

In series connection you have to be weary of the single cells drifting apart over time, which can lead to failures. This is especially a concern with lithium chemistries and rechargeable systems. As every cell is different (has a different capacity) some cells will be empty sooner than others and will be further discharged, below a critical point where the battery gets damaged. Same is true for charging, the cells with lower capacity will be charged to full faster, and after that will be overcharged. Depending on the chemistry different things can happen, lithium tends to be very dangerous in these scenarios, other chemistries are more forgiving and convert further charging current into heat.

A good online resource for all these things is the Battery University which I'd recommend to read first.

Answer 2

I don't know what you mean by equal and not equal, but if the criterion for equality is power dissipation, then all four circuits are equivalent.

Just for grins and for the sake of the discussion, let's assume that the 100mA spec on the batteries is really mAh, that the batteries are fresh, and that:

For the lamp resistance we have:

$$ R = \frac{E}{I} = \frac{3V}{0.2A} = 15\Omega, $$

and for the power dissipation of a single circuit on the left of your graphic:

$$ P = I\ E = 0.2A \times 3V = 0.6 \ watts. $$

A pair of circuits on the left will, therefore, dissipate \$ 1.2 \$ watts.

At the top right we have two 3 volt batteries in parallel, for a total of 3 volts across them, and two 15 ohm resistors in parallel for a total resistance of:

$$Rt = \frac{15\Omega \times 15\Omega}{15\Omega + 15\Omega} = 7.5\Omega $$

That 7.5 ohms connected across 3 wolts will dissipate:

$$P = \frac {E_{}^2}{R} = \frac {3V_{}^2}{7.5\Omega} = 1.2\ watts. $$

At the lower right we have two 3 volt batteries connected in series for a total of 6 volts, and two 15 ohm resistors connected in series for a total of 30 ohms.

Then, since the batteries and resistors are all connected in series, that circuit will dissipate:

$$P = \frac {6V_{}^2}{30\Omega} = 1.2\ watts. $$

Consequently, from the point of view of power dissipation, the pairs of lamps on the left and the circuits on the right are all equivalent.

Answer 3

two 1,5V cells in series is 3V battery, the rules for combining batteries parallel still apply to the 3V battery.

eual and and not equal in the diagram is not accurate, the circuits are different in both cases