This question is in reference to my earlier question:
Resolution gain in Delta-Sigma Converters
What I understand
From the answers I inferred that we need the input to have some amount of noise (to randomize the signal), so that averaging can improve the resolution of the signal. This noise dithering is done by the DS loop in DS ADC. If we use second order ADC instead of first order we are adding more noise so we increase the resolution better than the first order.
But I also read that statistically the uncertainty improvement that can be done by averaging, if the data is completely random is sigma/sqrt(n), where sigma is the standard deviation of the individual measurement and n is the number of samples we are averaging.
So averaging four samples, for instance, would give me an extra 1 bit accuracy as the uncertainty would be halved.
Question
Is the above understanding correct? By increasing the order are we trying to reach this maximum uncertainty improvement limit by trying to randomize the data better, thus, for instance, 1 extra bit if we are using averaging of 4 samples? In other words, is 1 bit increment in the resolution the maximum that can be achieved by averaging 4 samples for any kind of ADC be it normal Averaging ADC or DS ADC?
\$\begingroup\$
\$\endgroup\$
4
-
\$\begingroup\$ Because the way that a Sigma Delta ADC works the standard of 4 samples to get an extra bit is much better. Reread what Dave Tweed said in the previous question. This is due to noise shaping. \$\endgroup\$– Andy akaCommented Sep 1, 2015 at 7:36
-
\$\begingroup\$ Are you saying that we will get an accuracy better than 1 bit? Because I think this is not possible statistically as I explained in this question. \$\endgroup\$– sarthakCommented Sep 1, 2015 at 7:48
-
\$\begingroup\$ Re-read what Dave Tweed said - also note that you need to understand the difference between accuracy and resolution. This was also covered by Dave Tweed. Read his answer and digest. You are going over the same ground here. \$\endgroup\$– Andy akaCommented Sep 1, 2015 at 7:50
-
\$\begingroup\$ Sorry I meant resolution in the above comment. In Dave Tweed's answer he said that longer we average the better the resolution. My question is can we do better than 1 bit if we are averaging 4 samples in Delta Sigma case? \$\endgroup\$– sarthakCommented Sep 1, 2015 at 7:55
Add a comment
|
1 Answer
\$\begingroup\$
\$\endgroup\$
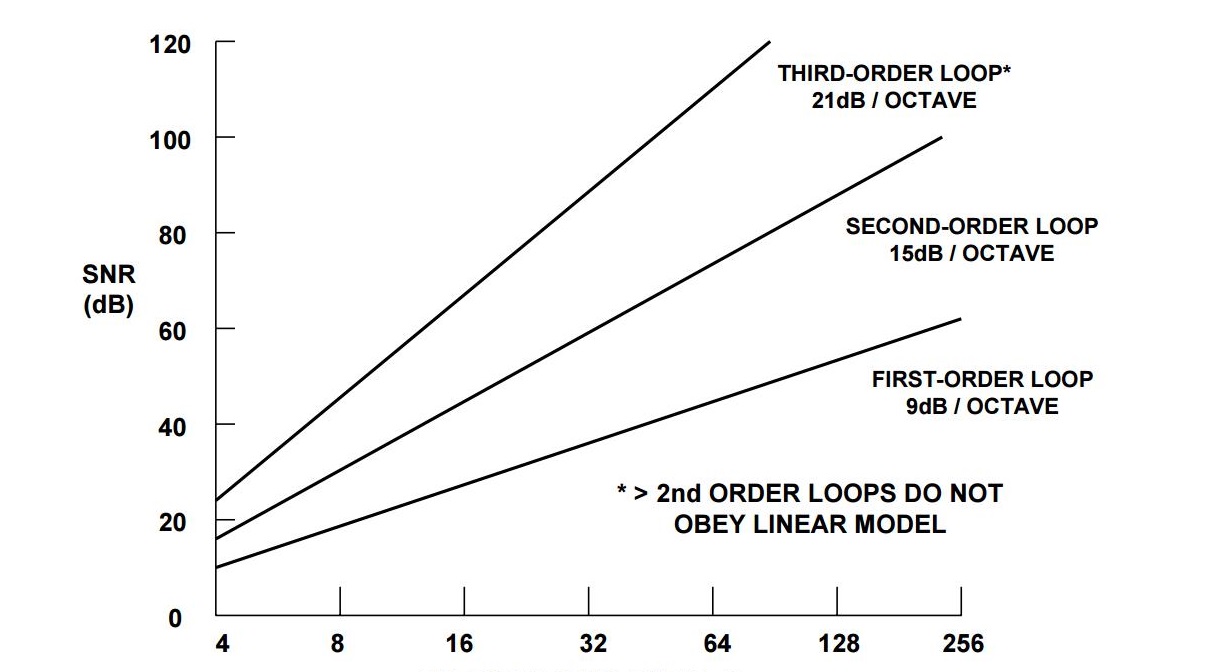
The number of effective bits calculated as follows ENOB = (SNR - 1.76) / 6.02 (this is my reference). So in the delta sigma converters where are able to change to SNR by means of noise shaping, filtering and decimation. The plot below taken from Analog devices app note MT-022 show SNR as a function of the number of samples of 1st, 2nd and 3rd, order sigma delta loops.