0
\$\begingroup\$

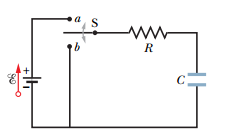
enter image description here

Made this question in physics stack, but maybe I'll get a better answer here.

If we apply loop rule while charging the circuit we get:

$$\varepsilon -iR-\frac{q}{C}=0$$

The way I understand, we follow through the circuit just before the battery, as we move through the circuit there is a increase in potential so \$+\varepsilon\$. Then we go through the resistor, since we are moving in the direction of the current, \$-iR\$. In the capacitor, there is a drop in potential, so \$-\frac{q}{C}\$.

The problem starts for me in the part of discharging the capacitor. Now the battery acts like part of a wire, \$\varepsilon=0\$. The top plate of the capacitor is at a higher potential. Current will flow from the top plate to the circuit in the direction of the resistor (opposite of what it was). Now loop rule would stand for (starting from just before the down plate):

$$\frac{q}{C}-iR=0$$

Because capacitor's top plate is at a higher potential, there is a increase in voltage. Loop rule is applied in the same direction of the current, so \$-iR\$.

But the equation resulted in the loop rule application when discharging a capacitor is given by:

$$\frac{q}{C}+iR=0$$

Why? What is the mistake in my reasoning? Please, help me understand. Excuse my bad english. Happy 2016.

\$\endgroup\$

1 Answer 1

Reset to default
2
\$\begingroup\$

Why? What is the mistake in my reasoning?

Your mistake is that you defined \$i\$ differently in the two situations. When charging, you defined \$i\$ to be positive when the current flows in the clockwise direction.

When discharging, you defined \$i\$ to be positive when current flows in the counter-clockwise direction.

Whoever came up with the alternate equation for the discharging case didn't do that. Instead they stuck with the same definition with positive \$i\$ in the clockwise direction.

To avoid confusion in the future it would be better to either use a consistent definition for your variables, or give them separate symbols (like \$i_c\$ and \$i_d\$ for the charging and discharging case) when you change the definition.

\$\endgroup\$
1
  • \$\begingroup\$ I read a suggestion that could be good to specify before doing the problem the higher potential of the resistor and the lower. In our case, the higher potential is the left (right?). So then, when analizing the discharging of the capacitor I should get (going clockwise in the loop rule): $-i_dR$. Since $i_d=-i_c$ the term goes positive. Is that right? It may seem i'm over complicating but I think the problem is that I've learned to apply kvl only when current don't change direction, so I calculate as either a drop or a increase in potential according to the direction of current... Thanks. \$\endgroup\$
    – João Pedro
    Commented Jan 2, 2016 at 3:24

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.