Control system stability with respect to gain margin and phase margin

Question

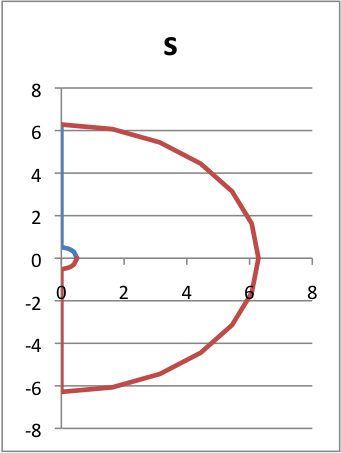
I have learn from control system that for a stable system gain margin and phase margin both must be positive. That is the truth.

But I have a doubt about this simple third order type 2 open loop transfer function whose phase margin is positive but gain margin is NEGATIVE. But both Nyquist stability criterion and Routh Hurwitz's criterion are showing that the close loop system will be stable.

How is it possible? Am I doing something wrong?

The system is as follows :

\$\dfrac{K*(s+3)*(s+2)}{(s^2)*(s+1)}\$ and value of K is "1".

I will be very thankful if someone clears my doubt. I have checked the system using MATLAB also.

Answer 1

For a minimum-phase system, the gain and phase margins must be positive for the system to be stable.

This system has two poles at the origin, and hence is not minimum-phase. Thus it can be stable even if the gain or phase margins are negative.

Answer 2

As Vladimir Cravero states in his comment, the Nyquist stability criterion is the one to be trusted. With an s-contour like this one, there are no encirclements of the -1 point and hence the system is stable. (The "cut-out" at 0 avoids crossing the two poles there.)

Your system only has 90 degrees of phase lag at high frequencies, while the gain margin is appropriate for a system with more, where the phase curve eventually exceeds 180 degrees of lag at high frequencies, and the gain margin, along with the phase margin, indicates how close the loop gain comes to the -1 point as it passes through that area.

There is actually a term to describe systems like this one with more than 180 degrees of phase lag at low frequencies: "conditionally stable", meaning that, while stable, if the gain K becomes low enough, the system becomes unstable. Conditionally unstable systems are to be avoided where possible, because you never know what may reduce the system gain and get you into trouble.