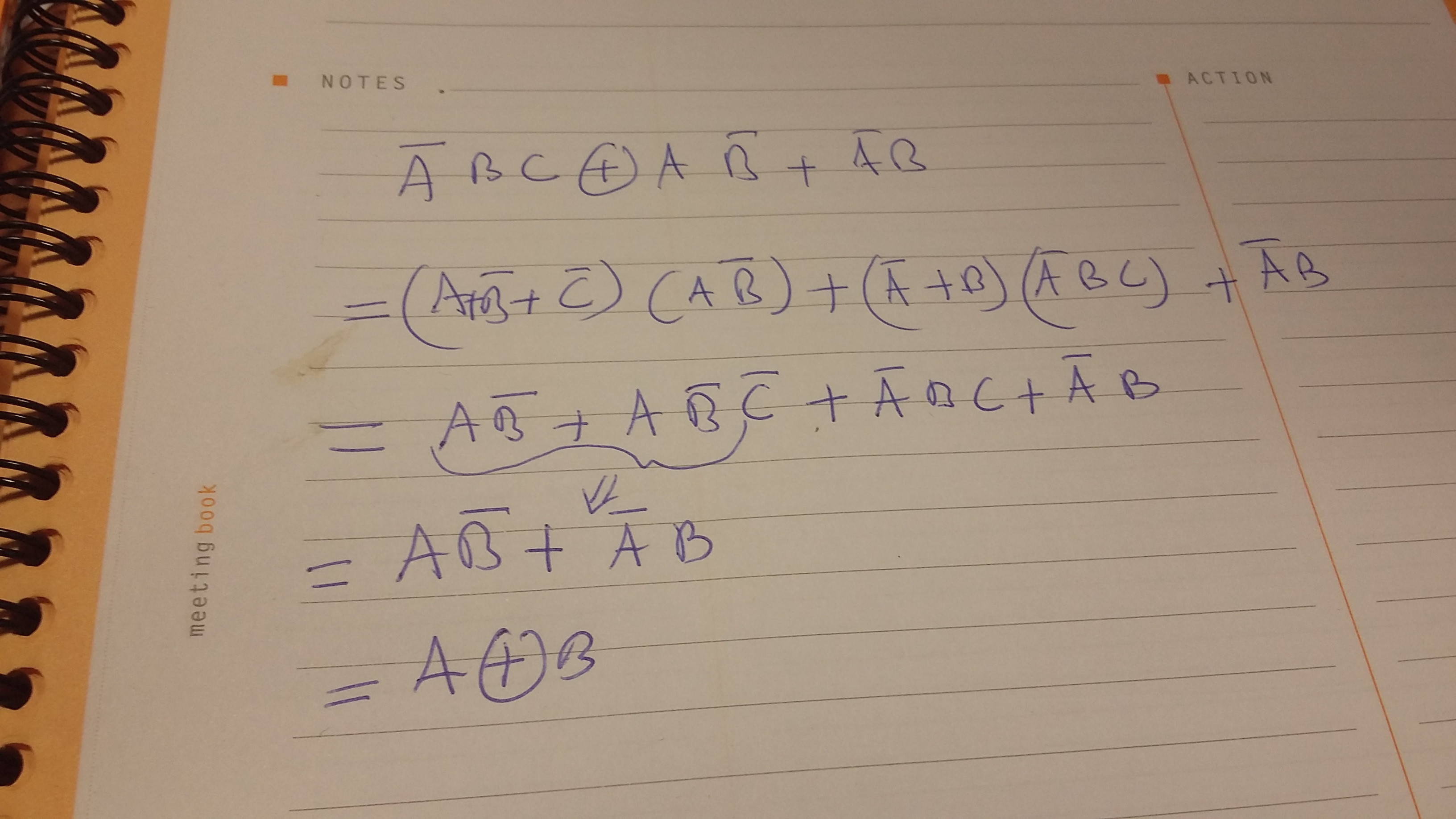Evaluate \$(\overline ABC\oplus A\overline B) + (\overline AB)\$

Question

I'm struggling with evaluating this one:

\$(\overline ABC\oplus A\overline B) + (\overline AB)\$

I've got to

\$A\oplus B + A\overline B \overline C +\overline ABC\$

But how do I prove that \$A\overline B \overline C +\overline ABC = 0\$

Edit: The answer is \$A\oplus B\$

Answer 1

A⊕B is AB(Bar)+A(bar)B

So the Expanded equation is

AB(Bar)+A(bar)B+AB(bar)C(bar)+A(bar)BC

Taking Common factors out

A(bar)B(1+C(bar))+AB(bar)(1+C)

1+ anything is always 1

So we Have A(bar)B+AB(bar) which is A(exor)B

Answer 2

Here is the answer:

Hope this help