I want to create a step-down transformer from 230V to 115V. This will give us 2:1 turns ratio. So this means 2 turns in primary for every 1 turn in secondary should work. But if the 230 Vac supply given to 2 turns of copper wire, it will burn the wire. So at minimum, how many turns in primary side are needed not to burn the wire. Please explain with maths.
\$\begingroup\$
\$\endgroup\$
2
-
\$\begingroup\$ The wire thickness on each side of the transformer must be enough to withstand the expected voltage and current. It is possible that the primary will need a thinner copper wire with better insulation, and the secondary will need a thicker copper wire with thinner insulation. This calculator may help. hyperphysics.phy-astr.gsu.edu/hbase/magnetic/tracir2.html \$\endgroup\$– jbarlowCommented Jul 20, 2016 at 7:30
-
\$\begingroup\$ You need to wind for Urms=4.44fNAB where f in your frequency in Hertz, N is number of turns, A is the cross section area of the core in m^2 and B is the flux density in Tesla. Aim for 0.8 Tesla. \$\endgroup\$– winnyCommented Jul 20, 2016 at 7:45
Add a comment
|
1 Answer
\$\begingroup\$
\$\endgroup\$
Here's a sort of worked example.
2 primary turns will have a very low primary inductance. You could make a general case that a single turn has about 10 uH for an "average" sized silicon-steel laminated transformer. Inductance is proportional to turns squared so that means a primary inductance of 40 uH for 2 turns.
Clearly this will see a melt-down if 230 V RMS at 50 Hz is applied because the impedance is only 0.013 ohms.
So, maybe go to one-hundred turns. Now, the inductance is 0.1 henries and the reactance will be about 31 ohms. The magnetization current will be 230/31 = 7.3 amps.
Maybe the copper could cope with this but what about core saturation?
100 turns and 7.3 amps is a magneto motive force of 730 amp-turns. To get towards a figure for peak flux density, core dimensions need to be known. The important dimension is mean magnetic field length and, for a middling, general purpose power transformer that length might be 100mm.
Now we can calculate the H field. H = MMF/length = 730/0.1 = 7300 At/m.
But we need the permeability of silicon steel and this somewhere in the region of 5000 (relative to air) or 0.0063 in actual terms.
Therefore flux density will be 7300 x 0.0063 = 46 teslas RMS
Peak flux density is root 2 higher at 65 teslas.
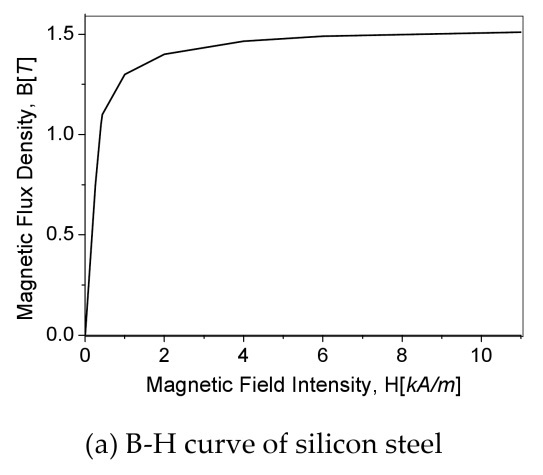
Silicon steel starts to saturate at about 1 tesla so absolutely clearly having only 100 turns on the primary is still a recipe for melt-down: -
So, if you had 1000 turns, inductance would be 100 times greater and current would be 100 times smaller so MMF is modified to 730 x 10/100. The x10 bit is because the number of turns has increased from 100 to 1000 and the /100 is because the current has fallen by 1000 due to the inductance rising by 100 due to ten times more turns.
So, new MMF is 73 and therefore H = 730. B (RMS) is now much smaller at 4.6 teslas RMS. Saturation is still going to be problematic so more turns are needed in this example.
Can you see that increasing turns does have a useful effect of reducing flux density.
Please explain with maths
I'll do it my way.
