0
\$\begingroup\$

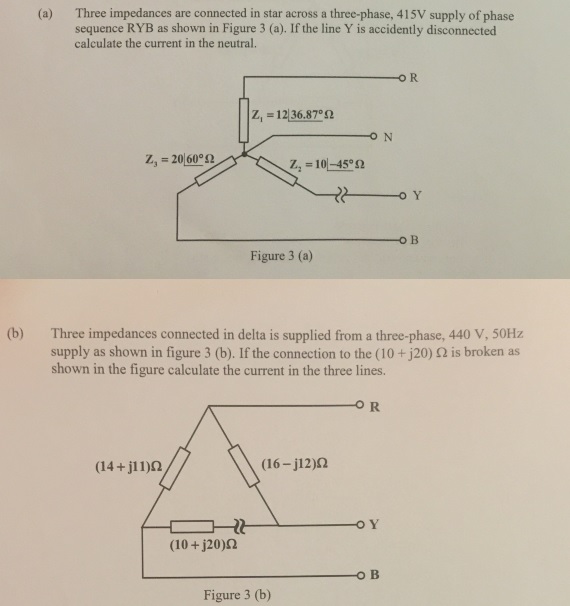
I am sorry if I am asking trivial question. I tried to search previous questions but unable to find it, there is only question on what will happen if neutral line disconnected.

My question this time is about what will happen when one of the three phase loads get disconnected just like the in the figure below?

3 Phase Loads Sudden Disconnection

For (a) which is star-connection, is the phase voltage is still Vline/sqrt(3)? Is Ineutral= -(IR+IB) still valid if IY=0?

For (b) which is delta-connection, is the phase current of still Iline/sqrt(3)?

How do I solve these two questions? I tried to find similar questions online but cannot find any with keywords like '3 phase system, one phase disconnected'.

My answers for part (a) and (b) are as shown below.enter image description here

If you know similar problems from some other source, would you kindly share with me? I can read there myself. Thank you.

\$\endgroup\$

1 Answer 1

Reset to default
1
\$\begingroup\$

Your question title is this: -

What happens when one of the 3 Phase load is disconnected suddenly?

And the pictures in your question (ignoring the line break symbols) are for three phase systems with an asymmetrical load. Your specific question is this: -

what will happen when one of the three phase loads get disconnected just like the in the figure below?

So, for me it's reasonable to assume that you know how to calculate the neutral current with the imbalanced load intact. If you do and it seems reasonable that you do then, for part (a), calculate the neutral current with Z2 set to infinity.

For part (b) it's a similar story just derive the formulas for the three currents and raise the YB impedance to infinity.

If in fact you cannot calculate the currents before the lines are broken then you are asking the wrong question and you should walk before running.

For (a) which is star-connection, is the phase voltage is still Vline/sqrt(3)? Is Ineutral= -(IR+IB) still valid if IY=0?

In the absense of any other knowledge about the 3 phase supply it has to be assumed that it is perfect and therefore the \$\sqrt3\$ relationship holds true always. Neutral current is (under general circumstances) IR+IB+IY but you have to take into account phase angles and therefore some current cancellation.

For (b) which is delta-connection, is the phase current of still Iline/sqrt(3)?

For an asymmetrical load no, the relationship does not hold-up. That relationship is only valid when the load is balanced.

\$\endgroup\$
13
  • \$\begingroup\$ Hellooooooo! Thanks for your answers and explanation. for (a), which is star connection, Yes, I know how to calculate the neutral line current for unbalanced load, which is just IR+IY+IB. If I just set Z2 to infinity, that means simply IY=0, and if the sqrt(3) is still true, that means there is no change to my phase voltage of star-connection, still 240V (415/sqrt(3)), and hence no increase in my phase currents of phase R and B even though Y is disconnected? Because it seems not so logical to me. \$\endgroup\$
    – Prietess
    Commented Jun 24, 2017 at 16:02
  • \$\begingroup\$ Correct but it's different for part b of course. \$\endgroup\$
    – Andy aka
    Commented Jun 24, 2017 at 16:04
  • \$\begingroup\$ Wait, Andy, for part(a), when phase Y is normal, not disconnected, my phase voltage VRN is 240V (angle 0) (if I set it as reference), VYN is 240V (Angle -120), and VBN is 240V(Angle 120). Now, when Y is disconnected, are you saying that they all do not change? If yes, hence no change in phase current also, and hence IN=IR+IB, just 1 term less than the case when Y is not disconnected. But it seems a bit unrealistic to me,.... (I might think too much). Sorry, I am unexperienced on this. Thanks again! \$\endgroup\$
    – Prietess
    Commented Jun 24, 2017 at 16:07
  • \$\begingroup\$ Sorry Andy, because I was reading this source here and I expected that my phase current (which is same with line current for star) will increase when one of the line is disconnected. marineinsight.com/marine-electrical/… It would be good if you could give a simple enlightenment to me on why they are different....? \$\endgroup\$
    – Prietess
    Commented Jun 24, 2017 at 16:10
  • \$\begingroup\$ Now I'm confused as to what you ask. \$\endgroup\$
    – Andy aka
    Commented Jun 24, 2017 at 16:13

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.