Circuit analysis - laplace transform

Question

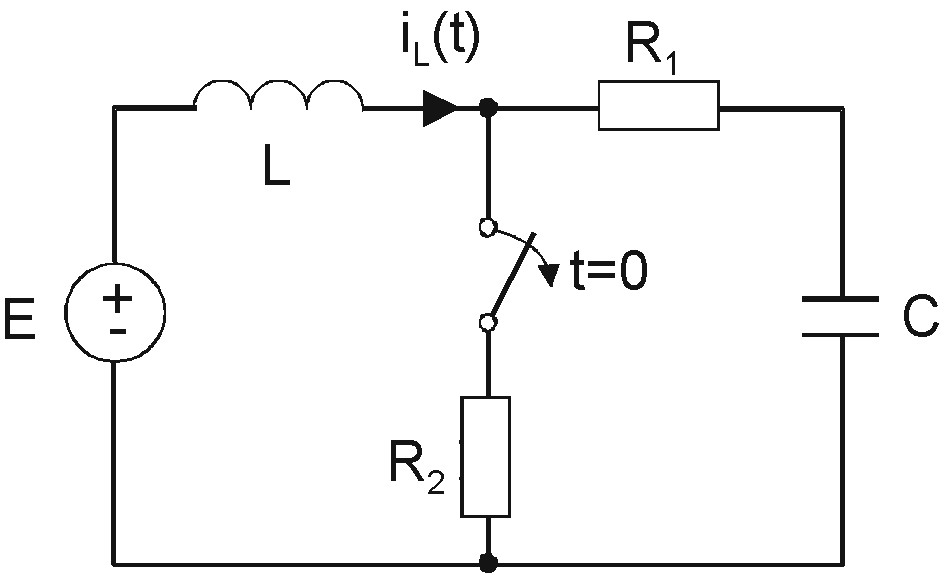
So i have a circuit where R1 = 5 W, R2 = 2 Ω, L = 1 H, C = 1/6 F ja E = 2 V. And i need to figure out what is i_L when t=0.5s with laplace transform.
Switch opens when t=0
When t<0 i got i_L(0)=1A and U_c(0)=0 for initial values.
When t>0 circuit will look like

And now i got for KVL i got

$$ E-U_L-U_R-U_C=0 $$ $$ E-Li_L'-\frac{i_L}{P}-\int\frac{i_L}{C}=0 $$ And now in need to do laplace transform. $$ E-L(sI_L-i_L(0))-\frac{I_L}{P}- \frac{1}{C}*(\frac{1}{s}*I_L)=0$$ But now i got stuck here. Thanks in advance.

Answer 1

Assuming R₁=5Ω ,

Initially, \$V_C\ + V_{R_1} = V_{R_2}\$. Since no current flows through C for \$t(0^-)\$ , \$i_{R_2}=1A\$. So, \$V_{R_2}=V_C=2V\$.

For \$t>0\$,

$$E=L\frac{di}{dt} + i\cdot R_1 + \frac{1}{C}\int i dt + V_C(0)$$

Taking Laplace Transform,

$$\frac{E}{s} = L(sI(s)-I_L(0)) + I(s) \cdot R_1 + \frac{1}{Cs}I(s) + \frac{V_C(0)}{s}$$

Here \$I_L(0) = 1A, V_C(0) = 2V\$

Rearranging,

$$\frac{E+LsI_L(0)-V_C(0)}{Ls^2 + s R_1 + \frac{1}{C} } = I(s)$$

Substitute the values and resolve into partial fractions and then take Inverse Transform to get the value of the current.

Edit:

The Laplace Inverse of 1/7 is 1/7δ(t), which is a function that takes the value 0 for all \$t\neq 0\$.