Calculating Rout of a bjt while using correct RL

Question

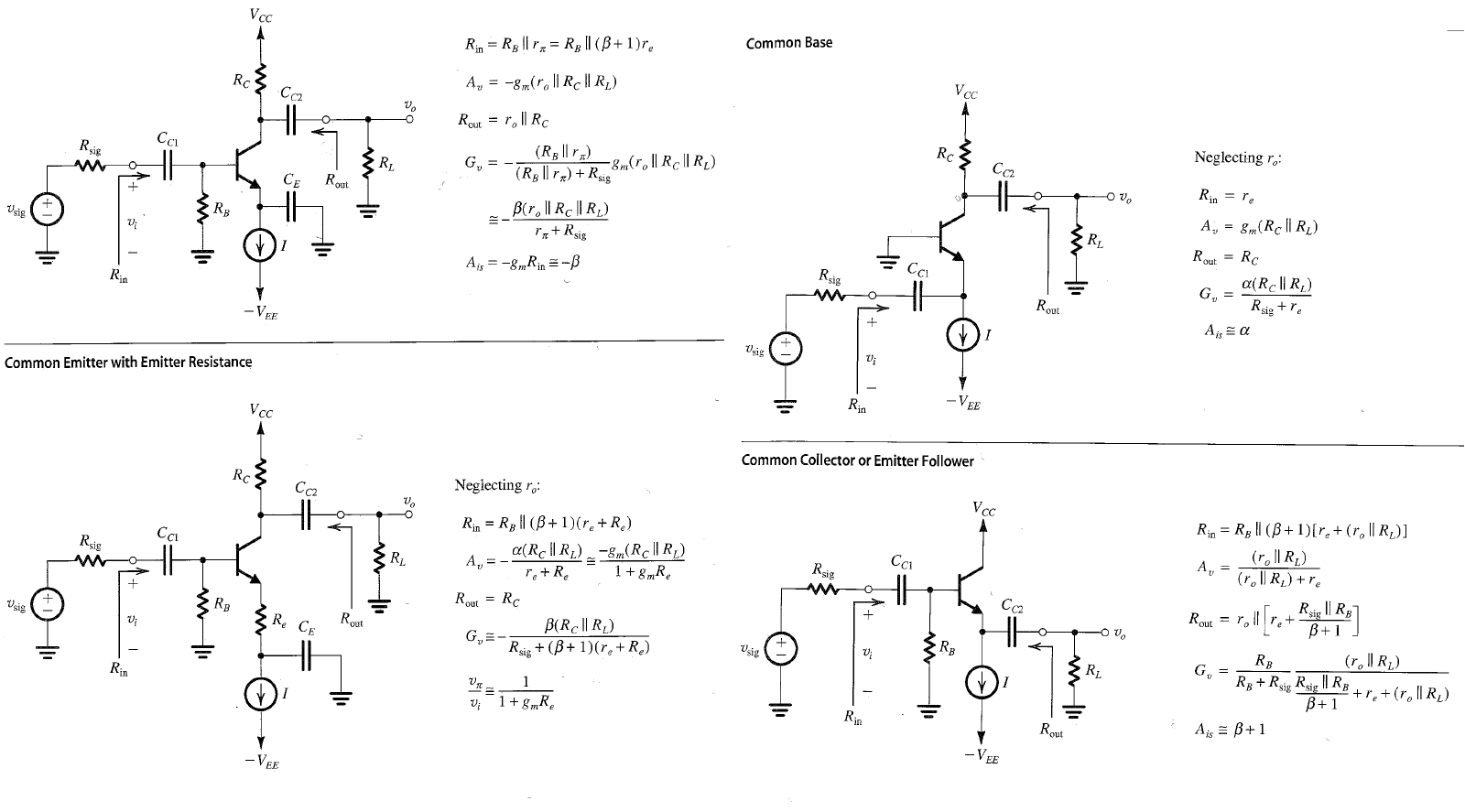
I'm studying the notes of my professor of electronics, and I'm noticing an inconsistency of \$R_{L}\:\$ compared to what I've seen around in books and on the web. Here's what is considered \$R_{L}\:\$ in those notes:

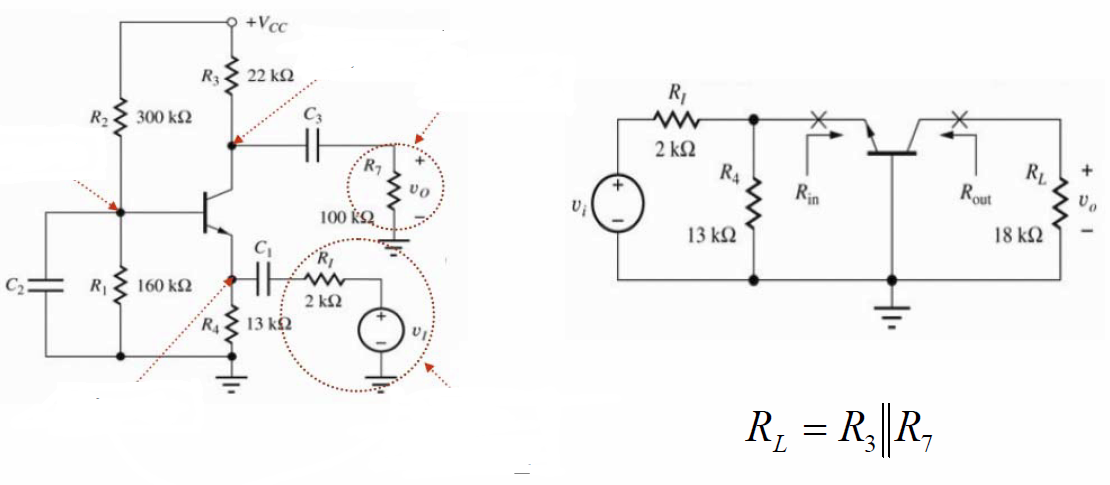
As you see, it's taking \$R_{L}\$ to be \$R_{3}\$ and \$R_{7}\$ in parallel. In my book, \$R_{L}\$ is meant to be what in this image specifically is \$R_{7}\$

My main issue rises when I try to calculate \$R_{out}\$, which is supposed to be calculated with

\$u_{in}=0\:\$, and

\$R_{L}=\infty\:\$, meaning \$R_{L}\$ is to be removed from the circuit to calculate \$R_{out}\$

Coming to this, I'm confused as to which \$R_{L}\$ I'm supposed to be using, or which one is the correct one to begin with.

For example, in the professor's notes \$R_{out}=r_{o}(1+g_{m}(r_{π}//R_{th}))\$, while in my book, a common base bjt has \$R_{out}=R_{C}=R_{3}\$.

Topologies table:

Which one of the two ways is the correct one?

Answer 1

The load is clearly just R7. The parallel combination of R3 and R7, is what I would call Rtc, the AC Thevenin equivalent resistance seen looking out of the collector which, in this case, is 18k. I think it was a poor choice to label that equivalent resistance R_L.

You calculate Rout to the left of R7, i.e., do not ever, ever include the load in the output resistance calculation.

Finally, the small signal resistance looking into the collector is in parallel with R3 so, unless you're ignoring the Early effect, i.e., set r_o to infinity, the AC output resistance is not just R3.

I highly recommend consulting the late Professor Leach's ECE3050 notes:

http://users.ece.gatech.edu/mleach/ece3050/

UPDATE:

I should, I think, provide a justification for my answer above so here it is. One of the parameters of a voltage amplifier is the open circuit voltage gain which is defined as the voltage gain when the load is an open circuit.

Now, when the load is just R7, the open circuit voltage gain is easy to compute and to measure in practice. Just remove R7. The circuit still functions as a voltage amplifier and the gain can be calculated or measured.

But, if R3 is part of the load, to open circuit the load, R3 must be removed! In theory and in practice, the circuit stops functioning altogether as an amplifier.

Answer 2

The problem is that your professor is using language and terminology that is inconsistent with the reference he has provided you (your textbook). I would agree with your survey of books and internet resources in that your professor is doing something weird, but it is weird to me because I have never seen that use of terminology. R_L means something very specific to me, but if I tell you that your professor is using language inconsistent with my education, that isn't going to help you very much.

The other posters have done excellent jobs articulating and demonstrating how they believe R_L should work (and I completely agree with their posts, and you might even find the majority of people would agree with them). However, I don't think this actually addresses your particular situation, and I think this actually requires some specific actions on your behalf and some interaction with your professor. If I tell you what I think is correct, then I have deprived you of an opportunity to learn, potentially hamstrung you on any coursework or exams on the subject, and ruined a chance at correcting your professor (but don't expect that to happen).

Here's what I think you should do (and perhaps keep in mind that this is based on experience with professors in the USA):

1. Visit your professor/TA during office hours, or make an appointment to go over course material.

2. Tell him/her that you are confused by conflicting information from lecture/notes and the book on common base amplifiers. Consider asking the following questions, or using them as guides.

   • What does R_L represent, and how is this different from the load resistance?
   • What distinction is there between the bias resistor R3 and the output resistor R7 when determining the value of R_L?
   • Does this affect other amplifiers (such as common emitter/source)?
   • Which method/terminology should I use for the test(s)/homework?
3. Study what he/she tells you will be used in the coursework. If it is the same as the lecture material, know that the majority of the world will think of R_L as the output load, not "all resistors connected to the output terminal".

As an aside, I think your professor is trying to simplify the solution process a bit by combining R3 and R7 on the way towards solving for V_out. Be courteous and respectful, but don't leave until YOU understand what the professor is talking about and what he wants. YOU (or someone on your behalf) is paying for your education, it is up to YOU to get the most value out of the experience!

I would be interested in hearing his/her responses.

Answer 3

Your professor is using sloppy notation. If you removed your professor's \$R_L\$, the amp would cease to work, so that picture is not appropriate for what you are calculating.

Let's step back a bit. In analyzing the Common Base configuration, you need to figure out the resistance "seen" from the output node. Take your left-hand diagram and simplify it under small-signal assumptions: \$C_3\$ can be ignored (shorted), \$V_{CC}\$ is grounded, etc. The resistance seen from the output node has three parts in parallel:

1. \$R_7\$, the output load for the CB amp. My preference would be to call this \$R_L\$.
2. \$R_3\$, the collector resistor; I would call this \$R_C\$.
3. The resistance "looking into" the collector of the BJT. This is calculated from the small-signal diagram, and I'll call it \$R_{intoCollector}\$.

Now you can see that your professor has assigned the label \$R_{L}\$ to \$R_L || R_C\$, and the label \$R_{out}\$ to \$R_{intoCollector}\$, as per the right-hand diagram. While the analysis is not incorrect, the labeling confuses matters.

By contrast, the book's \$R_{L}\$ is just \$R_{L}\$, and \$R_{out}\$ is \$R_C||R_{intoCollector}\$. However note that they have taken \$r_o\$ to infinity and have thus dropped \$R_{intoCollector}\$ from the expression for \$R_{out}\$.