0
\$\begingroup\$

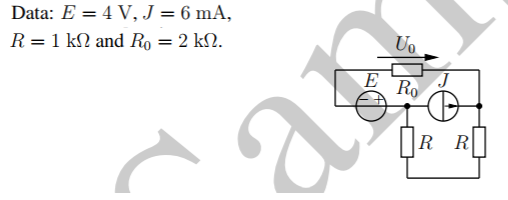
enter image description here

I want to do that by superposition rule.

Firstly i need to short the E source and compute U0 in case of current divider formula?

So i will get u1 which is voltage loss when the voltage source is short $$ U1=6mA\cdot \frac{2R}{2R+R0}$$ Then in second case i will use only E source. Now i have to find total current in that circuit which is equal $$ It=\frac{E}{2R+R0}$$ And then i just compute U2 which is voltage loss when we have our current source open $$ U2=1mA\cdot 2kOhm$$ And my result is $$ U0=U2+U1=5V $$ Am i right?

\$\endgroup\$
1
  • 2
    \$\begingroup\$ Start by correcting U1. There's 2R in parallel with Ro. Multiply the resulted resistance by J to get the J-part of Uo. Ask then again. \$\endgroup\$
    – user136077
    Commented Dec 10, 2017 at 23:41

1 Answer 1

Reset to default
0
\$\begingroup\$

With \$E\$ shorted out, you have \$R\$ in parallel with \$2 R\$. The \$J\$ current will divide between these. Select only the portion that goes through \$R_0\$ here and write it down.

Now return \$E\$ and remove (open) \$J\$. Now you have \$E\$ in a series loop containing two \$R\$ resistors and one \$R_0\$ resistor. Again, compute the current through \$R_0\$ here and write it down.

Now sum the two values. What do you get?

P.S. It may help a little that I also suggest you look at the direction of the current arrow for \$R_0\$. This may indicate a sign reversal to be thinking about.

\$\endgroup\$
4
  • \$\begingroup\$ Oh, okay i thought that second resistor is not needed, ten value od my u1 will be 3V. Current when J is open is 4V/4kOhm=1mA and now im calculating voltage loss on R0 which is R0*1mA=2V. Suming all up i get 5V. Am I correct now? \$\endgroup\$
    – c0rv
    Commented Dec 11, 2017 at 0:23
  • \$\begingroup\$ @c0rv There are two currents. What are you getting as the current through \$R_0\$ when shorting out \$E\$? \$\endgroup\$
    – jonk
    Commented Dec 11, 2017 at 0:33
  • \$\begingroup\$ To compute U1 i need Resistance and I, and value of I Is J? Or i am wrong? \$\endgroup\$
    – c0rv
    Commented Dec 11, 2017 at 1:23
  • \$\begingroup\$ @c0rv You have enough information to compute the current through \$R_0\$ with the voltage source shorted out. Do you see that fact? Or not? \$\endgroup\$
    – jonk
    Commented Dec 11, 2017 at 1:38

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.