Superposition principle and voltage division

Question

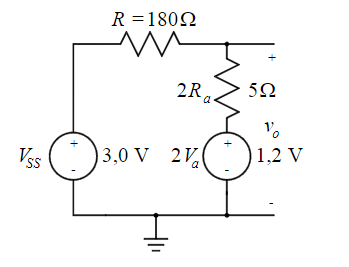
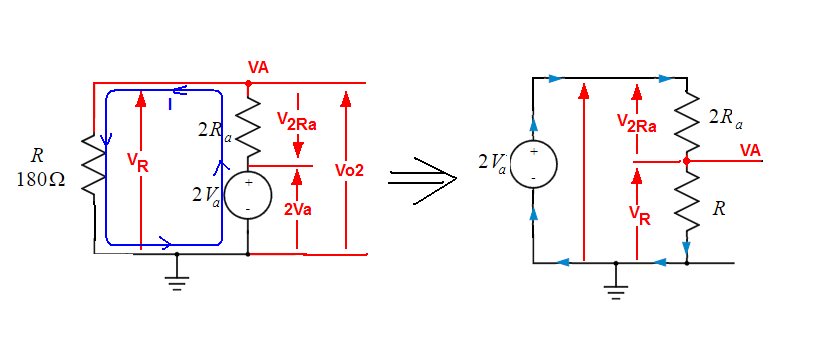
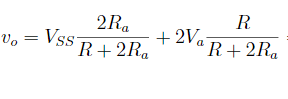I am looking at some circuit solving using the superposition principle and I came across this one.

We need to find \$v_0\$. My thought would be to use superposition and voltage division in the following way:

\$v_0=V_{ss}\cdot\frac{2R_a}{2R_a+R}+2V_a\cdot \frac{2R_a}{2R_a+R}\$

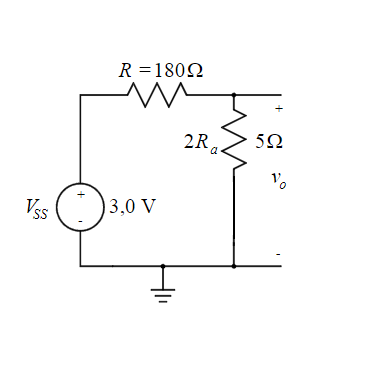
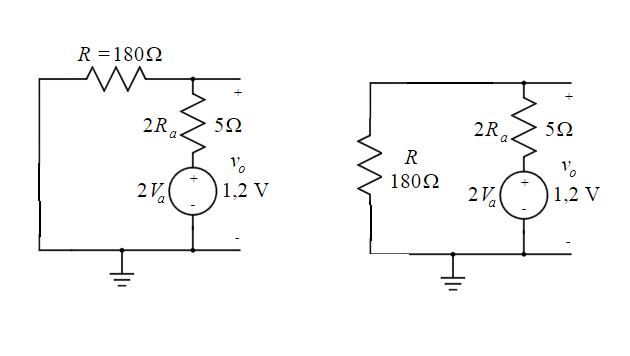
We in both cases find the voltage across \$2R_a\$ with the voltage divider formula. However, it turns out the solution is actually:

So my denominator and numerator should switch places in the second part of my solution, but I don't understand why this is. Aren't we then finding the voltage across \$R\$? And does that have an effect on \$v_o\$?

I hope someone can clarify this for me.

Answer 1

Hmm.

First I remove \$2V_a\$ source. So we left with this:

And we can find \$V_{O1}\$

$$V_{O1} = V_{SS} \frac{2R_a}{R+ 2R_a} = 0.081V = 81mV$$

Nowe we turn-off the \$V_{SS}\$ source and we are ending with this circuit:

And now we can solve for \$V_{O2}\$

As you can see this time \$V_{O2}\ = I\cdot R\$ or \$V_{O2} = 2V_a - I\cdot 2R_a\$

Where:

\$I = \frac{2V_a}{R+2R_a}\$ therefore:

$$V_{O2} =I\cdot R =\frac{2V_a}{R+2R_a}\cdot R = 2V_a \frac{R}{R+2R_a} = 1.167V $$

and finally

$$V_O =V_{O1}+V_{O2} = V_{SS} \frac{2R_a}{R+ 2R_a} + 2V_a \frac{R}{R+2R_a} =1.248V $$

Your circuit looks like this:

I mark the voltage drop by the arrows and the arrow tip is pointing the "positive" side.

And from KVL we have:

$$2V_a = V_{2R_a} + V_R$$ and also notice that \$V_o2\$ is a voltage drop betwenn \$V_A\$ node and GND.

$$V_A = 2Va - V_{2R_a} = 2Va - I*{2R_a} $$ or becouse \$V_A = V_R = I*R\$

Do you see it?