1
\$\begingroup\$

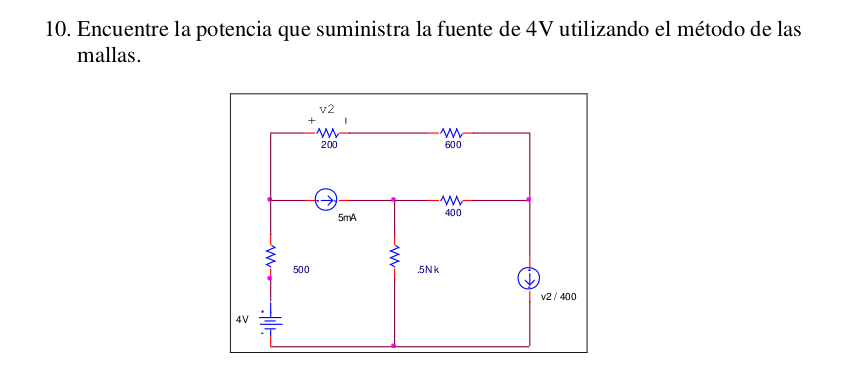
Translation: Find the supplied power from the 4V Source using meshes method enter image description here

I proposed five equations related with the circuit but I only find contradiction cause I find Rv value fixed. I suspect there should be voltage drops in current sources but I am not sure I think I am doing something conceptually wrong.

Here my calcuations enter image description here

I skipped some steps but I hope you can follow me.

I am currently getting I2=I3 = 0 which makes the first two equations to fix Rv. By my understanding it is a contradiction.

Note: .5Nk is a name for the resistor value (proportional to N), I have just called it Rv.

\$\endgroup\$
5
  • 1
    \$\begingroup\$ Welcome to EE.SE. Did you consult with your teacher? They are there to help you, but like us we will not solve your problem for you. Maybe someone will give you clues. \$\endgroup\$
    – user105652
    Commented Mar 3, 2018 at 21:34
  • \$\begingroup\$ \$I_2\ne I_3\$ is trivial to see. It's clear that \$V_2=200\cdot I_3\$. Substituting that into your other current source, you have:$$I_2=\frac{V_2}{400}=\frac{200\cdot I_3}{400}=\frac{I_3}{2}$$ So, they aren't equal. \$\endgroup\$
    – jonk
    Commented Mar 3, 2018 at 21:40
  • \$\begingroup\$ I will consult to teacher but I would like to see what do you think about my reasoning, I always form my opinion by different people thoughts . Also I am getting I2=I3=0 Which does not contradict I2=I3/2 \$\endgroup\$
    – Ariel Santiago Nowik
    Commented Mar 3, 2018 at 21:47
  • \$\begingroup\$ @ArielSantiagoNowik Okay. True enough. It's not a contradiction if both are 0. I'll write something up. However, I'll treat \$R_V\$ as simply that and let you worry about the N, etc. \$\endgroup\$
    – jonk
    Commented Mar 3, 2018 at 21:52
  • \$\begingroup\$ I know it is not what the teacher asked. But almost everything in this problem can be pretty easily written in terms of V2. The upper leg current is V2/200. The voltage across the 600 Ohm resistor is 3*V2. If you sum currents above the dependent current source, then you will see that the current flowing to the left is V2/400. So the current going into Rv is 5mA + V2/400. If nothing else, this can help you check your answer later. \$\endgroup\$
    – user57037
    Commented Mar 4, 2018 at 1:30

2 Answers 2

Reset to default
1
\$\begingroup\$

Let's set up the mesh equations as you have the schematic drawn up:

$$\begin{align*} 0\:\text{V}-200\cdot I_3-600\cdot I_3-400\cdot\left(I_3-I_2\right)-V_{5\:\text{mA}}&= 0\:\text{V}\\\\ 0\:\text{V}-R_V\cdot\left(I_2-I_1\right)-400\cdot\left(I_2-I_3\right)-V_{V_2\over 400}&=0\:\text{V}\\\\ 0\:\text{V}+4\:\text{V}-500\cdot I_1+V_{5\:\text{mA}}-R_V\cdot\left(I_1-I_2\right)&=0\:\text{V}\\\\ I_1-I_3&=5\:\text{mA}\\\\ I_2=\frac{V_2=200\cdot I_3}{400}&=\frac{I_3}{2} \end{align*}$$

This provides 5 equations and five unknowns: \$I_1\$, \$I_2\$, \$I_3\$, \$V_{5\:\text{mA}}\$, \$V_{V_2\over 400}\$.

Note that this includes the fact that there are, in fact, voltage drops across your current sources. Those are just two more variables, as shown.

Solving this yields 5 functions which depend upon \$R_V\$.

I can't tell you if \$I_2=I_3=0\:\text{A}\$ without knowing more about \$R_V\$. And I don't understand \$.5\:N\:k\$. (Does it mean \$500\cdot N\$?) So I'm stuck at this point, if you are looking for numerical results that aren't functions of \$R_V\$ (or N.)

(You can get \$I_2=I_3=0\:\text{A}\$ if and only if \$R_V=300\:\Omega\$. For obvious reasons.)

\$\endgroup\$
0
\$\begingroup\$

If you want to avoid the voltage drops across the current sources, you can write an equation for the loop that doesn't have any current sources (around the outside and down the middle). Use this as your third equation rather than the one you're using now.

\$\endgroup\$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.