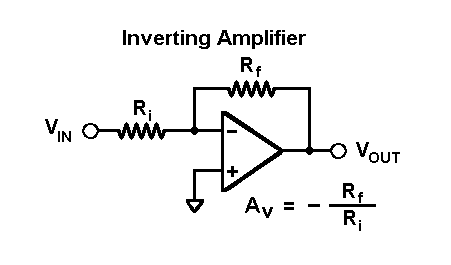
I normally would just rush forward with the obvious voltage divider equation. But I'm going to assume, because you reference it, that you want each voltage source activated one at a time while all other voltage sources are "shorted." It's more busy work. Oh, well.
You only have two voltage sources, \$V_\text{IN}\$ and \$V_\text{OUT}\$. So this isn't too much work. With \$V_\text{IN}\$ active and \$V_\text{OUT}=0\:\text{V}\$. There is a current \$\frac{V_\text{IN}}{R_i+R_f}\$. With \$V_\text{OUT}\$ active and \$V_\text{IN}=0\:\text{V}\$. There is a current in the opposite direction, \$\frac{V_\text{OUT}}{R_i+R_f}\$. The sum of these two superimposed currents is then \$\frac{V_\text{IN}}{R_i+R_f}-\frac{V_\text{OUT}}{R_i+R_f}\$. To find the voltage at the inverting terminal, we start with \$V_\text{OUT}\$ and add the voltage drop across \$R_f\$, so we find that:
$$\begin{align*}
V_-&=V_\text{OUT}+R_f\cdot\left(\frac{V_\text{IN}}{R_i+R_f}-\frac{V_\text{OUT}}{R_i+R_f}\right)\\\\
&=V_\text{OUT}+R_f\cdot\frac{V_\text{IN}-V_\text{OUT}}{R_i+R_f}\\\\
&=\frac{V_\text{OUT}\:R_i+V_\text{OUT}\:R_f+V_\text{IN}\:R_f-V_\text{OUT}\:R_f}{R_i+R_f}\\\\
&=\frac{V_\text{OUT}\:R_i+V_\text{IN}\:R_f}{R_i+R_f}\label{eq1}\tag{1}
\end{align*}$$
But you know, given that the non-inverting terminal is grounded, that \$V_\text{OUT}=-G\cdot V_-\$. So:
$$\begin{align*}
V_-&=\frac{V_\text{OUT}\:R_i+V_\text{IN}\:R_f}{R_i+R_f}\\\\
&=\frac{-G\: V_-\:R_i+V_\text{IN}\:R_f}{R_i+R_f}\\\\&\therefore\\\\
V_-&=V_\text{IN}\frac{R_f}{R_f+R_i\left(G+1\right)}=V_\text{IN}\frac{1}{1+\frac{R_i}{R_f}\left(G+1\right)}
\end{align*}$$
Then,
$$\begin{align*}
V_\text{OUT}&=-G\cdot V_-=-G\cdot V_\text{IN}\frac{R_f}{R_f+R_i\left(G+1\right)}\\\\&\therefore\\\\
\frac{V_\text{OUT}}{V_\text{IN}}&=-G \frac{R_f}{R_f+R_i\left(G+1\right)}\\\\
&=\frac{-R_f}{\frac{R_f}{G}+R_i\frac{G+1}{G}}=\frac{-R_f}{\frac{R_f+R_i}{G}+R_i}
\end{align*}$$
As \$G\to\infty\$ then \$\frac{V_\text{OUT}}{V_\text{IN}}=\frac{-R_f}{R_i}\$.
Not sure if that's what you wanted, though.
Nodal, done my way
I gather you worry about keeping the signs right. I also worry about that, too. I dislike, with some vigor, the way that nodal analysis is usually taught, where you have to consistently keep track (and continually re-inforce it mentally) of what is being subtracted from what. So here is my nodal approach that makes it a lot easier (for me) and I never worry about signs.
I set up the nodal equations such that outflowing currents are on the left and inflowing currents are on the right. Note that there are no minus signs anywhere.
Imagining myself as standing in the middle of the \$V_-\$ node:
$$\begin{align*}
\frac{V_-}{R_i}+\frac{V_-}{R_f}&=\frac{V_\text{IN}}{R_i}+\frac{V_\text{OUT}}{R_f}\\\\
V_-\left(\frac{1}{R_i}+\frac{1}{R_f}\right)&=\frac{V_\text{IN}}{R_i}+\frac{V_\text{OUT}}{R_f}\\\\
V_-\left(\frac{1}{R_i}+\frac{1}{R_f}\right)\frac{R_i\:R_f}{1}&=\left(\frac{V_\text{IN}}{R_i}+\frac{V_\text{OUT}}{R_f}\right)\frac{R_i\:R_f}{1}\\\\
V_-\cdot\left(R_f+R_i\right)&=\left(V_\text{IN}\:R_f+V_\text{OUT}\:R_i\right)\\\\
V_-&=\frac{V_\text{OUT}\:R_i+V_\text{IN}\:R_f}{R_i+R_f}\label{eq2}\tag{2}
\end{align*}$$
You can see that eq. \$\ref{eq1}\$ matches eq. \$\ref{eq2}\$.
It's a lot easier, I think, to handle the signs consistently and flawlessly, time after time, this way. Mentally stand in the middle of each node and just put all the outflowing currents on the left; all the inflowing currents on the right; and the rest just follows trivially. I learned this technique by observing source code for a Spice program and seeing how it handled the equation setup. Made a lot of sense to me and I've never gone back to the book-learned way, which I now consider to be designed for failure.
(Now imagine that you had to include an input impedance for the opamp. Easy. Let's call that input impedance, \$R_\text{in}\$. On the left side, you'd simply add \$\frac{V_-}{R_\text{in}}\$. On the right side, you'd add \$\frac{V_+}{R_\text{in}}\$. That's it. The process is trivial to follow. You almost cannot mess up.)