Differential amplifier circuit, problem with saturation

Question

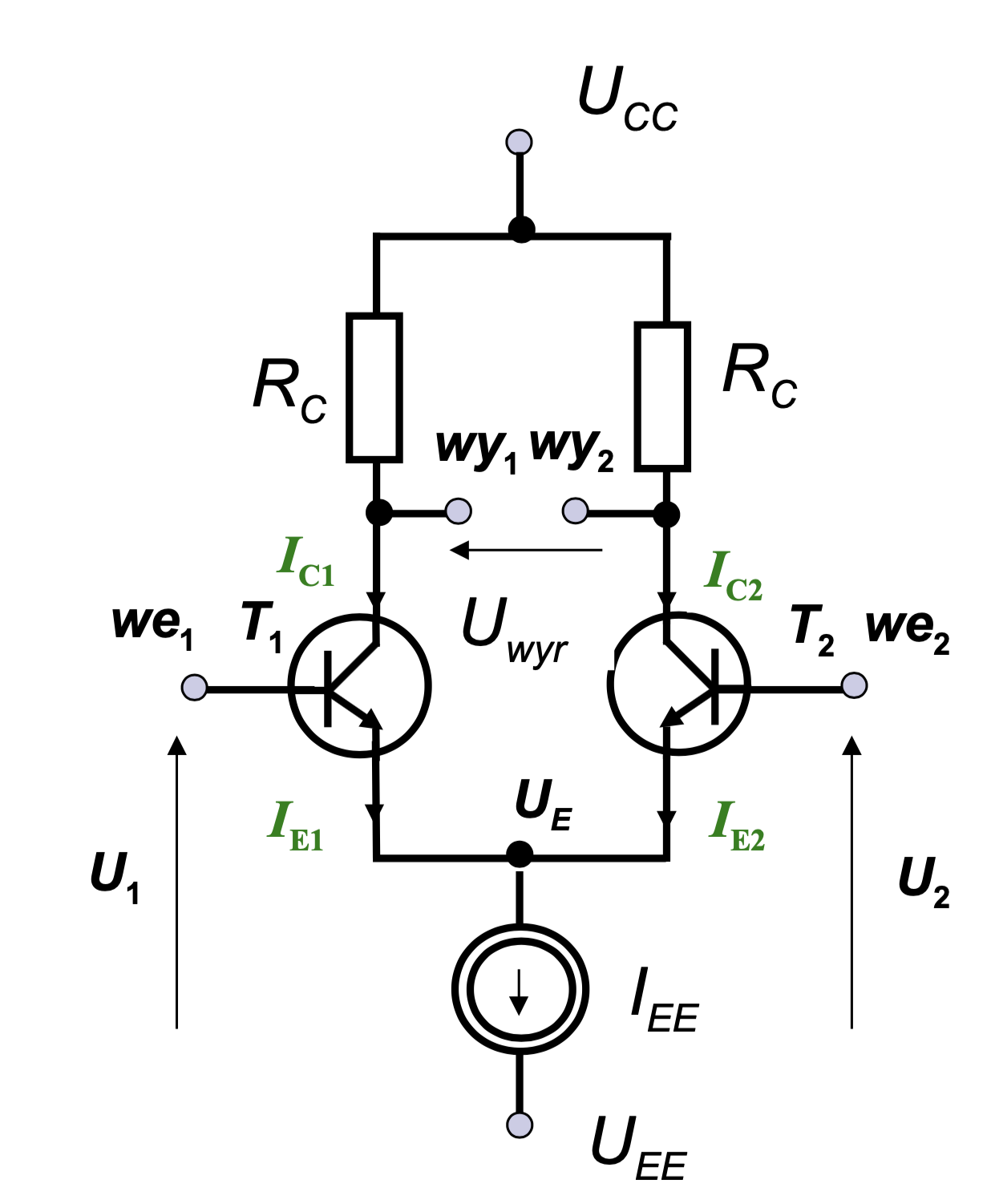
I have a homework problem regarding differential amplifier.

We have a differential amplifier with symmetric supply (i.e. \$V_{CC}=-V_{EE}\$). Emitters are connected to a perfect current source \$I_{EE}\$. T2's base is connected to the ground. T1's base (circuit's input) is connected to a sin wave generator, \$V_{PPUIN1}=2mV, \ \overline{V_{IN1}}=0V\$. We also know that \$\overline{V_{OUT2}}=8V, \ V_{PPOUT2}=400mV\$, where \$V_{OUT2}\$ is "wy2" in the image below. \$R_C=5k\Omega, \ V_{CESAT}=0.2V, \ V_{BE}=0.7V\$

1. Calculate \$I_{EE}, \ V_{CC}\$
2. \$V_{IN1}\$ was changed to \$-1V\$. Calculate \$V_{OUT1}, \ V_{OUT2}\$
3. Draw the transfer characteristic for both outputs for \$V_{IN1}\in<-1V;1V>\$

Since \$U_{IN}=U_{IN1}<2\varphi_T\$, then we assume that both transistors are active. Hence we can use this formula (I am not familiar with English naming convention, so pardon me, if my guess is incorrect):

\$G_{DIFF2}=\frac{g_mR_C}{2}=\frac{I_{EE}R_C}{4\varphi_T}=\frac{V_{PPOUT2}}{V_{PPUIN1}}=\frac{400mV}{2mV}=200V/V\rightarrow I_{EE}=4mA\$

The average value at the second output is \$\overline{V_{OUT2}}=V_{CC}-\frac{I_{EE}R_C}{2}\rightarrow V_{CC}=18V\$

And now let's calculate for what \$U_{IN1}\$ T1 becomes saturated:

\$V_{CC}-I_{EE}R_C-V_{CES}=V_{IN1}-V_{BE}\\ V_{IN1}=-1.5V\$

so.. it seems like our assumption was wrong and both transistors are not active. But from the other side, if T1 is saturated, then T2 must be cut off... which does not make sense, since we were given the information, that there is a sine wave at the second output. At this point I don't know what to do next

Answer 1

I am afraid you (we) were wrong here. Just take a look at this

As you can see from the diagram we have:

\$V_{CC} = 15V, I_{EE} = 1\textrm{mA}, Rc = 12\textrm{k}\Omega \$

And as you can see from the transfer characteristic the output voltage at the \$T_2\$ collector is equal to \$3V\$ for \$-1V\$ at the input (T1 base).

\$V_{out2} = 15V - 1\textrm{mA}\cdot 12\textrm{k}\Omega = 3V\$

And also notice that \$T_2\$ is not in the saturation region in this case.

Because while \$T_2\$ collector is at \$3V\$. The \$T_2\$ base is at \$0V\$ Therefore, the base-collector junction is reverse biased.

And the emitter voltage is around \$-0.7V\$ and \$V_{CE2} = 3V - (-0.7V) = 3.7V\$

And we can easily calculate the point at which \$T_1\$ transistor enters the saturation region. For example, if we assume \$V_{CE2(sat)}=0.2V, \ V_{BE}=0.7V\$ we can solve for the minimum voltage needed at the input to saturated the \$T_1\$ transistor.

\$U_{1(sat)} = 3V - 0.2V + 0.7V = 3.5V\$

And I hope that this does not need any further explanations?

But let me back to your current problem and this question:

2. \$V_{IN1}\$ was changed to \$-1V\$. Calculate \$V_{OUT1}, \ V_{OUT2}\$

In this circuit, we have \$ V_{CC}=18V, Rc = 5 \textrm{k}\Omega, I_{EE} = 4\textrm{mA} \$

It should be clear for you that such a large negative voltage at the \$T_1\$ base will cut-off \$T_1\$ and allows all the \$I_{EE}\$ current to flow through the \$T_2\$ transistor (T1 cannot be a turn-on because this would cause a very large voltage across T2 base-emitter junction).

If so, the voltage at the \$T_2\$ collector is:

\$V_{OUT2} = 18\textrm{V} - 4\textrm{mA}\cdot 5\textrm{k}\Omega = -2\textrm{V}\$

But notice that the \$T_2\$ base is at \$0V\$ and \$T_2\$ emitter voltage is at \$-0.7V\$.

And the collector-emitter voltage is \$V_{CE2} = -2V - (-0.7V) = -1.3V\$

But in NPN transistor we cannot have a negative voltage between collector and emitter (collector voltage cannot be lower than the emitter voltage).

So, this result tells us that our BJT (\$T_2\$) is in saturation region for \$-1V\$ at the input.

Therefore:

\$V_{out2} = V_{E2} + V_{CE(sat)} = -0.7+0.2V = -0.5V\$

And the \$T_2\$ collector current is

\$ I_{C2(sat)} = \frac{V_{CC} - V_{out2} }{R_{C2}} = 3.5\textrm{mA}\$

The base current is \$I_{B2} = I_{EE} -I_{C2(sat)} = 4\textrm{mA} - 3.5\textrm{mA} = 0.5\textrm{mA} \$

I hope that this explains why \$T_2\$ is indeed in the saturation region for \$-1V\$ at the input (T1 base). Because here we have a different situation than shown in your example transfer characteristic.