how to get the line voltage of 3 phase delta circuit with power factor known at first?

Question

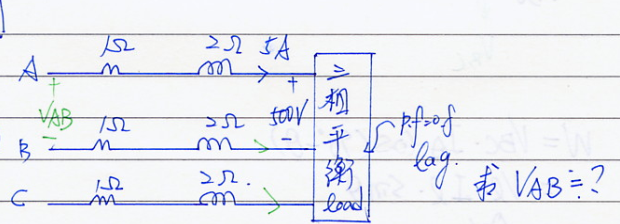
In the following picture, it shows that a black box at right. the circuit inside the black box is a 3 phase load balanced delta connection circuit. the line voltage of it is 500V and the power factor of it is 0.8 lag. then, I wanna get the voltage difference between A and B.

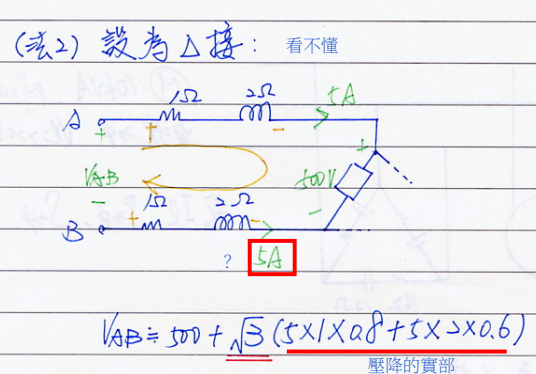
In the second picture, it shows the formula to get voltage wanted. However, I don't know why that there is a 3^(1/2) inside the formula. Can someone help me?

BTW, don't use the trick to change the delta circuit to star connection circuit. even though I know that it will be much easier to get the answer. I just wanna know how to get the voltage between A and B by delta connection.

Answer 1

there is a square root of 3 in the three phase power formula to begin with and seeing the terms I guess it came from there.

I think there are a lot of mistakes or at least a lot of things that need clarifying though

First if I assume those lines are resistors and inductors, their impedances are complex numbers, the inductor needs to be expressed as jwl(or i for imaginary number) you have the amount in ohms there so make it a phasor and the angle should be -90 if you add that resistor and that inductor together you get a line impedance around 2.2 ohms with an angle of 60 degrees. This is approximate and off my head, if you go through it there will be decimals.

The modules of the currents in A and B are the same at 5 Amperes in a balanced system, but their angles cannot be unless your system is sequence 0 or phase 0, but then the current inside the delta would be 0 making the power 0 on the load. if you make the A current the phase or angle reference then the B current is 120 degrees off at the very least. At least in the calculations you are doing I cannot see any of this.

In my opinion there are a lot of things you need to organize before moving on.

Please check what I mentioned.