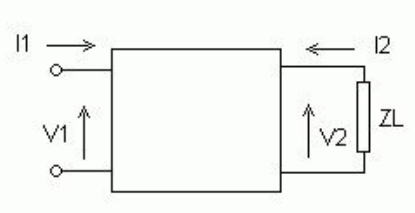
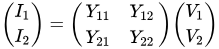I have the following two port network:
for which the Y matrix elements are:
- \$y11(s): (2+3s)\$
- \$y12(s): -1\$
- \$y21(s): -1\$
- \$y22(s): 1.5\$
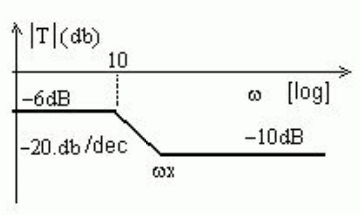
I need to get the value of \$ZL\$ in order for the transfer function of the circuit to corresopnd to the following Bode plot:
From the two port network: Based on the equations of the network,
and the fact that \$V_2 = -I_2*Z_L\$, writing the following equations system:
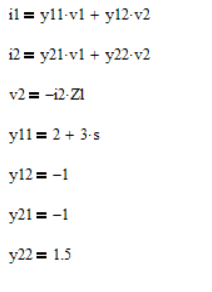
I get the transfer function \$H(s)=\frac{2*Z_L}{2+3*Z_L}\$
On the other hand, to find the Transfer function of the given Bode, I proposed:
- num. of decades between two frequencies: $$log\frac{\omega_x}{10} = \frac{4}{20} \implies \omega_x = 10^\frac{4}{20}$$
- In \$\omega_0\$ there is a single pole.
- In \$\omega_x\$ theres a single zero.
Therefore: \$H(s)=k\frac{(\frac{s}{\omega_x} + 1)}{\frac{s}{10} + 1}\$
Where \$k=0.5\$
With all that, I get the following value for \$Z_L\$:
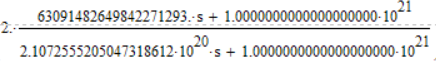
which has absurd numbers, and therefore I suspect I'm doing something wrong, although I'm not seeing where.
Thanks in advance for any help you may give.