0
\$\begingroup\$

I kept a coil in a linearly increasing magnetic field and calculated the impedance by measuring the induced current and voltage.

When the maximum value of magnetic field was reduced (while keeping the rate of increase of magnetic field with time constant) the impedance increased.

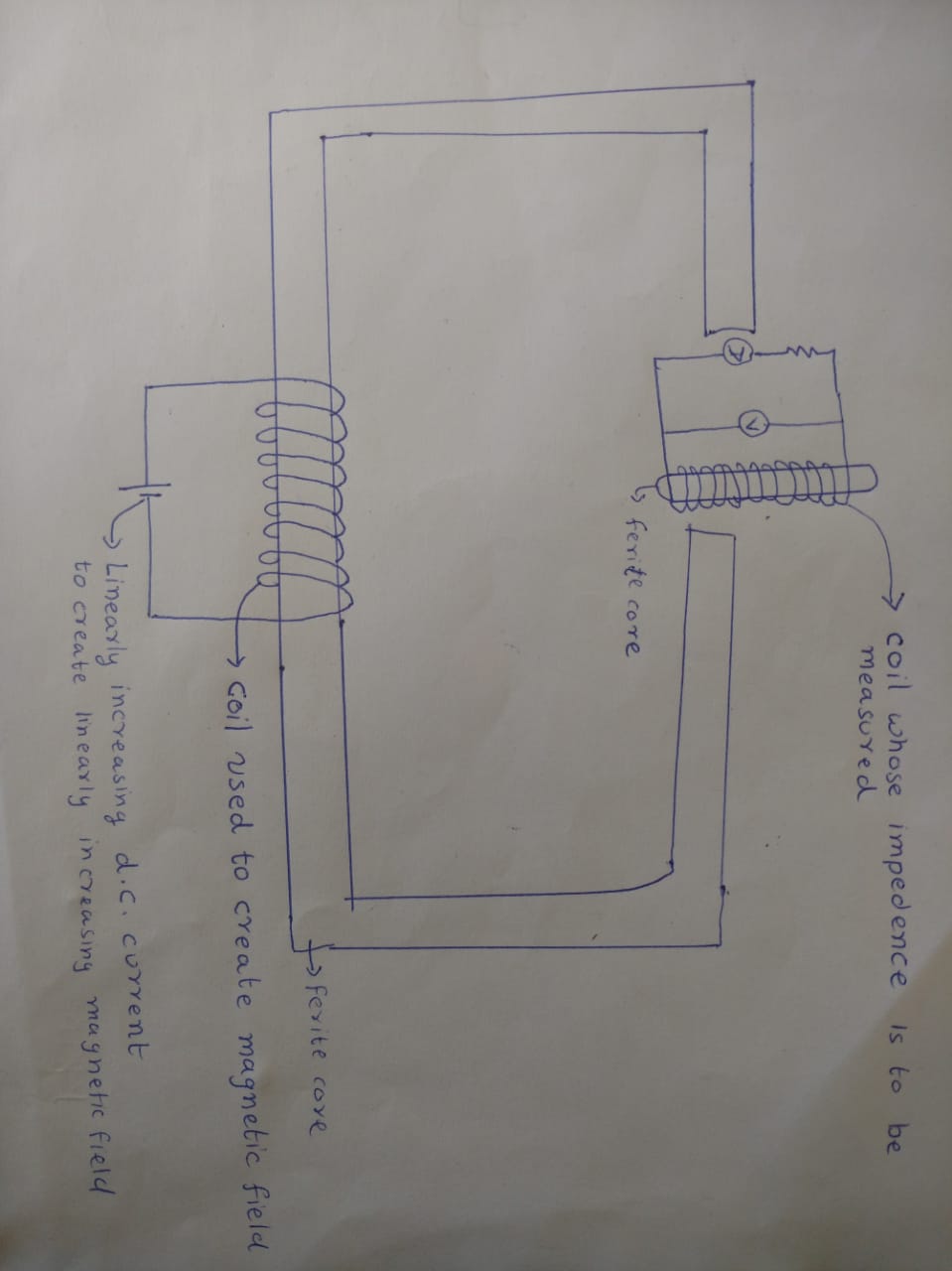
What might be the reason for this?enter image description here

\$\endgroup\$
4
  • \$\begingroup\$ can you add the formula you used to calculate the impedance to your question? If we're using the same notation as you do, it's probably easier to understand what we explain! \$\endgroup\$
    – Marcus Müller
    Commented Jun 8, 2019 at 14:26
  • 2
    \$\begingroup\$ Air cored, or iron cored? \$\endgroup\$
    – Neil_UK
    Commented Jun 8, 2019 at 14:29
  • \$\begingroup\$ Inductance L is linearly proportional to impedance X(f) and energy 1/2LI^2 and B field \$\endgroup\$
    – D.A.S.
    Commented Jun 8, 2019 at 14:45
  • 1
    \$\begingroup\$ "calculated the impedance by measuring the induced current and voltage" makes no sense. Voltage is induced (a la Faraday) not current and, on another level, explain exactly how you made the measurement. \$\endgroup\$
    – Andy aka
    Commented Jun 8, 2019 at 18:10

2 Answers 2

Reset to default
1
\$\begingroup\$

This depends on the inductor. If it is air cored, no.

However many inductors have some ferromagnetic core material, iron or ferrite, which have a much higher permeability, typically giving you hundreds of times as much inductance from the same winding.

(There are other reasons for ferromagnetic cores - they direct the mgnetic flux where you want it, for example into the rotor in a motor, or prevent stray magnetic field affecting other circuits) But the main effect is to multiply the magnetic field strength.

However any ferromagnetic material will saturate at a specific field strength - about 0.3 Tesla for a typical ferrite or just over 1 Tesla for iron. The onset of saturation can be gradual, or relatively sudden, and the details depend on the ferrite or iron alloy composition. It is the portion of the B-H curve where it flattens out.

The answer to the question is that, at saturation, increased current results in less (or no) increase in magnetic field strength - and this is measurable as a reduction in inductance.

This is exploited in saturable reactors, sometimes known as "magnetic amplifiers" where changing a DC bias current, to control saturation, changes the gain of a circuit.

\$\endgroup\$
0
\$\begingroup\$

Inductance is not constant with changing the strength of the induced magnetic field. In the figure below the flux linkage (magnetic flux times number of turns) is plotted against the current through a coil. Keeping this in mind inductance is defined as the change of flux linkage over the change in current. This means that inductance is the slope of the tangent to the function plotted in the figure below. As the current through the coil is increased (higher values of magnetic flux) the relationship looses its linear behavior and flattens a bit. This results in lower values of inductance.

Relationship between flux linkage and current through the coil

\$\endgroup\$
2
  • 1
    \$\begingroup\$ This result is only valid for inductors that have ferrous cores. An air-core inductor would not show this effect, or if it did it would only be at insanely high field strengths of interest only to theoretical physicists. \$\endgroup\$
    – TimWescott
    Commented Jun 8, 2019 at 17:27
  • \$\begingroup\$ You are absolutely correct. I think the answer of Brian Drummond explains it better. I forgot to discuss the reasons for this in my answer which is saturation, and saturation in air happens at very high field strengths which are not of interest as you mention. \$\endgroup\$
    – Ivan Vlaykov
    Commented Jun 9, 2019 at 7:27

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.