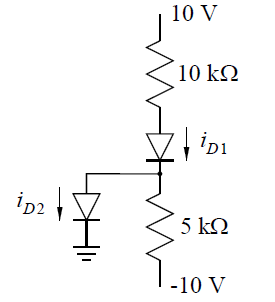0
\$\begingroup\$

I have a problem. I have this circuit:

enter image description here

I'm told that this is a limiter circuit. The question goes exactly like this:

"Assuming the diodes are silicon diodes (0.6V voltage drop needed in forward bias) what interval is this limiter circuit allowing \$v_o\$ to be in?

  • 0 to 5,2V
  • -5,2V to 0,6V
  • -0,6V to 5,2V"

I actually think I am capable of answering this question, but I don't understand what \$v_o\$ is. There are no schematics other than the one above so I'm very confused on how to arrive at an answer.

I hope someone can clarify this for me or give me a hint on how to arrive at the answer.

\$\endgroup\$
6
  • \$\begingroup\$ That subscript definitely must be the o from obvious! \$\endgroup\$
    – Huisman
    Commented Jan 31, 2020 at 21:19
  • \$\begingroup\$ Huh? I don’t understand. Are you joking? If so, then - ha ha haa \$\endgroup\$
    – Carl
    Commented Jan 31, 2020 at 21:27
  • 1
    \$\begingroup\$ Yes, there is no \$v_o\$ in the schematic at all and as the schematic is presented all nodes have a fixed/determined voltage. So, to none of them applies the voltage range given in any answer. \$\endgroup\$
    – Huisman
    Commented Jan 31, 2020 at 21:35
  • \$\begingroup\$ Huh, well that is pretty unaccptable. At least now I know I’m not alone in thinking that :) \$\endgroup\$
    – Carl
    Commented Jan 31, 2020 at 21:39
  • \$\begingroup\$ BTW, English has a point as decimal separator \$\endgroup\$
    – Huisman
    Commented Jan 31, 2020 at 21:56

1 Answer 1

Reset to default
1
\$\begingroup\$

There are only two non-trivial nodes : it's reasonable to assume Vo is one of them.

One approach is to assume each in turn is Vo and solve for that.

If both yield the same answer, ... good.

If they yield different answers, you can either report both, or pick one and clarify your assumption as part of the answer.

EDIT following comments...

Hmm, I can't seem to get an answer that matches any of the three intervals stated in the problem. Depending on what node I interpret as Vo, I get these two intervals: Either Vo ∈ {-3,5V ; 0,6V} or Vo ∈{-2,9V ; 1,2V}.

I don't see anything wrong in your result.

One of those ranges is a subset of (i.e. contained entirely within) one of the given intervals.

I would answer on that basis, noting the ambiguities (and your choice of Vo) and discrepancies as part of the answer.

Funnily enough, this is a very common real world situation : ambiguous or incomplete specification and unchecked calculations, (here, possibly inherited from a different question with different resistor values, not re-checked). Learning an honest and diligent way of dealing with such a situation is a necessary life skill in engineering; not much taught in coursework.

\$\endgroup\$
3
  • \$\begingroup\$ I am unsure of which nodes you are referring to. I can see three nodes, where only one is non-trivial. One at the top at 10V, one at the bottom at -10V and one connecting the second diode to the rest of the circuit. \$\endgroup\$
    – Carl
    Commented Feb 2, 2020 at 11:55
  • \$\begingroup\$ You missed D2 cathode, and the connection between D1 anode and a resistor, making 5 nodes, of which three have defined voltages. \$\endgroup\$
    – user16324
    Commented Feb 2, 2020 at 13:30
  • \$\begingroup\$ Hmm, I can't seem to get an answer that matches any of the three intervals stated in the problem. Depending on what node I interpret as Vo, I get these two intervals: Either Vo ∈ {-3,5V ; 0,6V} or Vo ∈{-2,9V ; 1,2V}. I wonder if I'm even doing anything wrong.. \$\endgroup\$
    – Carl
    Commented Feb 2, 2020 at 18:18

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.