Biasing circuit with mosfet: am I missing information or misreading it?

Question

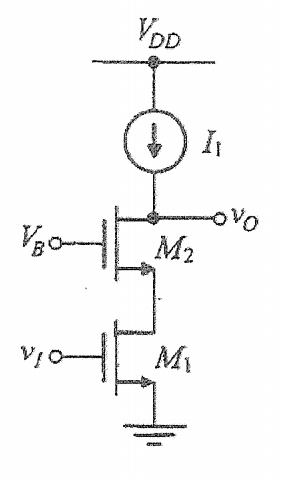
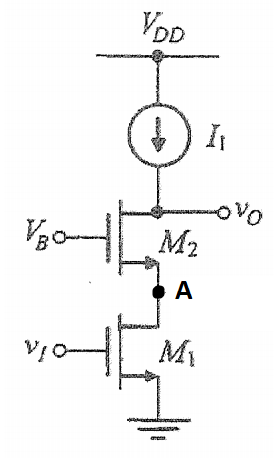
I have the following circuit with MOSFET (cascode amplifier):

Information given: $$V_t=1V$$ $$I_1=20\mu A$$ $$k=10 \mu AV^{-2}$$

Knowing that \$V_b\$ is 5V determine the minimum value of the output voltage so that both transistors are saturated.

I'm kinda confused on how to proceed on this.

First of all, don't we need VDD? How to relate VB with this? I started by assuming both transistors had the current I_1 (I assume this is correct).

Then I used the MOSFET equation for saturation $$i_D=k(v_ {GS}-Vt)^2$$ to determine \$v_{GS}\$ in both transistors. obtaining 2.4 V. Then I applied the boundary condition for saturation $$v_{DS}=v_{GS}-V_t$$ and so obtained for both transistors \$v_{DS}=1.4V\$ So the minimum value of \$v_O\$ is 2.8V.

This is incorrect, the answer should be 4V. I'm really not understanding how to proceed... Can someone give me an hint on how to do this? Thanks!

Answer 1

The current through both the MOSFETs is the same. Also it is given that both the MOSFETs are in saturation region. Therefore we can write: $$I_{D1} = I_{D2}= I_{D} = k(V_{GS} - V_t)^2 = 20 \mu A $$ on solving we get \$V_{GS}\$ should be \$2.4V\$ for both the MOSFETs, if \$ 20 \mu A\$ has to flow through both of them.

Now for M2, $$V_{GS} = 2.4V$$ $$ \implies V_B - V_A = 2.4 V $$ Given \$V_B\$ is 5V.

$$ \implies 5 - V_A = 2.4 V $$ $$\implies V_A = 2.6V$$

For M2 to enter saturation, \$ V_{DS}\$ of M2 should be atleast \$ V_{GS} - V_t\$ $$ V_{DS_{min}}= 2.4 - 1 = 1.4 V $$ At this point, \$ V_{DS} \$ of M1 will be: $$V_{DS(M1)} = V_A = 2.6V > 1.4V$$

So M1 will also be in saturation, well saturated over the minimum value of \$1.4V\$.

Now from the circuit we can express \$V_{DS}\$ of M2 as $$V_{DS} = V_o - V_A $$ $$\implies V_o = V_A + 1.4 = 4 V$$

If you look closely, the node voltage \$ V_A \$ decides the minimum output voltage. When M2 entered the saturation region (Boundary condition), M1 had already been saturated way before. So your assumption of having boundary conditions in both the MOSFETs at the same time, went wrong. And hence the calculations too.

Answer 2

If Vds of M1 is 1.4V and Vgs of M2 is 2.4V, how can Vb be 5V?

Vdd is not needed because I1 is an ideal current source.