RMS Current & Average Current Questions

Question

I understand that:

1. For purely DC current with fixed DC voltage, the DC & RMS current value will be the same, means that the total power RMS & DC will be the same using Pdc = Idc x Vdc

2. For pulsed current with fixed DC voltage (example flyback switching), RMS current value will be greater than DC current value, however the RMS will be not count in for power consumption, therefore the power consumption is still based on Pdc = Idc x Vdc

Based on that, meaning that in every calculation, I need to use DC current for power calculation, not RMS value.

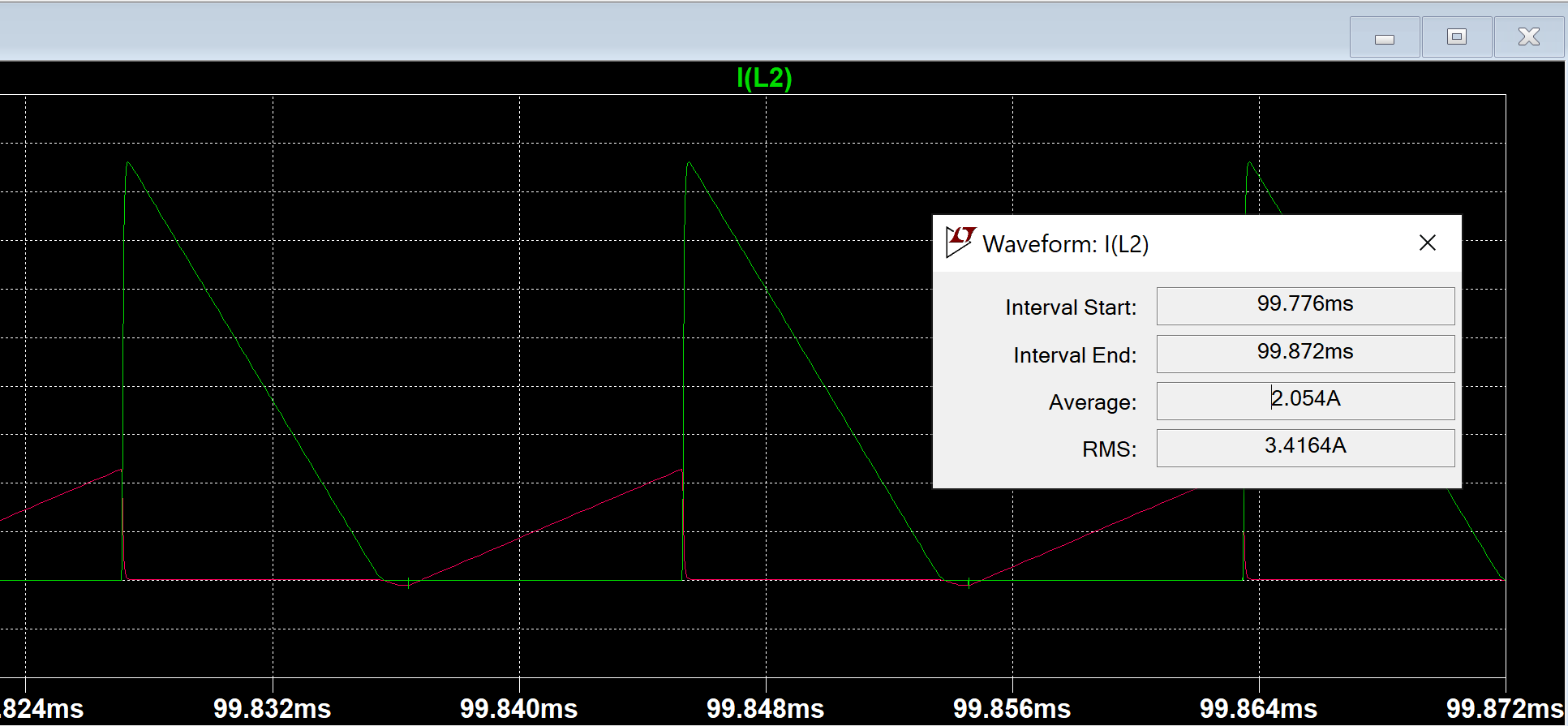
By using simulation, I managed to get RMS & average value at my flyback secondary side. Both value are correct based on my calculation. Refer image below:

Questions:

1. How to convert RMS current to average current? I noticed that most of power supply calculation will produce RMS current (example secondary RMS current, not secondary average current). Most of the time, I will use Pdc/Vout to get the average current, but how can I estimate the average current from the RMS current?
2. How can I measure this average current in actual condition? I noticed that most of my equipment (example oscilloscope, multi-meter) is based on RMS value & not average value.
3. What is the actual purpose of this RMS current value? When do we use this RMS value in real situation?

Thanks

Answer 1

how can I estimate the average current from the RMS current?

Generally you can't. For instance, think about a regular AC power waveform; the current will be a sinewave and a sinewave has an average value that is always zero (despite having a significant RMS value). However, if you want to understand how your half saw-tooth RMS value converts to "average", we can do this because we know what the waveform looks like. So, for approximately 58% of the time the waveform is zero (as per your drawing) and that means that all the energy is contained in the falling saw tooth part of the waveform (42%).

If that current waveform were a continuous saw-tooth, it would have an RMS value that is \$\dfrac{I_P}{\sqrt3}\$.

Here's the proof for a voltage waveform: -

This is the RMS formula for a triangle or saw-tooth waveform based on the peak value.

So, given that the peak current is around 9.1 amps (from your picture), AND the if waveform had no “dead time”, the RMS would be 5.25 amps. But, because we know that there is around 58% “dead time” after the saw tooth has fallen to zero, the square of that RMS value (the power) can be multiplied by 0.42 (then square rooted) to yield an effective RMS value of 3.41 amps. Your picture shows an RMS value of 3.42 amps: -

_{But, do you see that in order to go from RMS to average, you need to know a lot about the waveform shape. In other words there is no generic relationship.}

How can I measure this average current in actual condition

Use your meter set to measure DC current - it will average your true wave form.

What is the actual purpose of this RMS current value? When do we use this RMS value in real situation?

In your application it can be used to assess the \$I^2R\$ losses of the magnetics used in the flyback transformer (I recognize the waveforms in your question). The peak value of current can be used to assess the core saturation losses of the magnetics.