The explanation about why do we define the formula of Characteristic impedance,propagation constant and Velocity propagation

Question

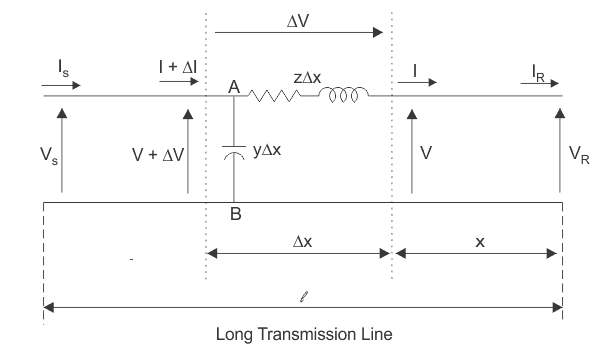
There is a long transmission line

1.Characteristic impedance \$Z_C=\sqrt{\frac{z}{y}}=\sqrt{\frac{impedance}{admittance}}\$,and in the lossless line,\$Z_C=\sqrt{\frac{L}{C}}\$

2.propagation constant \$r=\sqrt{yz}=\sqrt{admittance \times impedance}\$

3.Velocity propagation \$v=\frac{1}{\sqrt{LC}}\$

We will learn these formula above when we are learning the long transmission line,and i have three questions about these three formula.

Q1:

The definition of Characteristic impedance:the ratio of the amplitudes of voltage and current of a single wave propagating along the line,i know the ratio between \$A\$ and \$B\$ means \$\frac{A}{B}\$ ,but i still don't understand why can we define the Characteristic impedance \$Z_C=\sqrt{\frac{z}{y}}\$ according to its definition,can anyone tell me why?

Q2:

propagation constant \$r=\sqrt{yz}=\sqrt{admittance \times impedance}\$,why can we define that?because i don't think that there is any relation between "propagation" and " admittance and impedance" ,i think it is unreasonable when \$r=\sqrt{admittance \times impedance}\$ when there is no any relation between "propagation" and "admittance and impedance",why don't we just define \$r=\sqrt{y+z}\$ ,so can anyone know why can we define propagation constant like that?

Q3:

Why can we define Velocity propagation \$v=\frac{1}{\sqrt{LC}}\$?what is the relation between "Velocity or propagation" and "LC",L is inductance ,and C is capacitance.

Answer 1

The 2nd order form of Telegrapher's equation shows that pulses in the transmission lines propagate as waves and the term which shows the velocity of the propagation in the equation is your other square root term. In physics all wave motions obey an equation of the same form. The measurement unit of quantity 1/squareroot(LC) is m/s if L is Henrys per meter and C is Farads per meter.

If one solves voltage and current Telegrapher's equation as functions of place and time he founds that there's simultaneously waves to both directions. In a single wave the voltage and current at the same moment at the same place have always a certain ratio, the characteristic impedance. The squareroot of L/C really has measurement unit =Ohm.

The general solution of Telegrapher's equation in case of sinusoidal signals contains the propagation constant. It combines losses and the velocity to one complex number. It is how much phase lag and attenuation the line causes per unit length to the phasor which presents the propagating sinusoidal wave.

Propagation constant in its full form is used especially with lossy lines although by omitting the attenuation it works also perfectly with ideal lines.

Conclusion: The obscure looking definitions are based on the solution of Telegrapher's equation, not on the diagram in the question which is needed to derive Telegrapher's equation. The defined quantities fit perfectly into the solutions - the waves.