0
\$\begingroup\$

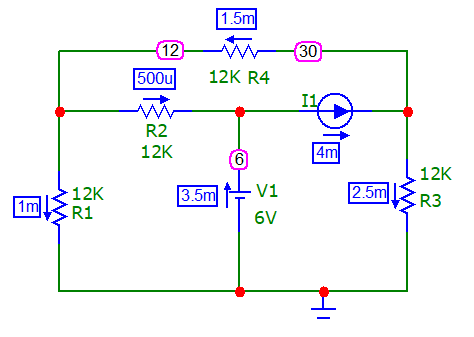
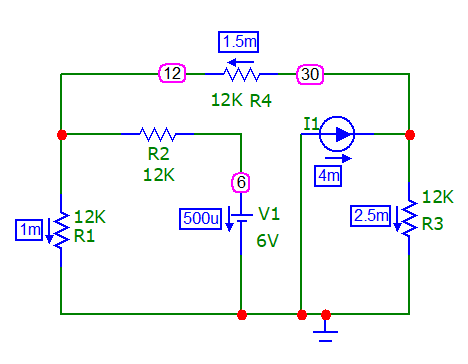
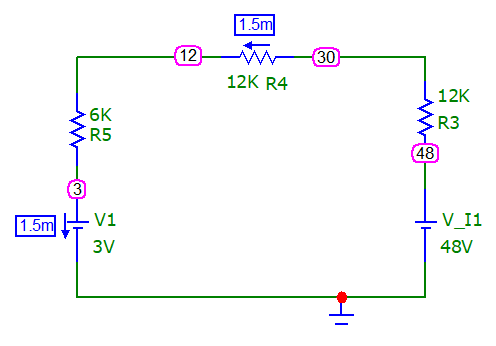
When I learned the superposition theorem, the book provided this circuit to me, and taught me how to use the superposition theorem to find the value of \$I_0\$:

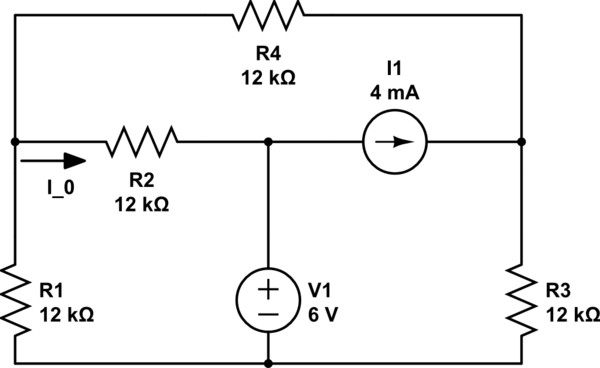
schematic

simulate this circuit – Schematic created using CircuitLab

However, I want to ask that why should use the superposition theorem to find the \$I_0\$ in this circuit? Must we use the superposition theorem to find the \$I_0\$?

I mean, is the superposition theorem the only method to calculate the \$I_0\$? If I have no idea about superposition theorem, that is, f I don't know what the superposition theorem is, I don't know there is a method called superposition theorem which can be used to calculate the \$I_0\$ value. Can we still use other methods to calculate the \$I_0\$? If yes, can anyone show me how to calculate the \$I_0\$?

\$\endgroup\$
2
  • \$\begingroup\$ Superposition implies that you identify the independent sources in the circuit (6 V and the 4-mA current source) and alternately turn them off to determine \$I_0\$: turn the 6 V off (replace it by a short circuit) while the 4-mA I-source is alive: determine \$I_{01}\$ in this mode. Then bring the 6-V source back on and turn the 4-mA off (open circuit it) and determine \$I_{02}\$. The current you want is simply \$I_0=I_{01}+I_{02}\$. I like superposition because it often leads to simple intermediate circuits you can solve by inspection only (no equation). \$\endgroup\$
    – Verbal Kint
    Commented Apr 22, 2020 at 8:57
  • 1
    \$\begingroup\$ Nodal analysis would work just fine. The big benefit of superposition is when you use both DC and AC sources in the same circuit. \$\endgroup\$
    – Adam Haun
    Commented Apr 22, 2020 at 15:46

1 Answer 1

Reset to default
5
\$\begingroup\$

i want to ask that why should use the superposition theorem to find the I0 in this circuit?must we use the superposition theorem to find the I0?

No, unless you are instructed to do so.

i mean is superposition theorem the only method to calculate the I0?

And...

can we still use some methods to calculate the I0? if yes!can anyone show me how to calculate the I0?

My natural instinct is to simplify....

So, I'd rearrange - the current source is attached to a grounded voltage source - that immediately allows it (the current source) to be moved directly across R3 - this simplifies any analysis because you can turn it into a voltage source of 48 volts in series with 12 kohm (R3).

I'd then rearrange V1, R1 and R2 into a 3 volt source in series with R1||R2 (= 6 kohm). It's simple math to see that the current through R4 flows right to left with a magnitude of 1.5 mA.

Drill down a bit more and the current though R2 is easily found (0.5 mA).

Simulation confirms: -

enter image description here

And, just in case anyone is perturbed by my suggested modification to split the current source from the voltage source and place it across R3: -

enter image description here

Then, convert I1 to a voltage source (I've called it V_I1 below) and rearrange the proper voltage source (V1), R1 and R2 into a source with a single resistor of 6 kohm (named R5) and it's really simple to find the current through R4.

enter image description here

As I said earlier, drilling down a little more finds I_0.

\$\endgroup\$
9
  • 1
    \$\begingroup\$ Nice solution, +1 \$\endgroup\$
    – Huisman
    Commented Apr 22, 2020 at 20:34
  • \$\begingroup\$ thanks a lot,this answer really helps me a lot,however,i have a question about your equivalent circuit,can you tell me is my thinking right or wrong?electronics.stackexchange.com/questions/495082/… \$\endgroup\$
    – shineele
    Commented Apr 23, 2020 at 6:09
  • \$\begingroup\$ Ehm, isn't this possible because of the super position principle?? Do you have a "proof" (next to your "natural instinc") this is different from applying the super position principle. (Not to critisize, I instinctively upvoted your answer as well and still support it). \$\endgroup\$
    – Huisman
    Commented Apr 23, 2020 at 7:15
  • \$\begingroup\$ Given that the analysis I did considers all energy sources simultaneously (albeit somewhat transposed), I don't see that it could be said that it is @Huisman \$\endgroup\$
    – Andy aka
    Commented Apr 23, 2020 at 7:53
  • \$\begingroup\$ @Andyaka So, could you please prove/explain why your method is valid? \$\endgroup\$
    – Huisman
    Commented Apr 23, 2020 at 9:08

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.