Is this reason why we can let the current source be attached to a grounded?

Question

In this question, someone shows me that these two circuit are equivalent. I want to make sure that my idea about why is these two circuits are equivalent, is correct.

The inner resistor of an ideal current source is infinity,and how much current that current source provide to the circuit, how much will the circuit feedback to the current source, I mean if a current of \$x\$ A flows from the current source, then that \$x\$ A current will flow into the same current source, that is the reason why we can let the current source be attached to a ground.

So if I connected the resistor next to the current source, the equivalent circuit is as below:

Is my thinking right?

Answer 1

The inner resistor of an ideal current source is infinity,and how much current that current source provide to the circuit, how much will the circuit feedback to the current source, I mean if a current of \$x\$ A flows from the current source, then that \$x\$ A current will flow into the same current source, that is the reason why we can let the current source be attached to a ground.

No. It is true that the same current that leaves the current source, has to enter it again. So, in the circuit below \$I_X=I_0+I_1 = x\$ A. But it is not the reason you can ground the current source. How would you know what value \$I_0\$ and \$I_1\$ have and conclude that you can simply connect the current source to ground?

^{simulate this circuit – Schematic created using CircuitLab}

The reason you can ground the current source is because you can apply the superposition theorem:

The superposition theorem states that in a linear circuit with several sources, the current and voltage for any element in the circuit is the sum of the currents and voltages produced by each source acting independently.

So,

• first take the contribution of the voltage source, zeroing out the current source, which implies it can be replaced by an open circuit. Note that in that partial circuit also the 100 Ω resistor can be removed.
• then take the contribution of the curent source, zeroing out the voltage source, which implies it can be replaced by a short circuit.

^{simulate this circuit}

Answer 2

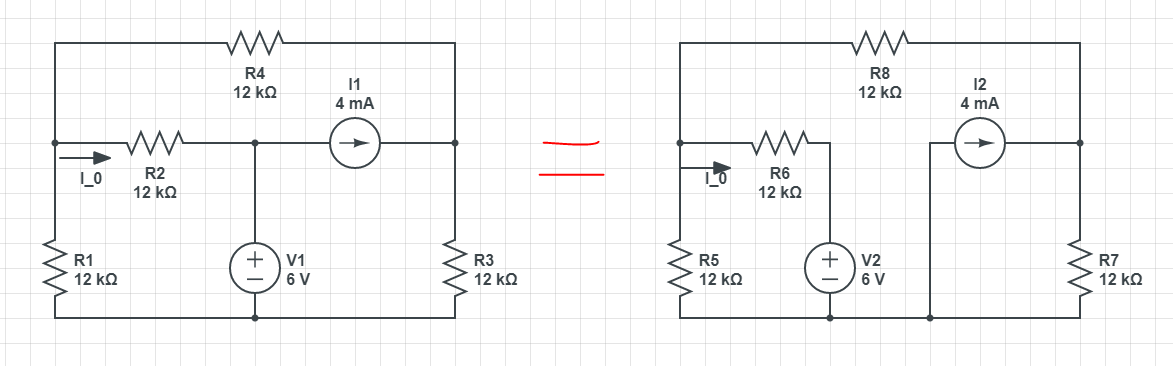
In this question, someone shows me that these two circuit are equivalent.

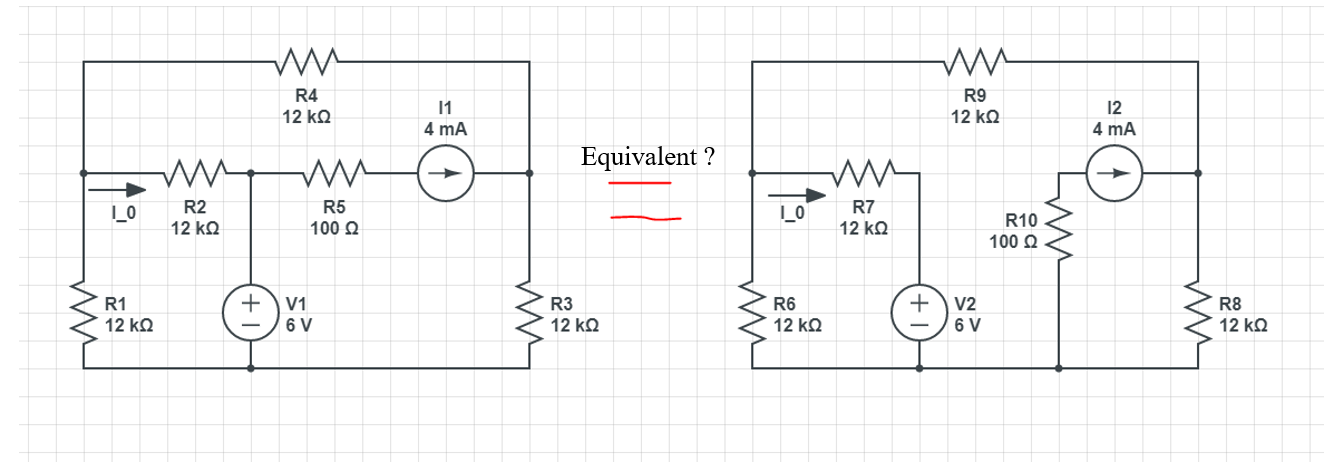
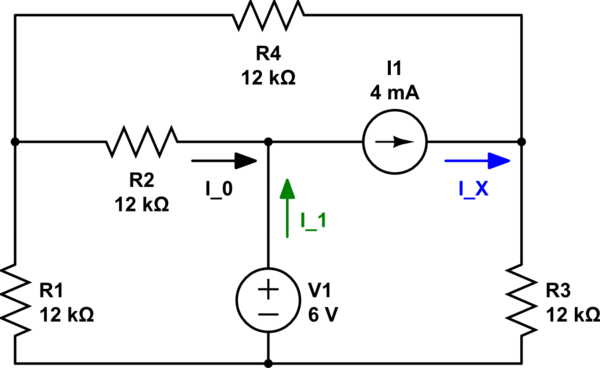
^{I didn't say that it was equivalent - I made a simplification that allowed me to find the current through R4 which then permits finding the current through R2. However, it can be made equivalent with one extra feature (splitting a current source): -}

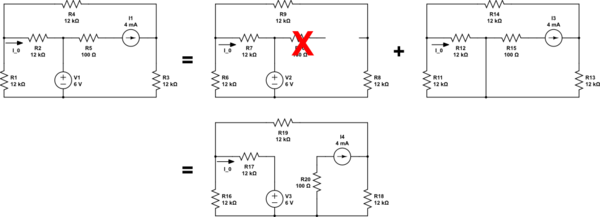
In your original question, you were interested in finding current \$I_0\$. So, my method was perfectly valid. However, if you were interested in knowing the current flowing from voltage source V1 then you would have to replicate the current source like this (I3 in the red box): -

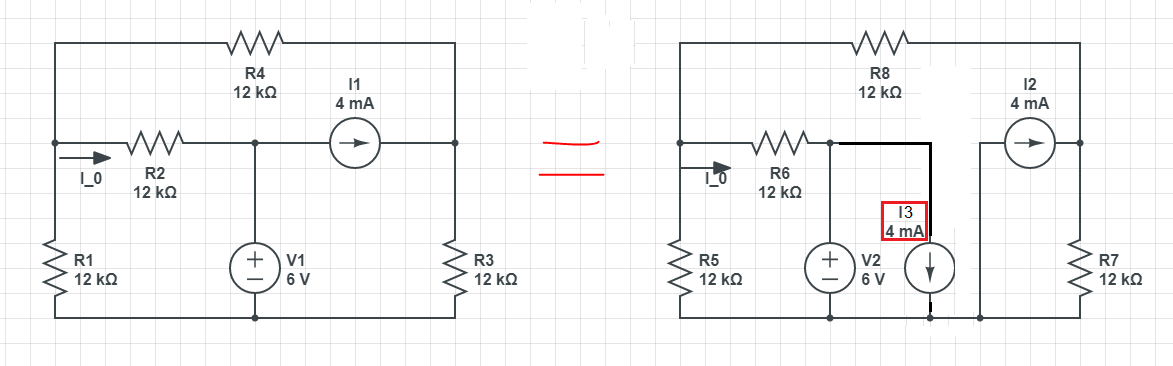
_{The above would be the "true" equivalent circuit.}

I3 (4 mA) is now correctly drawn from V1 but, it did not affect \$I_0\$ and so, in the original problem, I didn't bother to include it as you were only solving for \$I_0\$.

Regards your second question, if you were interested in the power dissipated in R10 then you have to keep it in series with the current source and, if the current source moves (to make analysis easier) then the added resistor moves too.

However, if you do introduce a new current source (as per above), you don't need to introduce a new extra resistor (a la R10) in series with current source I3 (placed across V1). There can only be one R10 even if you duplicate the current source. A resistor in series with a current source does not affect that current.