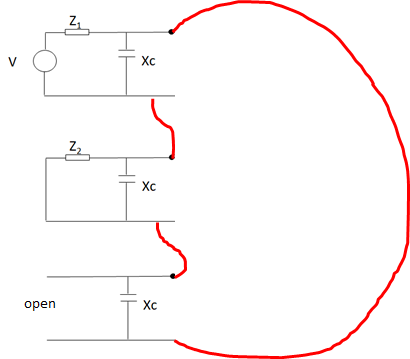
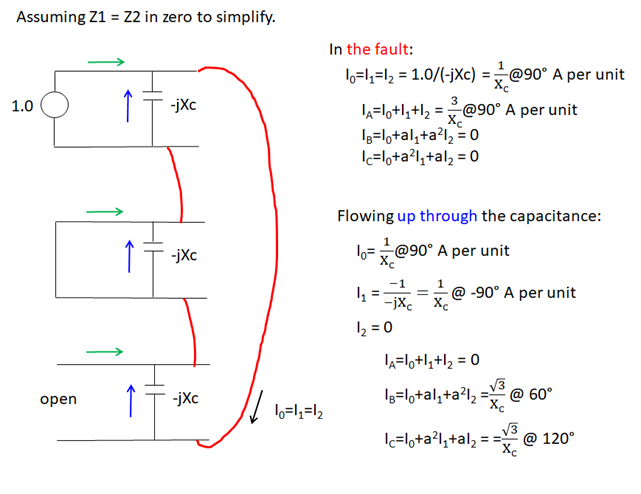
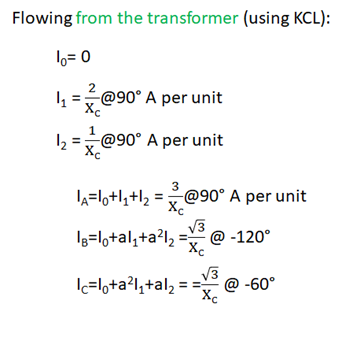
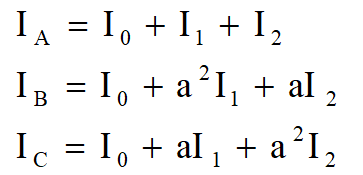The circuit that describes a single line to ground fault in a system with an ungrounded neutral is:
I neglect capacitance between phases and only look at capacitance to ground. The definition of zero sequence current is $$I^{(0)} = \frac{1}{3}(I_1 + I_2 + I_3)$$ But if apllied to the circuit above, Kirchoff current law should state that:$$(I_1 + I_2 + I_3) = 0$$ So how can there be a zero sequence current in this circuit? What am I missing here?
The parasitic phase-ground capacitance is a load in all three sequence networks. Even though your zero sequence source impedance is infinite (delta), the capacitance provides a path for sequence current to flow. In the figure below i show the 3 sequence networks for your system. The red lines show how they are interconnected for an A-phase to ground fault. This should help.
If you calculate the B & C phase currents in the parasitic capacitance (from the sequence currents you calculate with the above circuit) you will see the phase current path clearer.
UPDATE: Added further explanation on calculating the sequence components and phase currents in the fault, in the ph-ground capacitance, and from the transformer. Note the reference arrows i chose (if you use opposite direction just flip phase angle by 180):
