Question regarding the working of slightly different oscillator topologies

Question

I tried simulating two slightly different versions of the Colpitts oscillator in LTspice.

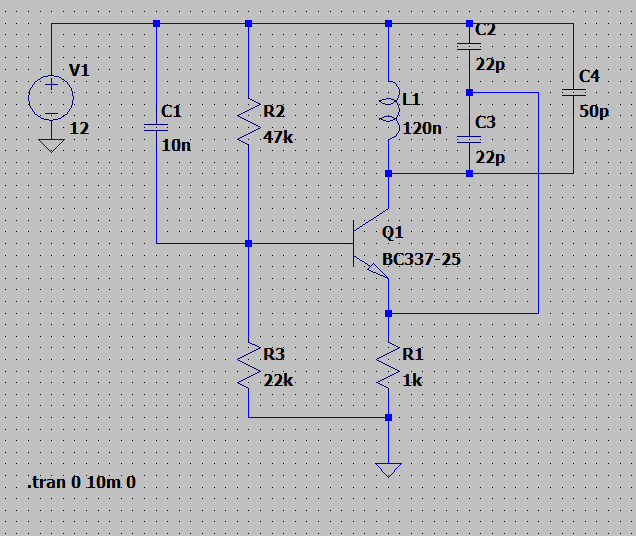
Here is the first one:

This circuit oscillates at 53.8Mhz. It is quite easy to understand how this circuit oscillates just by looking at it.

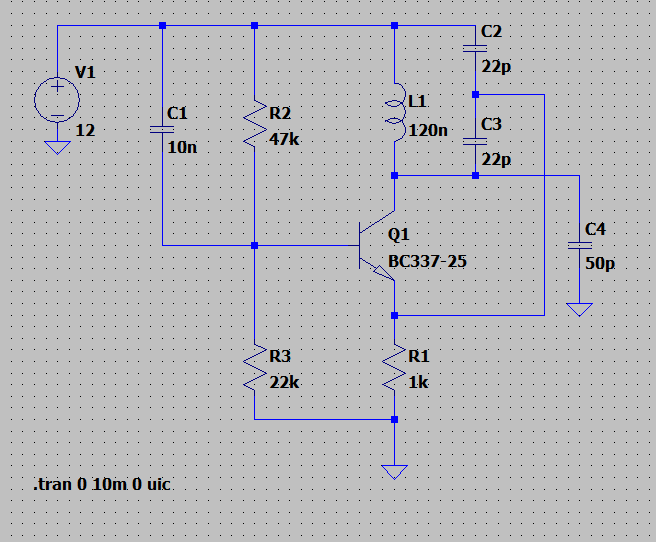
Then I tried grounding the main resonant capacitor, C4 from the collector, a commonly seen method used in self quenching super-regenerative receivers.

Initially the circuit did not oscillate, but in reality they do in the above-mentioned receivers. When I selected the option "skip initial operating point solution" from the simulation menu, the circuit fired up to life and began oscillating at 52.3Mhz.

Now, my question is how does the above version of the Colpitts oscillator work?

My thought is that there is no a visible LC resonant loop, apparently. Also, C4 easily shunts any oscillations to ground there by not startup any oscillations.

Answer 1

Regarding what you said about the first circuit: -

This circuit oscillates at 53.8Mhz. It is quite easy to understand how this circuit oscillates just by looking at it.

For the 2nd circuit you said this: -

Now, my question is how does the above version of the Colpitts oscillator work?

Given that you know how the first one works the only thing that needs to be explained about the 2nd one is that the collector capacitor, is still connected to Vcc regarding AC signals so, there is no difference in how they work. In other words, the DC source (V1) has zero AC (and DC) impedance and C4 is still effectively in parallel with inductor L1.