Control system -- closed-loop system problem

Question

Given a closed-loop system as shown below:

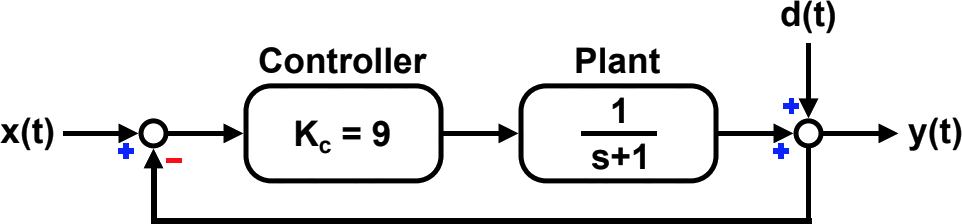
Suppose that \$x(t) = u(t)\$ and \$d(t)=0.5u(t)\$. Determine the output response of \$y(t)\$ and it's steady state at \$t\rightarrow \infty\$.

I've tried to solve the problem but I'm not sure about my answer since my professor only gave us a brief introduction to this material. Here's my attempt:

I know that \$Y(s)=\mathcal{L}\{y(t)\}=\frac{1}{s}\$ and \$D(s)=\mathcal{L}\{d(t)\}=\frac{1}{2s}\$, then

\$ \begin{aligned} Y(s)&= P\cdot C \cdot \left(X(s)-Y(s)\right) + D(s)\\ Y(s)\left(1-\frac{9}{s+1}\right)&=\frac{9}{s(s+1)}+\frac{1}{2s}\\ \frac{s+10}{s+1}Y(s)&=\frac{9}{s(s+1)}+\frac{1}{2s}\\ Y(s)&=\frac{s^2+19s}{2s^2(s+10)} \end{aligned} \$

Using inverse Laplace transform

\$ \begin{aligned} y(t)&=\mathcal{L}^{-1}\{Y(s)\}\\ &=\frac{19u(t)-9e^{-10t}}{20} \end{aligned} \$

For the steady-state value, I used the final value theorem

\$ \begin{aligned} \lim_{t \rightarrow \infty} y(t) &= \lim_{s \rightarrow 0} sY(s)\\ &=\lim_{s \rightarrow 0} s\frac{s^2+19s}{2s^2(s+10)}\\ &=\frac{19}{20} \end{aligned} \$

Any mistake?