I'm having a hard time with the maximum power disposable from a generator derivation, it's something that I should know but I'm confused about it.
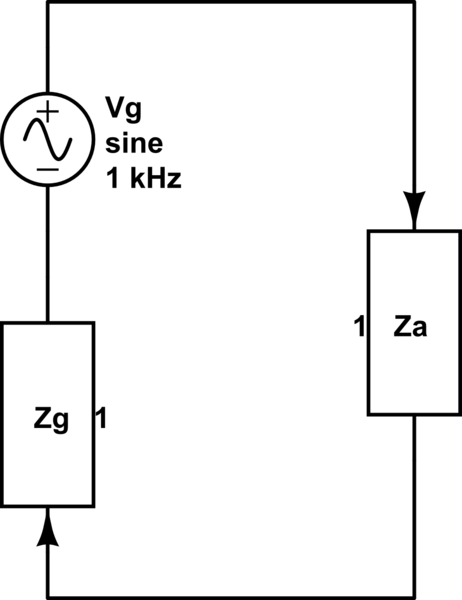
simulate this circuit – Schematic created using CircuitLab
My Professor started with this circuit and the complex power $$W_g= \frac{V_g I_g^*}{2}$$ Where \$V_g\$ and \$I_g\$ are phasors, he then said \$I_g=\frac{V_g}{Z_g+Z_a}\$, then we hypotize the MPT condition (Maximum Power Transfer) so \$Z_g=Z_a^*\$.
Now we have $$I_g=\frac{V_g}{2R_g}=\frac{V_g}{2R_a}$$
At this point we can substitute the Ig in the formula of power obtaining: $$W_g= \frac{V_g I_g^*}{2}= \frac{|V_g|^2}{8R_g}$$ Ok so the result is correct but the mathematical passage is not clear to me, because if you substitute the current there is a 2 factor that doesn't show in the math.
Edit with solution
The problem was stupid because i named Vg over the resistor and the Vg of the voltage generator the same, I had to use the voltage partition formula over \$ R_g\$ to get the \$V_{g_{resistor}}\$ and then multiply it by the current. in formulas: we have (MPT) $$I_g=\frac{V_g}{2R_g}=\frac{V_g}{2R_a} $$ $$V_{g_{resistor}} =\frac{R_g}{R_g+R_a}V_g = \frac{V_g}{2}$$ $$W_g= \frac{V_{g_{resistor}} I_g^*}{2}=\frac{V_{g} I_g^*}{4}=\frac{|V_g|^2}{8R_g}$$
