I'm tried to find the solution, but I failed.
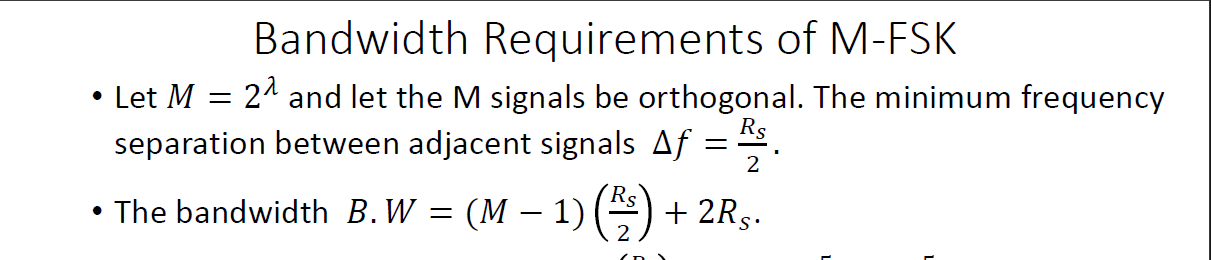
my question in the figure below, and the correct answer it's shown at the end of Figure.
I'm using this formula but the answer is not correct!
Is there another solution to this question?
I'm tried to find the solution, but I failed.
my question in the figure below, and the correct answer it's shown at the end of Figure.
I'm using this formula but the answer is not correct!

Is there another solution to this question?
I think there's not much right here in the question, the slides, and your answer, so that's not your fault. The author confuses "\$M\$-tone Frequency Shift Keying" (M-FSK) with "Minimum Frequency Shift Keying" (MSK). These are two different things.
In all literature I could find¹, MSK is defined as a 2-FSK with a \$\Delta f= \frac{R_s}{2}\$ spacing. However, the slides apply the properties only achievable through that to FSKs with more than two frequencies.
That doesn't work that way; the orthogonality can't be maintained at this spacing for \$M>2\$, nor is the bandwidth formula generally correct even for the \$M=2\$ case (see: the difference between "pure" minimum shift keying (MSK) and Gaussian minimum shift keying (GMSK).
So, as sad as that is, your question "what's wrong about my answer and right about the test's answer" is impossible to answer correctly, because the premises of that test are wrong. :(
¹ that would be