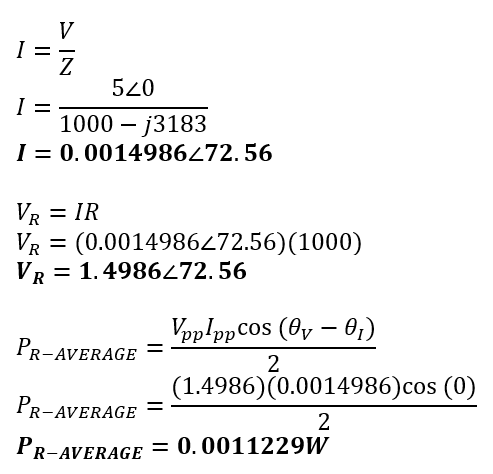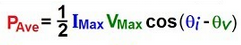0
\$\begingroup\$

So I tried to solve a simple circuit's average power at its resistor, but it seems the formula I used was wrong (?).

Circuit in question...

First thing I did was solve for the current passing through the load, given that this was in a series configuration. Then I solved for the voltage for R1, and then used the conventional average power formula:

Power formula

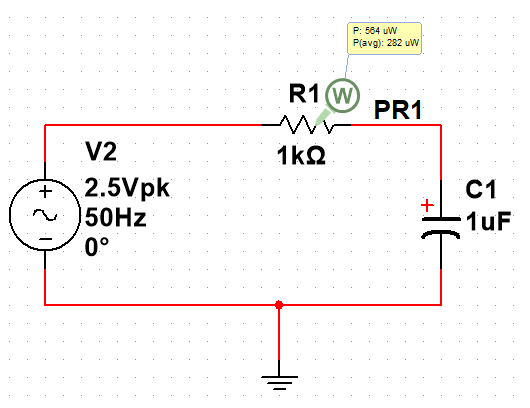
I got about 1.12mW across the 1kΩ resistor. Measured everything in Multisim, from the voltage to the current, and got them right. However, when I tried determining the wattage across the load, it was double what I got.

Actual power was 2.82mW

That led me to think if the 1/2 in the average power formula is required or not, because it seems that doubling it gets what the simulated values show. Perhaps I missed a theory or two? The internet gives me the same formula that I used, though, so I was wondering if anyone could tell me what was wrong with my notion.

Conventional average power formula

\$\endgroup\$

1 Answer 1

Reset to default
1
\$\begingroup\$

The RMS current through the resistor is: -

$$\dfrac{V_{RMS}}{Z} = \dfrac{2.5}{\sqrt2}\cdot \dfrac{1}{\sqrt{1000^2+3183^2}} = 529.8 \text{ }\mu A$$

Therefore, the power in the resistor is current squared x 1000 = 280.7 μW.

I see that your simulation tool calculated the average power as 282 μW

\$\endgroup\$
2
  • \$\begingroup\$ Ah, I see! You used the RMS version. I was wondering, though, if there was anything wrong with the average power formula using the Imax and Vmax values? They send me to the wrong answer, compared to yours. Plus the book I'm using (Alexander & Sadiku's Fundamentals of Electric Circuits) also uses Imax and Vmax, meaning their calculations would also be wrong when attaining average power \$\endgroup\$
    – enrico sebastian
    Commented Sep 27, 2021 at 10:15
  • 1
    \$\begingroup\$ @iheartchococake whenever calculating power I convert current or voltage to RMS values asap. But, as you can see in the top equation, I used "2.5" and that is the peak voltage but, I divided it by sqrt2 to convert the sinewave peak voltage to an RMS voltage. You used "5" i.e. 5 volts p-p when calculating current and that produced a p-p current that is 2.2824 times higher than the RMS current. \$\endgroup\$
    – Andy aka
    Commented Sep 27, 2021 at 10:31

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.