SITUATION:
Nodal Analysis is nothing more than applying KCL and is very powerful. I'm working on developing a more clearer perspective of two approaches in Nodal Analysis "GENERAL & FORMAT". Mesh Analysis is also quite powerful but my questions below are pertaining to Nodal Analysis.
The "General Approach" seems to be more extensive when it comes to factoring and simplifying equations into a form to later solve using simultaneous calculation. After studying the "Format Approach" I started to see a more clearer picture of what my equations were actually representing after the factoring and simplification steps. It was showing that the voltage at the node I was applying KCL too, was simply being multiplied by the sum of the conductance attached to that node.
QUESTION #1: Among the many methods used in solving for unknown currents and unknown voltages would I be theoretically correct in saying that the "Format Approach" is effective in most cases? If not could you explain?
EXAMPLE #1:
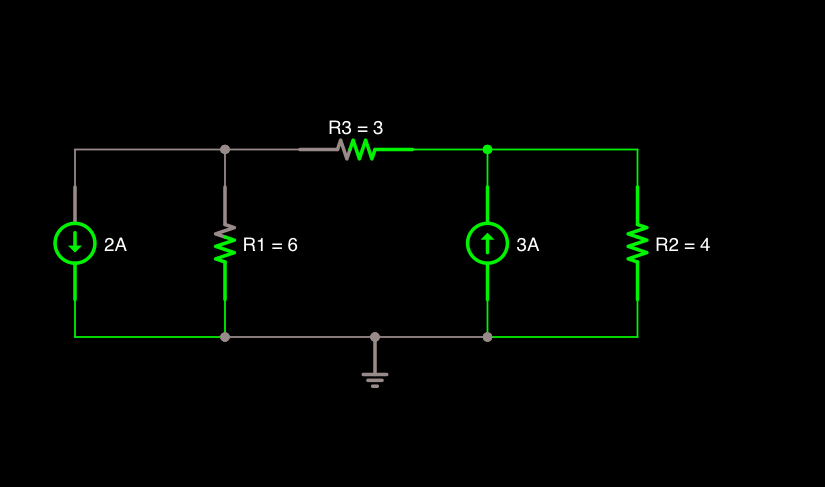
I'm including an example here from my textbook to show the "Format Approach".

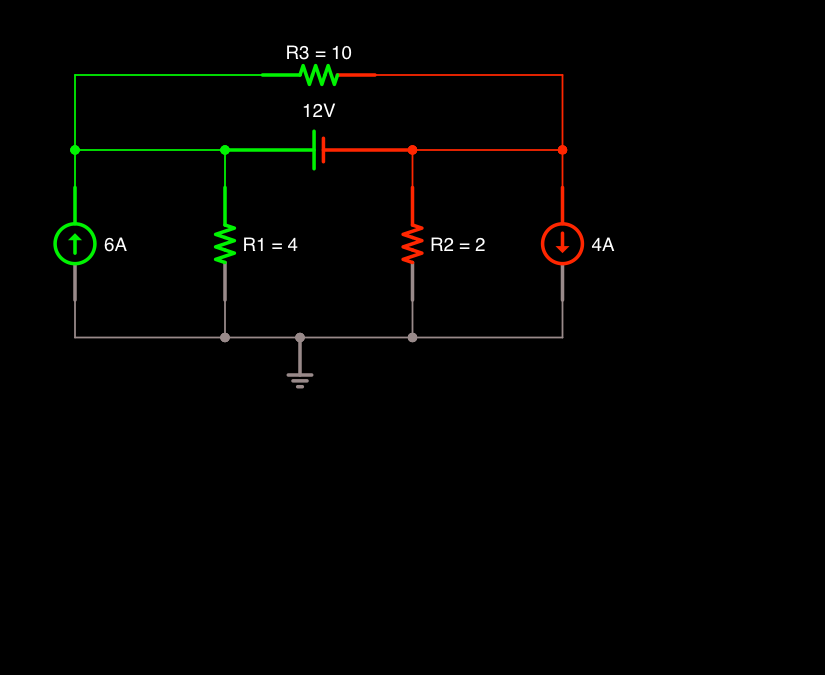
QUESTION #2: How do I write the equation, "in the same format as the first example", that is used to solve the circuit below? Since the circuit below has 2 components that are shared, "12V Source & 10 ohm Resistor", I just can't figure out how to write the equation using the same approach as above.