Using mesh current method find current and power in 15 Ω and 3 Ω resistor branches of the circuit

Question

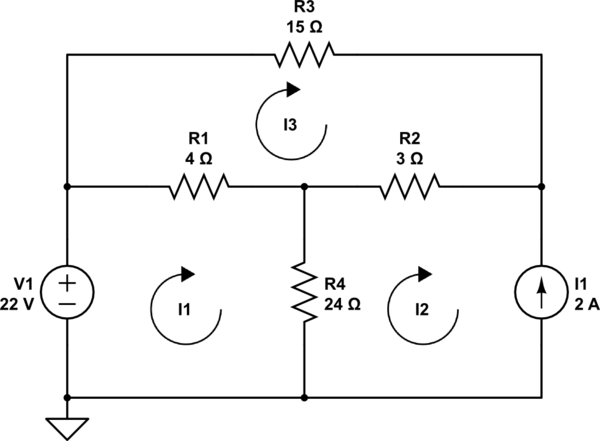
I assumed i1 in the left-most mesh, i2 in the right-most mesh and i3 in the top mesh, all clockwise.

i2=-2 A from the circuit

The equations I got were: from left mesh: 11 - 14 i1 + 12 i2 + 2 i3 = 0 which becomes -14 i1 + 2 i3 - 13 = 0 after putting value of i2

Equation from top mesh: 8 i3 + 3 i2 + 4 i1 = 0 which becomes 4 i3 - 3 + 2 i1 = 0 after putting value of i2

On solving this I got i3 = 1.134 A and i1 = -1.536 A

So current through 15 ohm resistor should be i3 = 1.134 A and current through 3 ohm resistor is coming out to be i2-i3 = -2-1.134 = -3.134 A

But on putting the values in the circuit simulation, I'm getting current through 15 ohm = 453.3 mA
and current through 3 ohm = 1.5467 A

Can someone help me out and tell me what the error is? Have I taken wrong equation, made mistake in computing the values or is my simulation wrong?

Answer 1

Here's the circuit with the mesh currents drawn as you described them, all clockwise:

^{simulate this circuit – Schematic created using CircuitLab}

These are the correct equations applying KVL with mesh currents:

Bottom left mesh:

$$ 24 (I_1 - I_2) + 4 (I_1 - I_3) - 22 = 0 $$

Top Mesh:

$$ 15 \cdot I_3 + 4 (I_3 - I_1) + 3 (I_3 - I_2) = 0 $$

Some points about how I derived these equations, with likely reasons that yours disagree with mine.

1. I tend to traverse each element in the direction opposing current, which allows me to add potentials across resistors. This is because the higher potential side of a resistor is the side where current enters. By traversing a resistor in the direction opposing current flow, I encounter a rise in potential, and I add. When traversing a resistance in the direction of current flow, I encounter a drop in potential, and I subtract. Applying this principle to the bottom left mesh:

   • R4: Moving from the bottom node of R4 upwards, I am travelling against \$I_1\$, and the potential change I encounter due to \$I_1\$ will be positive. However, I am traversing R4 in the same direction as \$I_2\$, so any contribution to change in potential due to \$I_2\$ will be negative. Therefore, the net potential change I encounter as I traverse R4 is: $$R_4(I_1-I_2)$$
   • R1: Moving left across R1, I encounter a positive change in potential due to \$I_1\$, but a drop in potential due to \$I_3\$ since I am traversing in a direction with \$I_3\$. The net potential change is: $$R_1(I_1 - I_3)$$
   • V1: As I cross downwards (still counter-clockwise) over V1 I encounter a drop in potential of 22V, which entails a subtraction.

   Adding all these terms, and assuming I must have traversed a total potential difference of zero (that's Kirchhoff's Voltage Law), we get: $$R_4(I_1-I_2) + R_1(I_1 - I_3) + (-22) = 0$$

2. When I substitute the known value for \$I_2\$, I must use \$I_2 = –2A\$ because my clockwise designation for \$I_2\$ opposes the direction of current source. It would have been better, perhaps, to choose mesh current direction to be consistent with the actual current source in that loop, to avoid confusion.

Answer 2

You can do a star->delta transformation to simplify first. Then try again by resolving parallel resistors etc. should become straightforward this way ...