Connection of Cx capacitors to neutral in 3-Phase EMI filter

Question

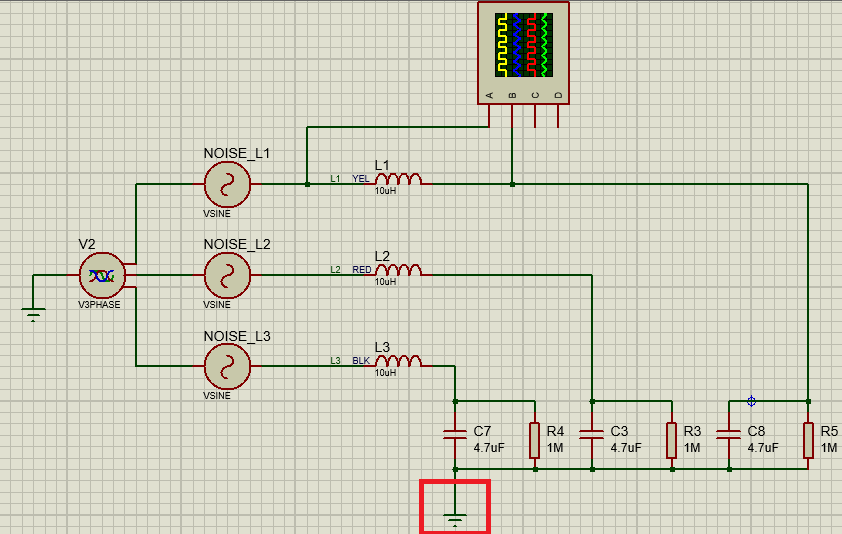
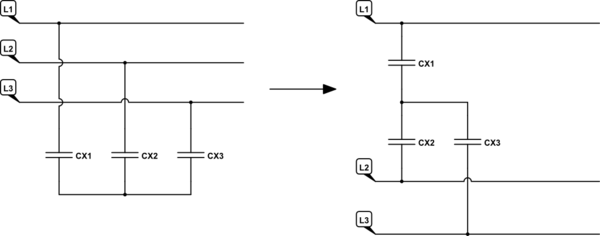
I'm working with a circuit which uses a common schematic for EMI filtering. I simulated the schematic and I added a 200 kHz AC signal to the main 50 Hz line, to model the differential mode noise.

In most of EMI filtering topologies, Cx capacitor are somehow connected to the neutral:

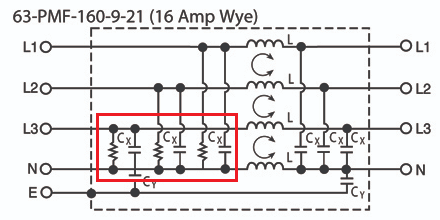
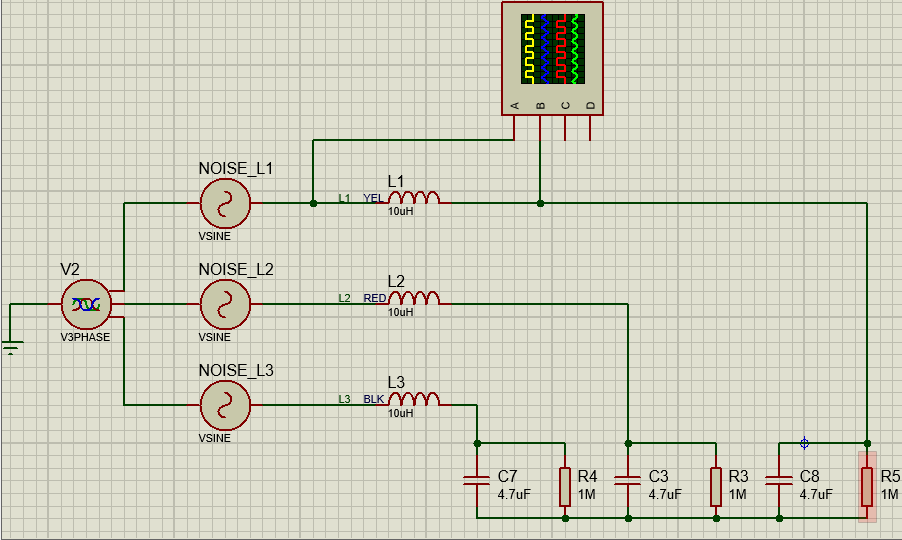
Also there are some topologies (maybe IT systems), in which there is no connection between CX capacitors and the neutral:

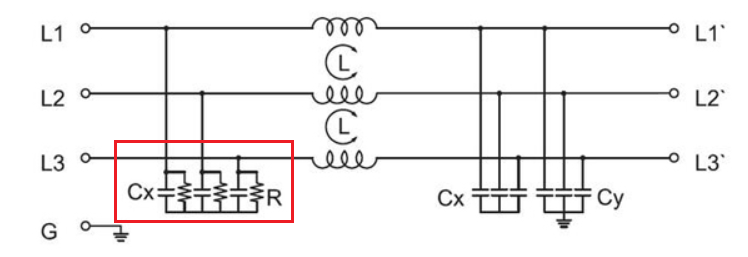
My question is how this CX capacitors filter the differential noise without having path to the neutral?

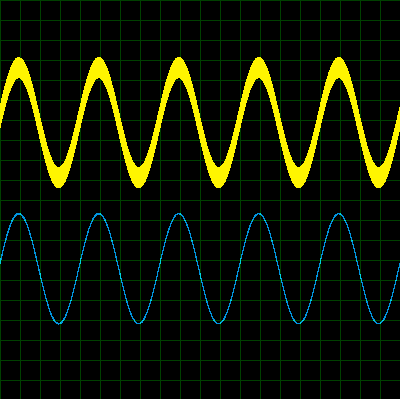
As you can see, from my simulation result, the 200 kHz noise which I added to the main signal, is filtered by the LC filter (only one line is displayed)

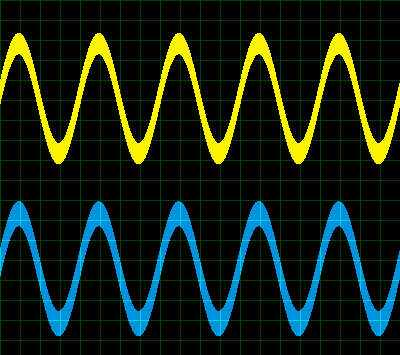
But when I remove the connection of Cx capacitors to the Neutral, no low pass filtering occurs:

Thanks

Answer 1

Referencing to N is only required for Common-Mode filtering. The Cx capacitors are not providing any Common-Mode filtering, they are creating a 2 stage Differential-Mode filter.

Cy are providing the single stage Common-Mode filtering by providing a "low impedance" circulatory path back to the LOAD, for any CM noise that may have circulate outside the unit

Answer 2

Let's talk about this one first:

If you re-arrange the drawing you can see the purpose:

^{simulate this circuit – Schematic created using CircuitLab}

CX capacitors' purpose is to provide filtering for "differential mode noise". Normally, the NEUTRAL is a current carrying conductor. So a filter for differential mode noise can be placed across each phase and neutral. CY capacitors (i.e. the ones having their one end connected to EARTH) are for common-mode noise filtering because common mode noise exists on each phase conductor and is EARTH-referenced. Remember that ground conductor is assumed to carry the fault current only, not the load current.

Now, looking at this one:

It's obvious that the CX capacitors are for differential mode noise. However, CY capacitors are only placed across L3-E and N-E. This might be an indication of that this product can be used for a single phase system (using only L3, N and E).