Difficulties in modelling magnetic levitation system?

Question

I am currently working on and writing a project (not homework) surrounding magnetic levitation with an electromagnet having to levitate an object underneath itself. I have taken a look at relevant papers, examined their calculations and have basically taken "inspiration" from their works to apply to my calculations, such as the modelling of the mechanical and electrical parts of my system.

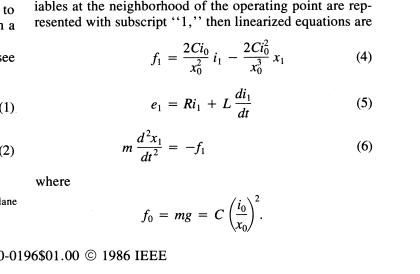
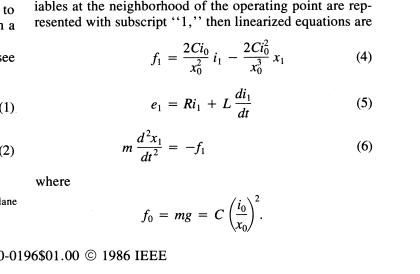
To simplify things, this modelling first required creating an equation of the electro-mechanical forces being applied, which would lead to a second order equation of motion, being \$mx" = mg - f(x, i)\$

where:

• x" is basically the acceleration already in second order.
• mg is the weight of the object being attracted(suspended) by the electromagnet.
• f(x, i) is the force of the electromagnet represented as a function of x and i, where x is the distance, and i is the current.

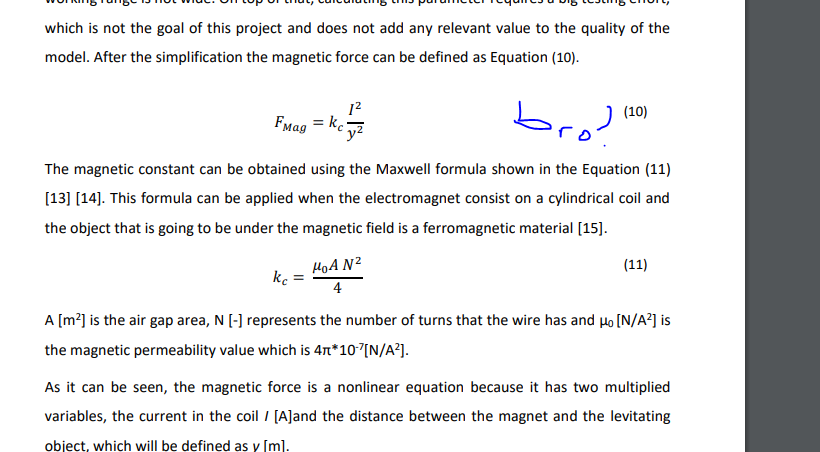
F(x, i) would be expanded through the use of Faraday's energy in inductor equation, but to not go off on an unrelated tangent, this is what you get, basically the f0 and f1. [0].

[0]: Taken from T.H. Wong's paper: Design of a Magnetic Levitation Control System???An Undergraduate Project.

Link: https://ieeexplore.ieee.org/document/5570565

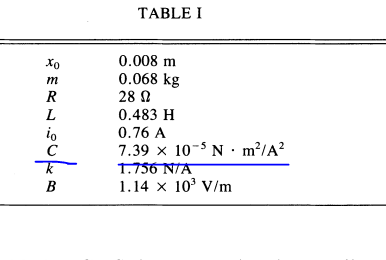
However, midway through calculations, I noticed that I could not obtain one of the values, being the so called magnetic constant C, yet all the authors somehow come up with random values assigned to this "constant" that was more or less left undefined.

This constant, \$C = \frac {L_0 x_0} {2}\$ which is calculated from doing a derivative as shown below 2 has its value already obtained, being given as a Newton-Meter^2/Area squared 3, which is somewhat consistent with the other papers.

2: Taken from T.H. Wong's paper: Design of a Magnetic Levitation Control System???An Undergraduate Project.

Link: https://ieeexplore.ieee.org/document/5570565

3: Taken from T.H. Wong's paper: Design of a Magnetic Levitation Control System???An Undergraduate Project.

Link: https://ieeexplore.ieee.org/document/5570565

4: Taken from Juan Sebastián Lafuente Larrañaga's paper: Design and Development of a Digitally Controlled Magnetic Levitation System

Link: https://addi.ehu.es/bitstream/handle/10810/36694/MasterThesis.pdf?sequence=1&isAllowed=y

But I do not understand how they even assigned it to them. Just when I had the calculations almost complete, this came out of the left field and just confused me completely. What am I supposed to do? How do I determine C's value? I apologise I cannot provide calculations because I seriously do not know what to calculate, lol, I have the model almost down, but I cannot complete it if I do not have the value for C, and I don't know how to obtain that. I may have missed something, surely, but I do not know what.

Bibliography (Other papers which basically do the same thing):

1. https://ieeexplore.ieee.org/document/5570565
2. https://addi.ehu.es/bitstream/handle/10810/36694/MasterThesis.pdf?sequence=1&isAllowed=y
3. https://ieeexplore.ieee.org/document/8986710
4. https://www.sciencedirect.com/science/article/pii/S2405896316301513
5. https://www.slideshare.net/SalimAlOufi/mse6183finalprojectwriteupslso05052015rev2#:%7E:text=This%20project%20is%20about%20controlling%20the%20position%20of,feedback%20of%20the%20position%20of%20the%20magnetic%20ball.?msclkid=ed73953da6fd11eca322287678e98ddb

Answer 1

So after brute forcing this problem, I finally managed to figure out what the issue was.

Each author of the paper basically have their own experimental calculations for determining the inductance change over distance with a ferromagnetic object, some albeit being the same. This, when combined with the Faraday inductance energy stored, creates an equation of the electromagnet's force in respect to distance and current. 1

Figure 1. Result of the calculation of Electromagnetic force taken from T.H Wong's paper. Link: https://ieeexplore.ieee.org/document/5570565

You basically get the attached result when combining the inductive equation for energy, inductance depending on distance and application of the Taylor Series. Since the system is not linear, Taylor's Series had to be applied as you can see the image attached.

An assumption had to be made(like other papers) that the $$C = \frac {i^2} {x^2}$$ was the force required to first lift the electromagnet, along with what you see as f1 being the linear dynamics required to keep the levitated object stationary. What I did not realise was that even though I assumed that it was the initial force to counteract the weight of the ball dropping, that it was basically equal to mg and cancelled out mg. Therefore, you could just equate the two and solve for C 🤦‍♂️ .

When I solved for C, I ended up getting $$C=mg\frac{x_0^2}{i_0^2}$$ I tested this calculation on one of the linked papers above and well it worked, I got the value of C. It was funny too because some of the other papers had this same equation flipped or some other random equation they obtained with no explanation or reference, hence my total confusion earlier. I also had realised that I got my units totally wrong, that it was Newton meter^2/Ampere^2. After obtaining that, all I had to do was plug in my object's mass and the current being used, and that was basically it.