I have been learning about LC and LCR circuits.
My question is about inductors themselves, more specifically ideal inductors with zero resistance.
If I disconnect an ideal inductor from a DC supply, there should be very high voltage spike across the inductor as per \$V=L\frac{dI}{dt}\$.
How can this be? The two ends of the inductor are connected via zero resistance through the coil of the inductor itself and therefore wouldn't the voltage across the two ends of the inductor always be zero?
The two ends of the inductor are connected via zero resistance through the coil of the inductor itself and therefore wouldn't the voltage across the two ends of the inductor always be zero?
You are assuming that an inductor obeys Ohm's law. It doesn't. Ohm's law is a law for resistors. An [ideal] inductor is not a resistor, therefore it doesn't obey Ohm's law (and neither does a voltage source, a capacitor, a diode, a transformer, or any other device that isn't a resistor).
The "law" that governs the operation of an inductor is
$$V = L\frac{dI}{dt}.$$
You need to analyze the operation of an inductor using this law rather than Ohm's law. And this law tells you that in order for the switch to open instantly, there must be an infinite (delta function) voltage impulse across the inductor.
This is a case that sometimes two "ideal" components in a circuit produces a logical contradiction, like two ideal voltage sources in parallel, or a switch connecting two ideal capacitors.
In this case you can't assume that both the inductor the switch are ideal and get a physically meaningful result in your analysis. You must consider either the arcing behavior of the switch, or the interwinding capacitance of the inductor (or both) to correctly model the circuit without singularities.
How can this be? The two ends of the inductor are connected via zero resistance through the coil of the inductor itself and therefore wouldn't the voltage across the two ends of the inductor always be zero?
An ideal inductor has zero resistance, but it has inductance.
there should be very high voltage spike across the inductor as per \$V=L\frac{dI}{dt}\$.
In a mathematical model of a circuit, inductance is defined by that equation. If you are analyzing a circuit that contains an inductor, you simply accept that that is how an inductor behaves.
Of course, there is a physical explanation of why a coil of wire exhibits inductance—of why the behavior of the coil is approximately described by that equation—but understanding it will take you deep into physics and math.
(see Wikipedia articles on Faraday's Law, and Lenz's Law, and on inductors in general.)
That's deeper than I can go, but the TLDR version is: When current flows in any conductor, the conductor is surrounded by a magnetic field. (The coils of conducting wire, and the ferromagnetic core in a practical inductor serve to concentrate that field.)
Anyway, that magnetic field stores energy. It takes energy to create the field (i.e., to ramp up the current), and the inductor returns that energy to the circuit when the field collapses (current ramping back down.) Those changes in energy are manifested as voltage that opposes the changing current, as described by the equation that you cited.
As long as there is variation in current, there will be a voltage \$V=L\frac{dI}{dt}\$ across an ideal inductor.
I think your misconseption is in "where" the "resistor" is in a non-ideal inductor: it has to be modelled in SERIES with the ideal inductor:
simulate this circuit – Schematic created using CircuitLab
So if the internal resistor (r) tends toward 0 (i.e. ideal inductor), then it is NOT shorting the terminals of the ideal inductor.
Now, why can we model the inductor as an ideal inductor as one end and a resistor at the other end?
Let's suppose we cut the inductor into N small (identical) inductors with resistance r/N, and inductance L/N, we get:
As the same current passing through each component is the same, you can reoder the components while keeping the same voltage between A and B.
Now, as resistors add in series, the total resistance is Rtot=N*(r/N)=r. Similarly, inductors also add in series, so Ltot=N*(L/N)=L.
EDIT: with your new comment, about the specif case of a DC circuit with only ideal inductor and switch.
If there is no internal resistor neither in the inductor, nor in the wires, nor in the supply, nor in the switch (ie all components are ideal), then you get a short circuit when the switch is closed. It means your voltage source provides infinite current (and therefore power).
Now let’s supose the voltage source somehow limits current (for example with an internal resistance). We get some current accross the inductor. When we open the switch:
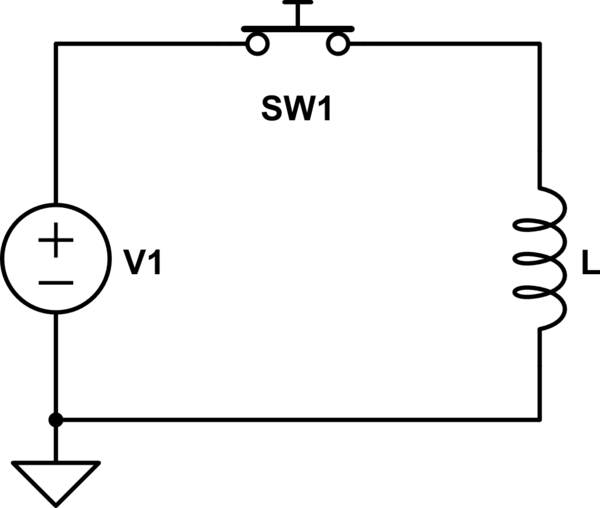
Often, for practical purposes, we do not draw a distinction between a voltage drop through a component and an electromotive force generated through that component. Both are measured in volts. However, sometimes the distinction needs to be drawn in order to avoid running into apparent paradoxes.
The voltage drop through a component is the line integral of the electric field through that component.
$$U = \int_a^b \vec{E} \cdot d\vec{s}$$
The emf generated by an inductor is the line integral of the rotational component of the electric field through that component.
$$\mathscr{E} = \int_a^b \vec{E}_{rot} \cdot d\vec{s}$$
According to Kirchhoff's Voltage Law, around any loop, the sum of the EMFs through the components in the loop equals the sum of the voltage drops through the components of the loop.
$$\sum_i \mathscr{E}_i = \sum_i U_i$$
Or in Kirchhoff's original presentation
$$\sum_i \mathscr{E}_i = \sum_i I_iR_i$$
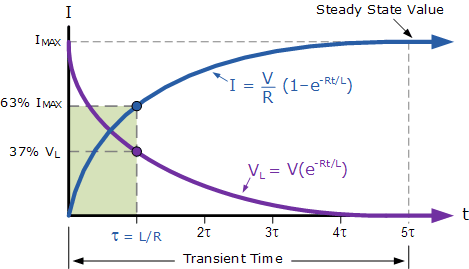
where \$I_i\$ is the current through a component, and \$R_i\$ is the resistance offered to that current by that component.
You are correct that the voltage drop, as defined above, through an inductor depends upon the resistance of the wire and the current through it.
The two ends of the inductor are connected via zero resistance through the coil of the inductor itself and therefore wouldn't the voltage across the two ends of the inductor always be zero?
For an ideal inductor, where the resistance is 0, the voltage drop across it will be 0. However, the EMF generated by an inductor will result in a voltage drop across resistances that are connected to the inductor in a circuit loop. Thus, if you connect a volt-meter across the terminals of an inductor (experiencing changing current), a voltage drop will be present across the volt-meter, and hence the volt-meter will give a reading proportional to the EMF generated by the inductor, rather than the voltage drop through the inductor. In a sense, the EMF causes a voltage drop elsewhere in the circuit.
The two ends of an ideal inductor are connected by a zero resistance wire. However, to make this an inductor, this wire encloses a loop of some area.
When a current flows round this loop of wire, a magnetic field is set up in the area defined by loop.
When the magnetic field in the area of the loop is changed, then a voltage is generated round the loop. This voltage appears across the ends of the wire.
We observe this to be so, experimentally. Why it happens depends on your definition of 'why'. You could explain it in terms of other deeper theories, quantum electro dynamics (QED) for instance is probably the deepest, or a little higher using Maxwell's equations. However, ultimately, you could say 'why' to these theories as well. Don't forget that both of these theories were developed to summarise the experimental results we observe.
To answer your question of 'How can this be that the two ends of a zero resistance wire generate a voltage between them when the current changes?' It happens. That's the observed reality. Deal with it.
There are two ways to view this: an inductor with resistance obeys the equation \$U = R\cdot I + L\cdot\frac{\mathrm d\,I}{\mathrm d\,t}\$. With zero resistance, the left term is zero, but the right term is still there.
The other way to look at it is by considering Kirchhoff's equations that state the total voltage in each loop of a circuit is zero: the amended version is that the total is not zero but adds up to the (negative) time derivative of the flux change through the loop. An inductor has a multiplicity of loops around the relevant flux, and it is also responsible for creating the flux in the first place via magnetisation of its core (or of air/vacuum) according to its permeability.
With either of those views, you need to take into account what makes the inductor an inductor (namely tying its current to a significant magnetisation and its voltage to the temporal change of the related significant magnetic flux). When stipulating an ideal inductor without resistance, no voltage is due to material's electric conductivity, but that does not take care of the coupled magnetic fields and the voltages induced by their change.
It does not matter if the inductor is ideal or not. And ideal inductor just has inductance, it does not have any series resistance. A changing magnetic field induces a voltage in any inductor. If current to inductor is suddenly turned off, the rate of change of current di/dt is infinite so induced voltage is also infinine.
(Image source: Electronics Tutorials - Transient Curves for an LR Series Circuit)
Referencing the picture:
As R goes to 0 and time goes to infinity the exponential term approaches 0.
I = V/0 > infinity
VL = V x 0 = 0
No R
DC V, infinite bus
Your system is undefinable.
This thought model will not help you understand L in a circuit.
The time constant is L/R = infinite meaning the time must go to 0, or instantaneous.
Close switch: I goes instantaneously to infinity, V/0
There will be no V across L
Open switch: I goes to 0 instantly
Since I is unbounded it's derivative is also, but we know V = 1, not possible.
The energy is infinite also
E = 1/2 L I^2
