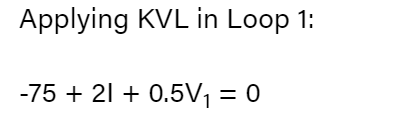Applying KVL in loops

Question

So, this is sort of a basic question but I keep getting this wrong somehow. The Kirchhoff's voltage law says that the source voltage is equal to sum of all voltage drops and mathematically it is written as ΣV - ΣiR = 0. Now, if we apply this in the first loop as shown

We need to consider the polarity of the voltage sources also. Now, current flows from +ve to -ve inside the battery, so if we write the kvl equation w.r.t. the direction of current as shown, what would be the correct answer and why? No matter how I see it, I always get this

-75(because battery polarity) -2kI(because ΣV - ΣiR = 0) +0.5V1(because +ve to -ve) =0

Now, if the current flow reference that we take were to be opposite of this, then the kvl equation (according to me) would be

-0.5V1(because -ve to +ve) +2kI(because ΣV - ΣiR = 0 and I is opposite to our current reference) + 75(because +ve to -ve) = 0

which is the same as above. But, the solution on the site where this question is from shows that the equation will be

What am I doing wrong?

Answer 1

First let me point out that your statement that current flows from positive to negative inside the battery is probably wrong. That would be the case if the battery were being charged.

In the usual situation where a battery is providing energy to the rest of the circuit, the exact opposite is true. Current leaves the battery from the positive terminal, goes around outside and re-enters into the negative terminal. Thus when the battery is being an energy source, inside current flows from its negative terminal to positive.

The second thing to realise is that you don't necessarily know, until you've solved the equations, if the battery is charging or discharging. The thing is, you don't need to, because you do know the voltage across the battery and its polarity.

So, when you apply Kirchhoff's Voltage Law to a loop containing a cell or battery of cells, the voltage you add (or subtract, depending on your direction around the loop) does not depend in any way upon current direction. Its positive end is higher in potential than its negative end regardless of the direction of current through it!

This logic does not apply to resistors, though, and this is perhaps where your confusion comes from. The polarity of voltage across a resistor depends entirely upon the direction of current through it. That's why, when applying KVL to resistances in a loop, you absolutely must account for current direction. For a resistance, the higher potential is always the terminal where current enters.

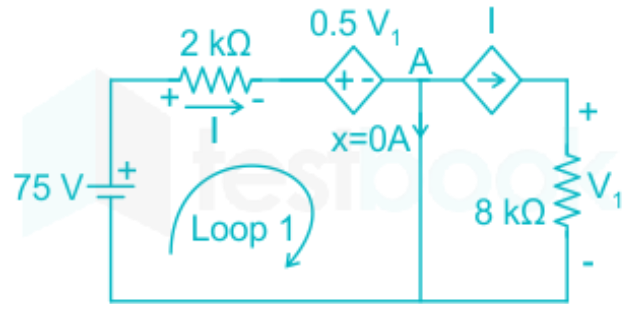
Applying these principles to your circuit, you've started by labelling current direction in loop1. This is essential, because we can't possibly assign polarity of voltage across the 2kΩ resistor without it. We may have labelled current direction incorrectly, but that's OK; the solution will simply have the wrong sign, but the algebra will be correct.

Concerning the battery, though, we don't care which direction current flows, we know simply that the top end is positive, period.

Now take a journey around loop1 starting wherever you want. I'll start at the bottom left node, the battery negative. I'll go clockwise, in the direction that I assume (even guess) current is flowing. First I jump over the battery. That's an increase of 75V, so I add. Then I traverse the 2kΩ resistor, which entails a decrease in potential, because as I said, current in a resistor always flows inside it to the lower potential, so I subtract that potential difference. The last jump will be across the dependent voltage source, where I encounter a drop in potential. Again, I must adhere to the polarity labels; as I cross the source, potential falls as indicated, so I subtract. Because I am back at the node where I started, I must necessarily have returned to the same potential, which is why the sum of all those potential increases and decreases must be zero. The equation I get is:

$$ +75V -(2k\Omega \times I) - 0.5V_1 = 0V $$

If I went anticlockwise around the same loop, starting between the resistor and dependent source, I'd get the same equation, but with terms in a different order, and all signs negated. It's the same expression though (algebraically speaking), because I adhered to the facts I have available regarding polarity, and the predictable behaviour of batteries and resistors:

$$ +(2k\Omega \times I) -75V +0.5V_1 = 0V $$

This is what you should take away here:

1. For resistors, you must label the polarity of voltage across them in accordance with your label for current through them. Current in a resistor flows from high potential to low. If, in the solution, current comes out negative, so will the voltage across the resistor. the maths is still correct, you just guessed the polarity and current direction wrong.

2. For independent voltage sources, polarity is known ahead of time, so apply KVL with no concern for current direction. Current direction will come out in the solution, and only then can you know if the source is supplying or receiving energy.

3. Treat the application of KVL as a journey around a loop, keeping your direction of travel in mind as you go. Add increases of potential that you encounter as you traverse each element, and subtract decreases. This is easy if you've labelled all the polarities, so label everything first, and use what you know about those components to keep your "guessed" voltages consistent with your "guessed" current directions.

Answer 2

In loop 1, the voltage sources 75V and 0.5V1 are in opposition to each other hence the effective voltage source is (75 - 0.5V1). This is your ΣV. Now, the voltage drop "iR" across the 2K ohm resistor is 2kI.

Thus, keeping current direction across 2K ohm resistor in mind KVL equation (ΣV - ΣiR = 0) can be written as follows: equation: (75 - 0.5V1 - 2kI = 0) OR, (-75 + 0.5V1 + 2kI = 0).

Answer 3

In the right hand loop, V1 = 8k * I

and so, in the left hand loop 75 - (2k * I) - (4k * I) = 0 which gives I = 12.5 mA

and from my first equation V1 = 100 V

There will be 100 V across the current source.