0
\$\begingroup\$

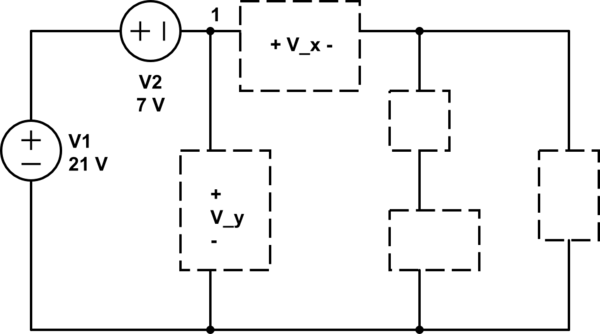
There is a Problem in our textbook which asks us to find, using Kirchoff's law, the voltage across component v_x and v_y.

According

I know that the voltage at node 1 should be 14 volts from [21 + (-7)]=14 (in terms of $$v=\frac{dw}{dq}$$ not in terms just of potential difference.

The voltage across V-x is 9 because the voltage across the two components in series right below it is 5.

Of course, v_y is 14. This said:

Is there going to be any voltage across the rightmost component?

schematic

simulate this circuit – Schematic created using CircuitLab

\$\endgroup\$
1
  • \$\begingroup\$ If you say the voltage at node 1 is 14V, that is most certainly in terms of a potential difference. The implication is that the bottom node in your diagram is ground, which is the assumed point of reference when we speak of a single node having a given voltage. \$\endgroup\$
    – Joe Hass
    Commented Sep 27, 2013 at 17:20

1 Answer 1

Reset to default
3
\$\begingroup\$

I'm going to assume you meant "rightmost" since the leftmost component is a supply.

The voltage across the rightmost component must be the same as any components in parallel. Hence, it has 5V across it.

\$\endgroup\$
1
  • \$\begingroup\$ Ahhhhhh... that absolutely makes sense on the surface of it. So if Applying Kirchoff's voltage law, every node providing a in parallel counts as only one entry departure from the closed surface? \$\endgroup\$
    – user1833028
    Commented Sep 27, 2013 at 5:26

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.