2
\$\begingroup\$

In my Circuit Analysis book, I am tasked with finding \$v_x\$ and \$v_y\$. So \$v_y\$: easy enough. It's - 6 volts. But I encounter a problem when trying to find \$v_x\$ - I can't develop enough independent equations to solve for all of the elements in a given loop.

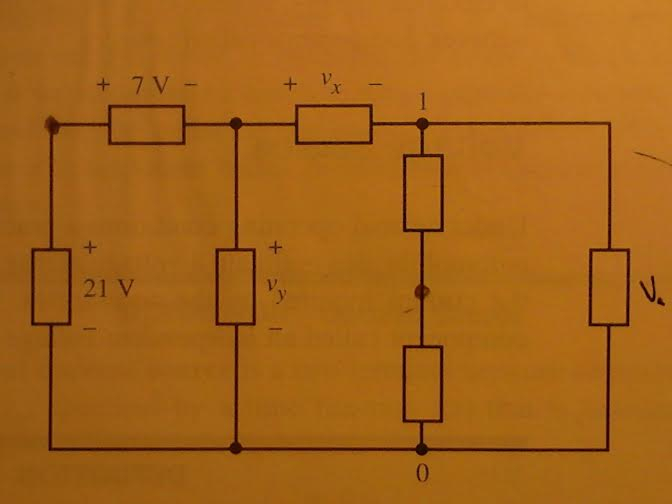
Circuit Diagram

Of the components in series, I called the topmost one \$v_c\$ and the lower one \$v_b\$. I called the rightmost component \$v_a\$.

$$ -21+7+v_x+v_a=0\\ v_x-14+v_c+v_b=0\\ -v_b-v_c+v_a=0\\ -21+7+v_x+v_c+v_b=0\\ $$

Sadly, I can show at least to of these to be the same equation (they are not indepenet of each other!) so I have literally no idea how to solve this.

This is only the second problem of this type I have ever tried to solve, that I remember.

I would really appreciate it if someone could get me out of this jam!

The book gives the answer to be 9 volts.

\$\endgroup\$
4
  • 1
    \$\begingroup\$ Well, there is several notable things about this question. 1) 21-7 != -6 2) these blocks in the diagram serve an unknown function, are they voltage sources? are they resistors? 3) most notably, there is not enough information here to determine \$V_x \$. \$\endgroup\$
    – placeholder
    Commented Sep 15, 2014 at 14:39
  • \$\begingroup\$ Not enough information? Thank god. That's what I thought. If you can prove this, I'll gladly accept it as an answer. \$\endgroup\$
    – user1833028
    Commented Sep 15, 2014 at 15:49
  • 1
    \$\begingroup\$ What do the "1" and "0" mean in the diagram? Are the just node numbers? Without component values or voltages or currents for these three elements on the right they could be anything. For example, if the two in series in the center are 0 ohm resistors then they short out node 1 to node 0. If they tend to very large values then the answers depend on their exact values. There must be some text missing from the figure. (oh, and 21V-7V = 14V...) \$\endgroup\$
    – mixed_signal
    Commented Sep 29, 2014 at 3:22
  • \$\begingroup\$ Could you type in the complete question found in the book? Perhaps the labels $0$ and $1$ refer to a logic $0$ and a logic $1$, where a logic $1$ is defined to be 5 volts? \$\endgroup\$
    – JRN
    Commented Sep 30, 2014 at 1:30

1 Answer 1

Reset to default
1
\$\begingroup\$

According to your image ,Vy is equal to 14 volts. ( 21 - 7 = 14 ) You can't find Vx just by information in this image. The proof is you can write 3 independent equations, but you have 4 independent variables! If you know the voltage of node "1" or node that you marked it, you can find all of the voltages. You should find more information in the book. Otherwise you can't solve this problem. If you want to know that why you can't solve a system of three equations in the four variables you should search the related math books.

\$\endgroup\$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.