How do you calculate the noise of an op-amp circuit?

Question

I think I know how to do this, but you can find a lot of different instructions and calculators online that contradict each other. I have yet to find a clear, concise procedure for calculating the self-noise of op-amp circuits (including thermal noise, shot noise, etc., but not including interference from external sources), and one of the sources many people cite apparently has a number of errors, so I'll ask it here and see who can explain it best.

For example, how would you calculate the output noise of this circuit?

Which noise sources do you include?

• Op-amp internal input voltage noise
• Op-amp internal input current noise
• Resistor thermal noise
• Op-amp output stage noise?

How do you calculate each component's contribution? How do you combine the noise components together? What gain do you use to get the output noise from the input equivalent noise? How do you calculate the gain? Is it the same as the signal gain? What kind of simplifications and shortcuts can be made and how different will the result be from the real world?

etc. etc. etc.

Answer 1

The question which noise sources need to be taken into consideration depends on how severe they are. Your question indicates that you are interested in noise generated at the op amp and not noise generated by interference from neighboring circuits (internal/external noise).

In order to make things comparable, all noise is referred to the op amp's input (RTI). In theory, I guess any point in your circuit might work as long as you refer all noise sources to that point, but it is common practice to act as if all noise sources were directly at the input pins. Sources include noise in the resistors, noise generated by current flowing into the op amp's input pins and noise that may be considered as a voltage between the inputs pins.

There is a very good discussion at this Q&A-style source and also in this nice article from 1969 (!), both authored by Analog Devices' staff.

Without re-typing everything in these sources, here are some rules of thumb:

Noise in the resistors becomes bad when the resistor values are high (some 100k or some 1M) and when the circuits are designed for high bandwidth since the noise is proportional to \$ \sqrt{4k \cdot T \cdot B \cdot R}.\$

You can try to minimize R, you can try to limit the bandwidth B if possible, you can put the circuit in liquid nitrogen (low temperature T), but you can't go for a low Boltzmann constant, because Boltzmann is dead (quote stolen at Analog Devices).

Current noise, i.e. noise generated by current flowing into the op amp inputs, will be converted to a noise voltage by the resistors around the input (\$R_f\$, \$R_g\$) and amplified by the circuit's gain. This is one of the reasons why one prefers op amps with very low input currents especially for high-ohmic circuits.

Voltage noise results from a real op amp's inability to completely null the voltage between the input pins.

All noise sources can be combined as the square root of the sum of their squares since they are independent of each other, which will work only if all sources are RTI.

Answer 2

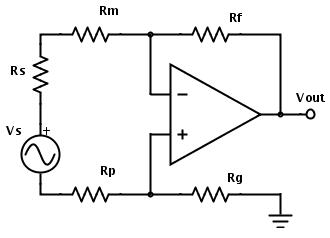
OK, I know how to do this now.

There are 3 main sources of noise that need to be calculated:

• Thermal noise of the resistors themselves
• Voltage noise of the op-amp itself
• Current noise of the op-amp, which interacts with the resistors to produce a voltage noise

So first, you want to find the equivalent resistance seen from the inputs of the op-amp looking outward into the circuit, with voltage sources (such as the op-amp output) set to 0 V (equivalent to converting them to short-circuits to ground). For this circuit: $$ R_\mathrm{eq}=(R_\mathrm{m}+R_\mathrm{s}+R_\mathrm{p})\|(R_\mathrm{f}+R_\mathrm{g}) $$

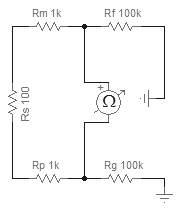
So for example, if Rs = 100 Ω, Rm = Rp = 1 kΩ, and Rf = Rg = 100 kΩ, then Req = 2.1 kΩ.

To find the thermal noise of this equivalent resistance, use the Johnson–Nyquist formula: $$ v_\mathrm{n}={\sqrt {4k_{\text{B}}TR\Delta f}} $$ There are online calculators to do this for you:

• sengpielaudio.com
• Daycounter, Inc.

For example, with Req = 2.1 kΩ, at 27 °C, with an audio bandwidth of 22 kHz, the resistors would contribute 0.87 μV_RMS = −121 dBV input noise.

Then find the voltage and current noise of the op-amp in the datasheet. Typically:

• If \$R_\mathrm{eq}\$ is small, you want a BJT-input op-amp, which has lower voltage noise (0.7-5 nV/√Hz), but higher current noise (500-4000 fA/√Hz).
• If \$R_\mathrm{eq}\$ is large, you want an FET-input op-amp, which has lower current noise (1-10 fA/√Hz), but higher voltage noise (3-15 nV/√Hz).

To convert the spectral density \$\tilde v\$ (in nV/√Hz) to a voltage (in V_RMS), you need to multiply it by the square root of the bandwidth: $$ v_\mathrm{RMS}=\tilde v \cdot \sqrt{\Delta f} $$ So for example, if the op-amp is a TLC071, with equivalent input noise voltage density of 7 nV/√Hz, the voltage noise of the op-amp contributes 7 nV/√Hz ⋅ √(22 kHz) = 1.04 μV_RMS = −120 dBV.

The resistor noise and op-amp noise are similar levels, which means they'll combine to about 3 dB higher, or −117 dBV. To calculate their combination exactly, since they're uncorrelated, you need to use root sum squared: $$ v_\mathrm{total}=\sqrt{{v_\mathrm{R}}^2+{v_\mathrm{OP}}^2} $$ So √(0.87²+1.04²) = 1.36 μV_RMS = −117 dBV, as estimated.

The current noise is probably irrelevant for an FET-input op-amp, so we can skip to calculating the output noise: Just multiply the input noise by the gain of the amplifier. However, you need to multiply by the "noise gain", not the signal gain. To find the noise gain of the amp, convert your existing sources into short circuits and put a test voltage source right in series with the non-inverting input of the amp:

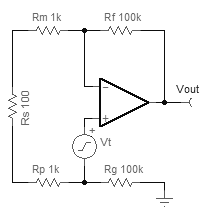
So the op-amp will do whatever it takes for the inverting input to equal the non-inverting input. There will be one current path: $$ I=\frac{V_\mathrm{out}}{R_\mathrm{f}+R_\mathrm{m}+R_\mathrm{s}+R_\mathrm{p}+R_\mathrm{g}} $$ and this is related to \$V_\mathrm{t}\$ by: $$ V_\mathrm{t}=I(R_\mathrm{m}+R_\mathrm{s}+R_\mathrm{p}) $$ combining and solving: $$ \frac {V_\mathrm{out}}{V_\mathrm{t}} = \frac {R_\mathrm{f}+R_\mathrm{m}+R_\mathrm{s}+R_\mathrm{p}+R_\mathrm{g}}{R_\mathrm{m}+R_\mathrm{s}+R_\mathrm{p}} $$ So in our case, this is a noise gain of 96.2× = +39.7 dB, and our input noise of −117 dBV becomes −77 dBV at the output. (A TINA simulation gives 137.5 μV_RMS = −77 dBV, for comparison.)

More detailed steps

There are several extra steps you can do to make your calculation more accurate:

To calculate the effect of the op-amp's current noise, take the current noise and multiply it by the equivalent resistance calculated earlier. For the TLC071, this is 0.6 fA/√Hz. So, combined with \$R_\mathrm{eq}\$ of 2.1 kΩ, we get 0.00126 nV/√Hz. Obviously this is much smaller than the op-amp's voltage noise, so it will have no effect on the result in this example. In cases with large \$R_\mathrm{eq}\$, it will have an effect. You can calculate it this way and combine it with the other sources as shown above: $$ v_\mathrm{total}=\sqrt{{v_\mathrm{R}}^2+{v_\mathrm{V}}^2+{v_\mathrm{I}}^2} $$ Also likely to have an effect is the bandwidth of your measurement equipment. The previous measurements assume a brickwall filter at 22 kHz, but brickwall filters can't exist in reality. You can correct for the fall-off of a real-life filter by calculating the equivalent noise bandwidth (ENBW). Here's a table of ENBW Filter correction factors vs order. See also Why are there two sets of ENBW correction factors?

In fact, voltage noise of the op-amp is not actually a constant. It varies with frequency, so is better written as \$\tilde v(f)\$. You can calculate it more accurately with numerical integration. See Noise and what does V/√Hz actually mean?