How do I expand the divider?

Question

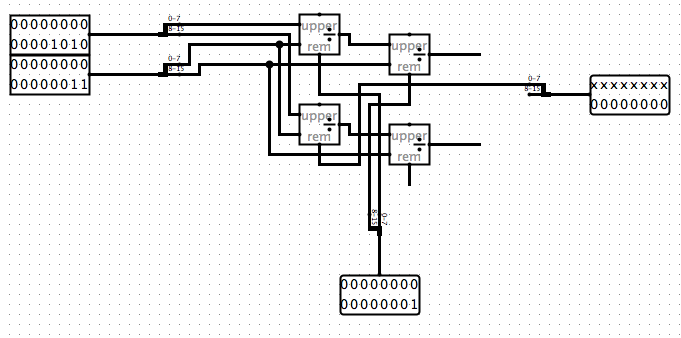
I'm trying to make a 128-bit divider in Logisim using Logisim's built-in arithmetic library. How do I connect the dividers so that I can work with divisions of more than 32 bits?

What I have considered:

1. Create the divider from logic gates (takes too long and would crash the simulation).
2. Write my own divider library (I don't know Java or the Logisim source code that well and I'm still stuck with the original problem).
3. Work with the dividers like I did the multipliers, but chain them in the reverse order (is that even plausible?).
4. Write an application to generate the divider (the resulting circuit would crash the simulation).

I might be able to provide a screenshot of what I described for #3 with my multiplier if I need to clarify that point.

Edit: Here is what I've come up with so far based on the answer I got:

Answer 1

When you want to build a 64 bit multiplier from 32 bit ones you can use the equation (a+b)*(c+d) = ac+bc+ad+bd You have four multiplications of 32 bit numbers for one 64 multiplication.

When you want to build a 64 bit divider from 32 bit ones the equation is (a+b)/(c+d) = a/(c+d) + b/(c+d) but this does not help much, you still have to divide 32 bits by 64 bits. You may try (a+b)/(c*d) = a/c/d + b/c/d but this is useful only if the divider may be split into two factors each smaller than 32 bits.

The properties of the division operation don't allow an expansion like that for multiplication.