I have heard my teacher saying** that low pass filter is more commonly used than high pass filter because in high pass filter, frequency increases with gain and thus noise also increases and thus it distorts the output. In case of low pass filter, frequency decreases with gain and thus noise decreases and thus output is less distorted.
I was skeptical about this explanation as I thought that low pass filter is used as much as high pass filter according to needs and there is no such thing that 'this' filter is more often than used than 'that'. I thought of doing a bit of research and I found that there are two types of noise- whiter noise and pink noise. White noise increases linearly with frequency and pink noise is inversely proportional to frequency. Generally, white noise is more dominant than pink noise and it is said that pink noise is still not exhibited for frequency as low as \$10^{-6}\$ Hz. So, I am assuming that white noise is exhibited and for higher frequency, white noise is dominant and thus distort the output. I found this forum of help and it says how to eliminate noise in low pass filter. So, my question is - Is low pass filter more often used than high pass filter? Is there any reason for this or is a false claim?
**My teacher said this statement during we were doing circuit analysis using simulink. He said to always use integrator instead of differentiator because in the laplace transform of differentiation is \$sX(s)-x(0)\$ whereas the laplace transform of integration is \$\frac{X(s)}s - x(0)\$ where \$s=j\omega\$. So, in differentiator, frequency increase and so is noise(act as high pass filter) and in integrator, frequency decreases and so is noise(act as low pass filter). So, eventually he made the statement of using low pass filter than high pass filter.

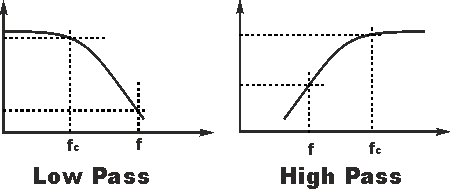
gain increases with frequency.. notfrequency increases with gain.... even though it seems to be the same when you say it. ... the gain is dependent on frequency, not the other way around. ... same thing with the low-pass ...gain decreases with frequency\$\endgroup\$