0
\$\begingroup\$

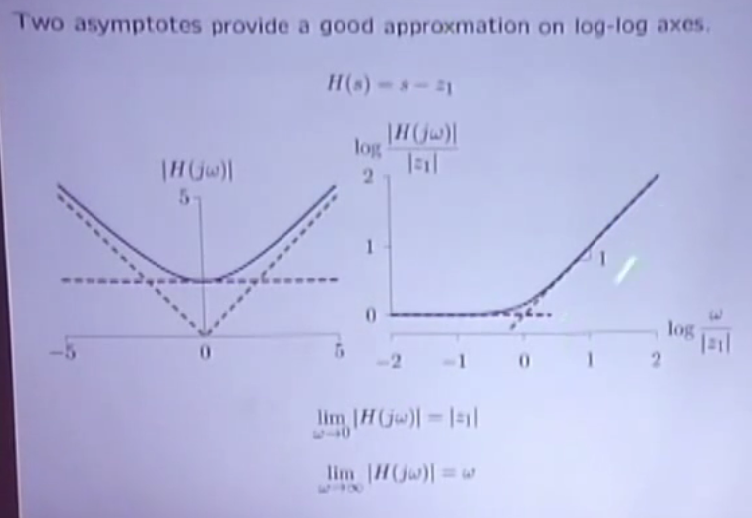
In below picture there are two graphs representing the function \$H(s) = s-z_1\$ (assume \$z_1=2\$ is a constant).

The left graph has \$\omega\$ on x axis and \$\|H(j\omega)\|\$ on y axis.
The right graph has \$\log\dfrac{\omega}{\|z_1\|}\$ on x axis and \$\log\dfrac{\| H(j\omega)\| }{\|z_1\|}\$ on y axis.

I completely understand the left graph. I don't understand how they got the right graph. I get why \$\|H(j\omega)\|\$ approaches \$\|z_1\|\$ as \$\omega \to 0\$, and I get why \$\|H(j\omega)\|\$ approaches \$\omega\$ as \$\omega \to \infty\$. But what happened to the graph for \$\omega \lt 0\$ ? I don't see it in the right graph ? enter image description here

EDIT : Kindly bear with the poor quality screenshot. Looks MIT folks have compressed too much these videos... Here is the video from which I took this screenshot

\$\endgroup\$
6
  • \$\begingroup\$ Consider using the snipping tool if you're using Windows. Your picture is a little fuzzy. \$\endgroup\$
    – user103380
    Commented Jun 26, 2018 at 16:11
  • \$\begingroup\$ "a little" is "barely readable". \$\endgroup\$
    – Marcus Müller
    Commented Jun 26, 2018 at 16:29
  • \$\begingroup\$ @MarcusMüller, are you on a phone? On a desktop it's a bit fuzzy but clear enough to convey what's needed to answer the question. \$\endgroup\$
    – The Photon
    Commented Jun 26, 2018 at 16:30
  • 1
    \$\begingroup\$ Desktop with less than optimally backlit screen; the fact that OP actually used a flash kind of kicked this from "was a little careless when supplying info" to "oh, come on, you can do better than that with this much reputation" \$\endgroup\$
    – Marcus Müller
    Commented Jun 26, 2018 at 16:31
  • \$\begingroup\$ Sorry friends I don't mean to be careless, actual quality of the youtube video itself is poor. I can point the video if you want ? \$\endgroup\$
    – AgentS
    Commented Jun 26, 2018 at 16:33

1 Answer 1

Reset to default
3
\$\begingroup\$

But what happened to the graph for ω < 0 ?

A log scaled axis can't show the range \$\omega < 0\$, because the logarithm function isn't defined (or if you do define it, isn't real valued) for arguments less than or equal to zero.

If this is a real filter (real-valued inputs and outputs) then you know that the response to negative frequencies is the complex conjugate of the response to positive frequencies, so graphing the negative frequencies doesn't give you any new information and isn't necessary.

\$\endgroup\$
3
  • \$\begingroup\$ Ohk... right side graph is only for \$\omega \gt 0\$, then I guess it makes sense... when \$\omega = |z_1|10^{-2}\$ etc the x values get negative, but the y value stays around \$0\$ because \$\log \dfrac{\|H(j\omega)\|}{\|z_1\|} \approx \log \dfrac{\|z_1\|}{\|z_1\|} = 0\$. I think I get this. Thank you so much :) \$\endgroup\$
    – AgentS
    Commented Jun 26, 2018 at 16:43
  • \$\begingroup\$ I have a small question, why are they using \$\log \dfrac{\omega}{\|z_1\|}\$ on x axis ? Because, for the magnitude we have $$\|H(j\omega)\| = \sqrt{\omega^2+4}=2\sqrt{(\omega/2)^2+1}$$ Taking log both sides and rearranging gives $$\color{blue}{\log \dfrac{\|H(j\omega)\|}{2}} = \dfrac{1}{2}\color{red}{\log((\omega/2)^2+1)}$$ \$\endgroup\$
    – AgentS
    Commented Jun 27, 2018 at 1:08
  • \$\begingroup\$ This is of form $$\color{blue}{y} = \dfrac{1}{2}\color{red}{x}$$ shouldn't we be letting \$\color{red}{x=\log((\omega/2)^2+1)}\$ instead ? \$\endgroup\$
    – AgentS
    Commented Jun 27, 2018 at 1:10

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.