Cascade multiple Full-bridge Rectifiers Connected to Multiple AC Sources

Question

I want to rectify multiple isolated AC signals (Generated from multiple wireless power receiving coils) to a single DC load. For example, consider the following the schematic with two voltage sources \$V_1\$ and \$V_2\$ representing two wireless power receiving coils. \$V_1\$ and \$V_2\$ are at the same frequency (say around \$500~\rm{kHz}\$) and same phase.

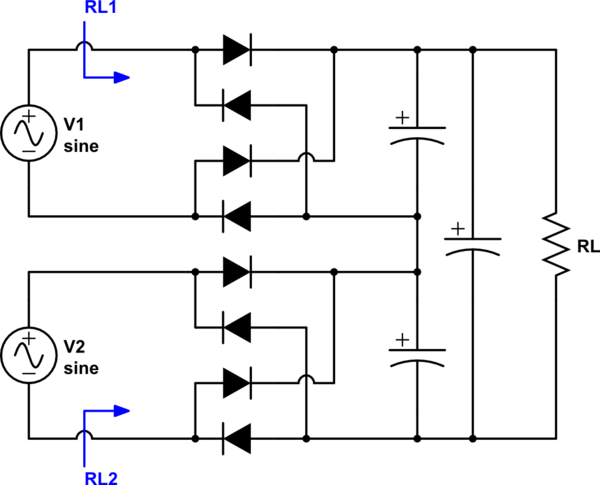
^{simulate this circuit – Schematic created using CircuitLab}

Question One:(solved more or less from the answer by @AndyAka and the other comments)

1. Will this circuit works even if the magnitudes of \$V_1\$ and \$V_2\$ vary significantly? For instance, the magnitude of both sources can vary between \$5\$ V to \$20\$V independently. Is there any better topology?

Question Two

2. How do we calculate effective AC load impedances seen by two sources (i.e. \$R_{L1}\$ and \$R_{L2}\$)?

For example, if we have a single rectifier, we know its equivalent ac-side impedance is \$\frac{\pi^2 R_L}{8}\$[reference]. Now the question is, how can we calculate the equivalent impedance seen by two AC sides (see schematic \$R_{L1}\$ and \$R_{L2}\$)?

Here is my approach so far: Both ac links will have the same current. Therefore, equivalent load impedances should be proportional to the voltages, i.e. \$\frac{R_{L1}}{R_{L2}}=\frac{V_1}{V_2}\$. But what will be their values? is \$R_{L1}+R_{L2}=\dfrac{\pi^2 R_L}{8}\$? If this way of calculation is correct, I have another problem because of my \$V_1\$ and \$V_2\$ also dependent on the equivalent load impedances \$R_{L1}\$ and \$R_{L2}\$. In this case, do I have to use an iterative method to solve this problem?

Answer 1

Observations:

• With the bridge rectifiers in series, if (say) V2 was quite active and (say) V1 was close to zero volts, the DC output would be 4 diode drops down on the AC voltage and this is worse than just collecting energy from V2 and its bridge rectifier.
• Having the bridge outputs in parallel means you always collect "decent" energy even if only 1 source is active but, the down side is that you only collect energy from 1 source (that source being the one with the largest amplitude).
• If the receive coils are individually resonant tuned (using parallel capacitors) then you might consider having all receive windings in series and one parallel tuning capacitor.

I would urge you to do simulations as this will tell you a lot and, the number of scenarios that can be tested will prove to be a better source of what you need to know than my limited set of observations. If this was my project, I'd be simulating it to death because IT WILL provide decent and real-world repeatable results.