Gaussian Channel: Capacity and Explanation

Question

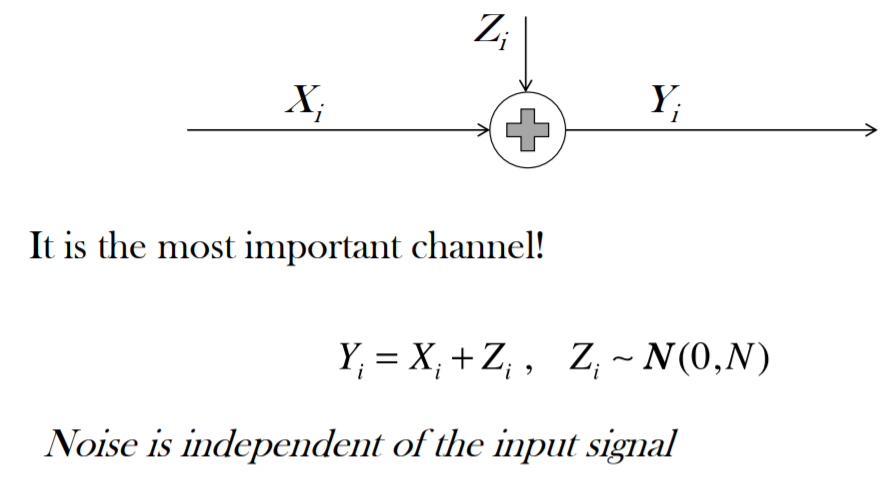
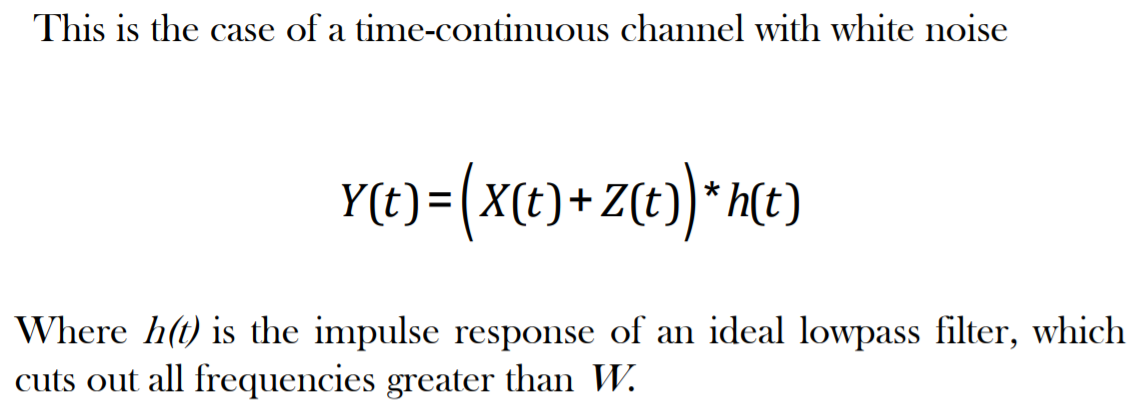Let's consider a gaussian communication channel, which is represented through this scheme:

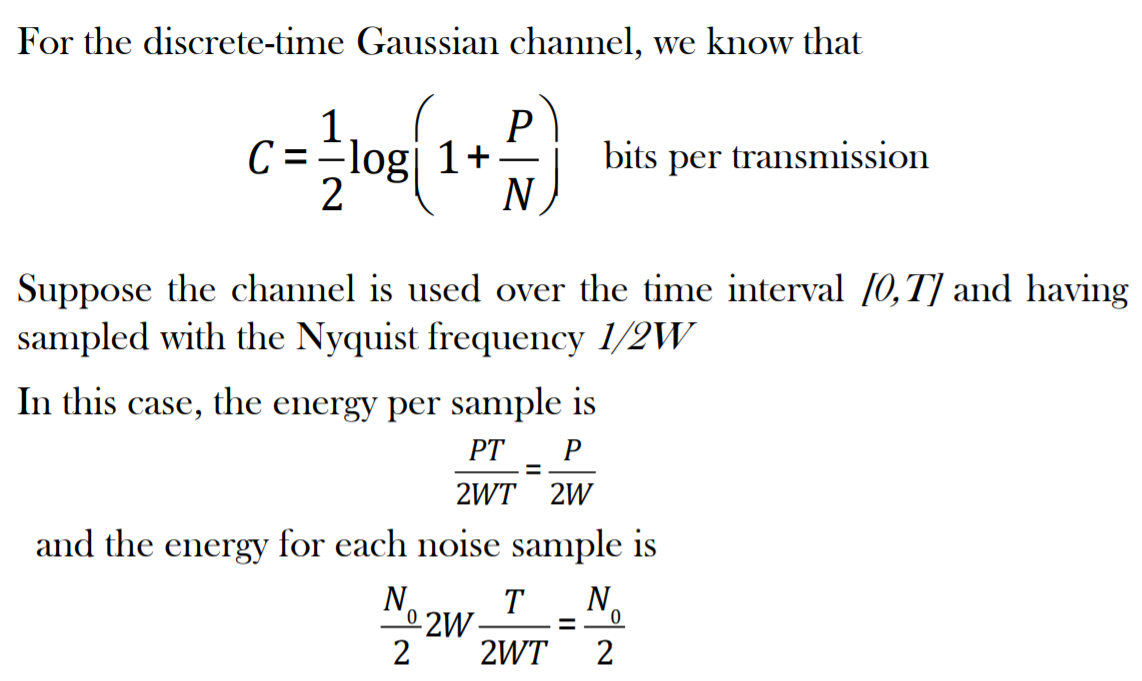
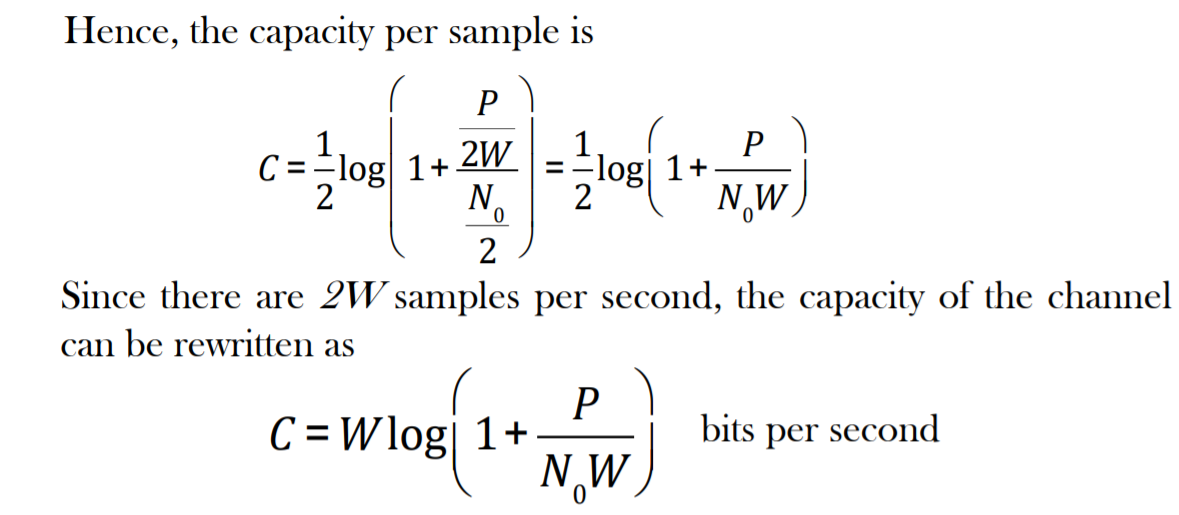
Starting by this assumption it is possible to prove that the channel capacity is equal to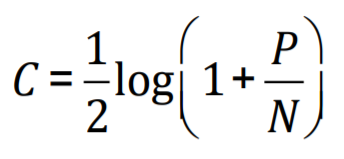

The computation of the last one is written in these slides. Now, in these slides this situation is considered:

Now I have the following questions:

1) Why is there the filter h(t)? What does it represent? Is it a physical filter that we put in the channel (and if yes, why?) or is it used to model the fact that a channel is naturally a low pass filter?

2) Why is the signal X sampled?

3) Should X be band limited or not? And why?

Answer 1

1) Why is there the filter h(t)? What does it represent? Is it a physical filter that we put in the channel (and if yes, why?) or is it used to model the fact that a channel is naturally a low pass filter?

Real channels filter the signal passed through them.

Transmission lines are lossy at high frequencies. Optical fibers have dispersion that smears out high frequency signals. To use a free space channel you have to pass your signal through a transmitter and a receiver that have bandwidth restrictions.

The channel response \$h(t)\$ represents whatever filtering effect the channel has.

2) Why is the signal X sampled?

To obtain quantifiable information from it.

3) Should X be band limited or not? And why?

If it wasn't originally, it is after passing it through the ideal band-pass filter \$h(t)\$.